矩阵乘法 POJ3070 Fibonacci
| Time Limit: 1000MS | Memory Limit: 65536K | |
| Total Submissions: 15215 | Accepted: 10687 |
Description
In the Fibonacci integer sequence, F0 = 0, F1 = 1, and Fn = Fn − 1 + Fn − 2 for n ≥ 2. For example, the first ten terms of the Fibonacci sequence are:
0, 1, 1, 2, 3, 5, 8, 13, 21, 34, …
An alternative formula for the Fibonacci sequence is
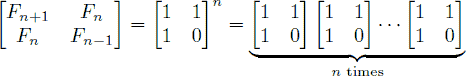 .
.
Given an integer n, your goal is to compute the last 4 digits of Fn.
Input
The input test file will contain multiple test cases. Each test case consists of a single line containing n (where 0 ≤ n ≤ 1,000,000,000). The end-of-file is denoted by a single line containing the number −1.
Output
For each test case, print the last four digits of Fn. If the last four digits of Fn are all zeros, print ‘0’; otherwise, omit any leading zeros (i.e., print Fn mod 10000).
Sample Input
0 9 999999999 1000000000 -1
Sample Output
0 34 626 6875
Hint
As a reminder, matrix multiplication is associative, and the product of two 2 × 2 matrices is given by
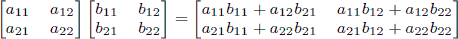 .
.
Also, note that raising any 2 × 2 matrix to the 0th power gives the identity matrix:
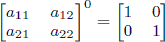 .
.
Source
1 #include<iostream> 2 #include<cstdio> 3 #include<cstring> 4 #include<algorithm> 5 using namespace std; 6 int n,a[2][2],b[2][2]; 7 void mul(int a[2][2],int b[2][2],int ans[2][2]){ 8 int t[2][2]; 9 for(int i=0;i<2;i++) 10 for(int j=0;j<2;j++){ 11 t[i][j]=0; 12 for(int k=0;k<2;k++) t[i][j]=(t[i][j]+a[i][k]*b[k][j])%10000; 13 } 14 for(int i=0;i<2;i++) 15 for(int j=0;j<2;j++) ans[i][j]=t[i][j]; 16 } 17 int main(){ 18 while(scanf("%d",&n)){ 19 if(n==-1) return 0; 20 a[0][0]=a[1][0]=a[0][1]=b[0][0]=b[1][1]=1; 21 a[1][1]=b[1][0]=b[0][1]=0; 22 while(n){ 23 if(n&1) mul(a,b,b); 24 n>>=1; 25 mul(a,a,a); 26 } 27 printf("%d\n",b[1][0]); 28 } 29 return 0; 30 }





【推荐】编程新体验,更懂你的AI,立即体验豆包MarsCode编程助手
【推荐】凌霞软件回馈社区,博客园 & 1Panel & Halo 联合会员上线
【推荐】抖音旗下AI助手豆包,你的智能百科全书,全免费不限次数
【推荐】轻量又高性能的 SSH 工具 IShell:AI 加持,快人一步
· 深入理解 Mybatis 分库分表执行原理
· 如何打造一个高并发系统?
· .NET Core GC压缩(compact_phase)底层原理浅谈
· 现代计算机视觉入门之:什么是图片特征编码
· .NET 9 new features-C#13新的锁类型和语义
· Spring AI + Ollama 实现 deepseek-r1 的API服务和调用
· 《HelloGitHub》第 106 期
· 数据库服务器 SQL Server 版本升级公告
· 深入理解Mybatis分库分表执行原理
· 使用 Dify + LLM 构建精确任务处理应用