PAT 甲级 1086 Tree Traversals Again

https://pintia.cn/problem-sets/994805342720868352/problems/994805380754817024

 

An inorder binary tree traversal can be implemented in a non-recursive way with a stack. For example, suppose that when a 6-node binary tree (with the keys numbered from 1 to 6) is traversed, the stack operations are: push(1); push(2); push(3); pop(); pop(); push(4); pop(); pop(); push(5); push(6); pop(); pop(). Then a unique binary tree (shown in Figure 1) can be generated from this sequence of operations. Your task is to give the postorder traversal sequence of this tree.

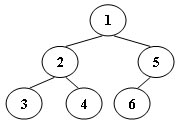
Figure 1

Input Specification:

Each input file contains one test case. For each case, the first line contains a positive integer N (≤) which is the total number of nodes in a tree (and hence the nodes are numbered from 1 to N). Then 2 lines follow, each describes a stack operation in the format: "Push X" where X is the index of the node being pushed onto the stack; or "Pop" meaning to pop one node from the stack.

Output Specification:

For each test case, print the postorder traversal sequence of the corresponding tree in one line. A solution is guaranteed to exist. All the numbers must be separated by exactly one space, and there must be no extra space at the end of the line.

Sample Input:

6
Push 1
Push 2
Push 3
Pop
Pop
Push 4
Pop
Pop
Push 5
Push 6
Pop
Pop

Sample Output:

3 4 2 6 5 1

代码：

#include <bits/stdc++.h>
using namespace std;

int N;
vector<int> in, post, pre, val;

void postorder(int root, int st, int en) {
    if(st > en) return;
    int i = st;
    while(i < en && in[i] != pre[root]) i ++;
    postorder(root + 1, st, i - 1);
    postorder(root + 1 + i - st, i + 1, en);
    post.push_back(pre[root]);
}

int main() {
    scanf("%d", &N);
    stack<int> s;
    string op;
    int cnt = 0;
    for(int t = 0; t < N * 2; t ++) {
        cin >> op;
        if(op == "Push") {
            int x;
            scanf("%d", &x);
            pre.push_back(cnt);
            val.push_back(x);
            s.push(cnt ++);
        } else {
            in.push_back(s.top());
            s.pop();
        }
    }

    postorder(0, 0, N - 1);
    for(int i = 0; i < N; i ++) {
        printf("%d", val[post[i]]);
        printf("%s", i != N - 1 ? " " : "");
    }

    return 0;
}

　　push 的顺序是前序遍历的顺序 按照题目 pop 得到的中序遍历的顺便 in 和 pre 存的是数字的位置 val 求数字的值 递归求出后序遍历