#Leetcode# 63. Unique Paths II

https://leetcode.com/problems/unique-paths-ii/

 

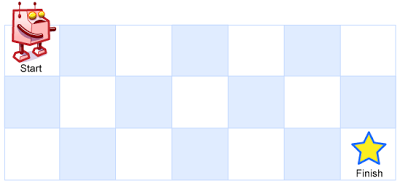
A robot is located at the top-left corner of a m x n grid (marked 'Start' in the diagram below).

The robot can only move either down or right at any point in time. The robot is trying to reach the bottom-right corner of the grid (marked 'Finish' in the diagram below).

Now consider if some obstacles are added to the grids. How many unique paths would there be?

An obstacle and empty space is marked as 1 and 0 respectively in the grid.

Note: m and n will be at most 100.

Example 1:

Input:
[
  [0,0,0],
  [0,1,0],
  [0,0,0]
]
Output: 2
Explanation:
There is one obstacle in the middle of the 3x3 grid above.
There are two ways to reach the bottom-right corner:
1. Right -> Right -> Down -> Down
2. Down -> Down -> Right -> Right

代码：

class Solution {
public:
    int uniquePathsWithObstacles(vector<vector<int>>& obstacleGrid) {
        int m = obstacleGrid.size(), n = obstacleGrid[0].size();
        if(obstacleGrid[0][0] == 1) return 0;
        vector<int> dp(n, 0);
        dp[0] = 1;
        for(int i = 0; i < m; i ++) {
            for(int j = 0; j < n; j ++) {
                if(obstacleGrid[i][j] == 1) dp[j] = 0;
                else dp[j] += dp[j - 1];
            }
        }
        return dp[n - 1];
    }
};

　　

$m$ 行 $n$ 列真的是写不习惯 这个只多了一个是 $1$ 的时候走不动 那就到该点的时候 $dp$ 设成 $0$ 就好了 本来想用深搜搜路径数量但是。。。很难受写错了。。。晚上回去再好好撸 $dfs$ 吧