Loj 10115 「一本通 4.1 例 3」校门外的树 (树状数组)
题目链接:https://loj.ac/problem/10115
题目描述
原题来自:Vijos P1448
校门外有很多树,学校决定在某个时刻在某一段种上一种树,保证任一时刻不会出现两段相同种类的树,现有两种操作:
- K=1,读入l,r 表示在l 到 r 之间种上一种树,每次操作种的树的种类都不同;
- K=2,读入 l,r 表示询问 l 到 r 之间有多少种树。
注意:每个位置都可以重复种树。
输入格式
第一行 表示道路总长为 n,共有 m个操作;
接下来 m 行为 m 个操作。
输出格式
对于每个 K=2 输出一个答案。
样例
样例输入
5 4
1 1 3
2 2 5
1 2 4
2 3 5
样例输出
1
2
解题思路:开始怎么想都不知道怎么维护不同段中树的种类是否相同的情况,感觉这题有个思维技巧还是挺难想的,就是我们要开两个数组,sum1分别维护左端点的数目,另一个数组sum2维护右端点的数目。这样区间[l,r]的树的种类的数目就是1-r中左端点的数目减去1-(l-1)中右端点的数目,即表示为sum1[r]-sum2[l-1]。
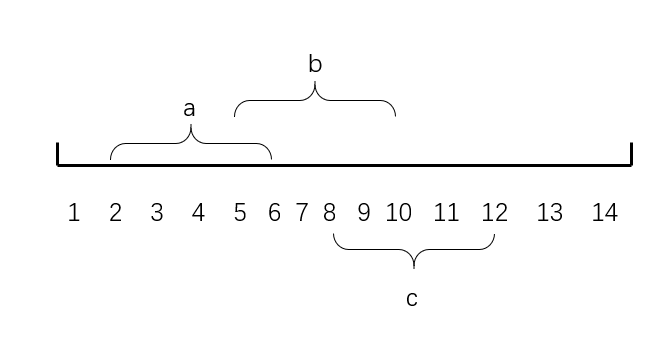
如图假如我们第一次在区间a[2,6]种上一种树,然后再在区间b[5,10]种上一种树,这时我们要统计区间c[8,12]中树的种类数目,我们就统计[1,12]中左端点的数目即 sum1[12]等于2,说明有两种树可能在给定区间内,然后我们再求区间[1,7]中右端点的数目即sum2[7-1]=1,表示有一种树完全在给定区间左边,并不是我们要求的,所以减去就好了,所以答案就为sum1[12]-sum2[7-1]了。
代码:
#include<iostream> #include<cstdio> #include<cstring> #include<cmath> #include<map> #include<algorithm> #include<queue> #define mod 1000000007 using namespace std; typedef long long ll; const int maxn=5e4+10; int n,m,k,l,r,sum1[maxn],sum2[maxn]; //sum1[i]表示的是区间[1,i]中左端点的数目,sum2[i]表示的是区间[1,i]右端点的数目; int lowbit(int x){return x&(-x);} void update1(int x,int val){ //更新左端点的数目 while(x<=maxn){ sum1[x]+=val; x+=lowbit(x); } } void update2(int x,int val){ //更新右端点的数目 while(x<=maxn){ sum2[x]+=val; x+=lowbit(x); } } int ask1(int x){ //查找区间[1,x]中左端点的数目 int res=0; while(x){ res+=sum1[x]; x-=lowbit(x); } return res; } int ask2(int x){ //查找区间[1,x]中右端点的数目 int res=0; while(x){ res+=sum2[x]; x-=lowbit(x); } return res; } int main(){ cin>>n>>m; while(m--){ cin>>k>>l>>r; if(k==1){ update1(l,1); update2(r,1); }else{ cout<<ask1(r)-ask2(l-1)<<endl; } } return 0; }



