POJ1390Blocks(DP+好题+抽空再来理解理解）

Blocks

Time Limit: 5000MS		Memory Limit: 65536K
Total Submissions: 4744		Accepted: 1930

Description

Some of you may have played a game called 'Blocks'. There are n blocks in a row, each box has a color. Here is an example: Gold, Silver, Silver, Silver, Silver, Bronze, Bronze, Bronze, Gold.
The corresponding picture will be as shown below:

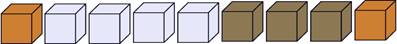
Figure 1
If some adjacent boxes are all of the same color, and both the box to its left(if it exists) and its right(if it exists) are of some other color, we call it a 'box segment'. There are 4 box segments. That is: gold, silver, bronze, gold. There are 1, 4, 3, 1 box(es) in the segments respectively.

Every time, you can click a box, then the whole segment containing that box DISAPPEARS. If that segment is composed of k boxes, you will get k*k points. for example, if you click on a silver box, the silver segment disappears, you got 4*4=16 points.

Now let's look at the picture below:

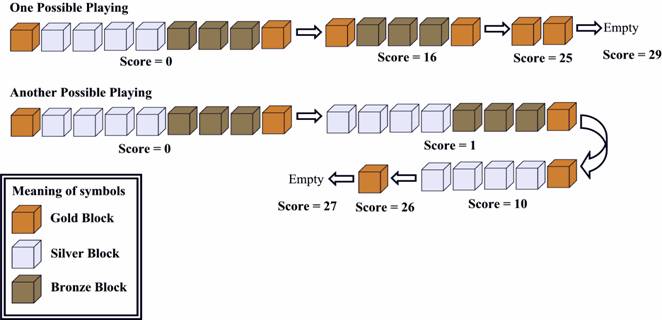
Figure 2

The first one is OPTIMAL.

Find the highest score you can get, given an initial state of this game.

Input

The first line contains the number of tests t(1<=t<=15). Each case contains two lines. The first line contains an integer n(1<=n<=200), the number of boxes. The second line contains n integers, representing the colors of each box. The integers are in the range 1~n.

Output

For each test case, print the case number and the highest possible score.

Sample Input

2
9
1 2 2 2 2 3 3 3 1
1
1

Sample Output

Case 1: 29
Case 2: 1
题意：通过点击某一颜色消除相邻的所有的这种颜色，得分为len*len,求最大分；
http://wenku.baidu.com/view/d956d2f30b4c2e3f5627630b
分析：当看代码的时候却觉着原来就是这么简单

#include <iostream>
#include <cstring>
#include <cstdio>
#include <algorithm>

using namespace std;
struct box_segment
{
    int color,len;
};
box_segment segment[200];
int score[200][200][200];
int click_score(int start,int End,int extra_len)
{
    if(score[start][End][extra_len] > 0)
        return score[start][End][extra_len];
    int result;
    result = segment[End].len + extra_len;
    result = result * result;
    if(start == End)
    {
        score[start][End][extra_len] = result;
        return score[start][End][extra_len];
    }
    result += click_score(start, End - 1, 0);
    for(int i = End - 1; i >= start; i--)
    {
        if(segment[i].color != segment[End].color)
            continue;
        int temp = click_score(start, i, segment[End].len + extra_len) + click_score(i + 1, End - 1,0);
        if(temp <= result)
            continue;
        result = temp;
        break;
    }
    score[start][End][extra_len] = result;
    return score[start][End][extra_len];
}
int main()
{
    int t,n,End;
    int num = 0;
    scanf("%d", &t);
    while(t--)
    {
        End = 0;
        scanf("%d", &n);
        scanf("%d", &segment[End].color);
        segment[End].len = 1;
        for(int i = 1; i < n; i ++)
        {
            int color;
            scanf("%d", &color);
            if(color == segment[End].color)
                segment[End].len++;
            else
            {
                segment[++End].color = color;
                segment[End].len = 1;
            }
        }
        memset(score, 0, sizeof(score));
        printf("Case %d: %d\n", ++num,click_score(0, End, 0));
    }
    return 0;
}