poj-3150-Cellular Automation

poj-3150-Cellular Automation

 

Cellular Automaton

Time Limit: 12000MS		Memory Limit: 65536K
Total Submissions: 3795		Accepted: 1557
Case Time Limit: 2000MS

Description

A cellular automaton is a collection of cells on a grid of specified shape that evolves through a number of discrete time steps according to a set of rules that describe the new state of a cell based on the states of neighboring cells. The order of the cellular automaton is the number of cells it contains. Cells of the automaton of order n are numbered from 1 to n.

The order of the cell is the number of different values it may contain. Usually, values of a cell of order m are considered to be integer numbers from 0 to m − 1.

One of the most fundamental properties of a cellular automaton is the type of grid on which it is computed. In this problem we examine the special kind of cellular automaton — circular cellular automaton of order n with cells of order m. We will denote such kind of cellular automaton as n,m-automaton.

A distance between cells i and j in n,m-automaton is defined as min(|i − j|, n − |i − j|). A d-environment of a cell is the set of cells at a distance not greater than d.

On each d-step values of all cells are simultaneously replaced by new values. The new value of cell i after d-step is computed as a sum of values of cells belonging to the d-enviroment of the cell i modulo m.

The following picture shows 1-step of the 5,3-automaton.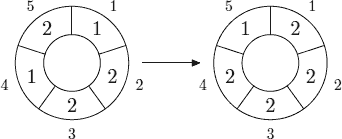

The problem is to calculate the state of the n,m-automaton after k d-steps.

Input

The first line of the input file contains four integer numbers n, m, d, and k (1 ≤ n ≤ 500, 1 ≤ m ≤ 1 000 000, 0 ≤ d < ⁿ⁄₂ , 1 ≤ k ≤ 10 000 000). The second line contains n integer numbers from 0 to m − 1 — initial values of the automaton’s cells.

Output

Output the values of the n,m-automaton’s cells after k d-steps.

Sample Input

sample input #1
5 3 1 1
1 2 2 1 2

sample input #2
5 3 1 10
1 2 2 1 2

Sample Output

sample output #1
2 2 2 2 1

sample output #2
2 0 0 2 2

Source

Northeastern Europe 2006

 

 

17861637

3150

Accepted

408K

3579MS

G++

1036B

2017-11-19 20:48:11

 

元宝自动机。

经典的矩阵快速计算方法。

将有规律的矩阵简化为向量，由原来的 n^3 复杂度减小到 n^2 。

 

首先来看一下Sample里的第一组数据。
1 2 2 1 2
经过一次变换之后就成了
5 5 5 5 4
它的原理就是
a0 a1 a2 a3 a4
->(a4+a0+a1) (a0+a1+a2) (a1+a2+a3) (a2+a3+a4) (a3+a4+a0)
如果用矩阵相乘来描述，那就可以表述为1xN和NxN的矩阵相乘，结果仍为1xN矩阵
b = 1 2 2 1 2
a =
1 1 0 0 1
1 1 1 0 0
0 1 1 1 0
0 0 1 1 1
1 0 0 1 1
b * a = 5 5 5 5 4
所以最终结果就是：a * (b^k)

复杂度是n^3logk，过不了

于是我们发现这个矩阵长得很奇葩，每一行都是上一行后移一位得到

所以我们每个矩阵可以变成一行

 

参考： https://www.cnblogs.com/iwtwiioi/p/3946211.html

 

// poj-3150 

#include <cstdio> 
#include <cstring> 
const int MAXN = 500 + 10; 

long long n, m, d, k, num[MAXN], a[MAXN], b[MAXN], tmp[MAXN], ans[MAXN]; 

void MultiPle(long long *aa, long long *bb, long long *cc, int len){ 
	for(int i=0; i<len; ++i){
		tmp[i] = 0; 
		for(int j=0; j<len; ++j){
			if(i - j >= 0){
				tmp[i] += (aa[j] * bb[i - j]) % m;  
			}else{
				tmp[i] += (aa[j] * bb[i - j + n]) % m; 
			}
			tmp[i] %= m; 
		}
	}
	for(int i=0; i<len; ++i){
		cc[i] = tmp[i]; 
	}
}

int main(){
	freopen("in.txt", "r", stdin); 

	while(scanf("%lld %lld %lld %lld", &n, &m, &d, &k) != EOF){
		for(int i=0; i<n; ++i){
			scanf("%lld", &num[i]); 
		}
		memset(a, 0, sizeof(a)); 
		memset(b, 0, sizeof(b)); 

		for(int i=0; i<=d; ++i){
			a[i] = 1; 
		}
		for(int i=n-1; i>n-1-d; --i){
			a[i] = 1; 
		}
		b[0] = 1; 

		while(k){
			if(k % 2 == 1){
				MultiPle(b, a, b, n); 
			}
			k /= 2; 
			MultiPle(a, a, a, n); 
		}
		MultiPle(num, b, ans, n ); 

		for(int i=0; i<n-1; ++i){
			printf("%lld ",  ans[i]);
		}
		printf("%lld\n", ans[n-1] );
	}
	return 0; 
}