codeforces 486C Palindrome Transformation 贪心求构造回文

点击打开链接

C. Palindrome Transformation

time limit per test

1 second

memory limit per test

256 megabytes

input

standard input

output

standard output

Nam is playing with a string on his computer. The string consists of n lowercase English letters. It is meaningless, so Nam decided to make the string more beautiful, that is to make it be a palindrome by using 4 arrow keys: left, right, up, down.

There is a cursor pointing at some symbol of the string. Suppose that cursor is at position i (1 ≤ i ≤ n, the string uses 1-based indexing) now. Left and right arrow keys are used to move cursor around the string. The string is cyclic, that means that when Nam presses left arrow key, the cursor will move to position i - 1 if i > 1 or to the end of the string (i. e. position n) otherwise. The same holds when he presses the right arrow key (if i = n, the cursor appears at the beginning of the string).

When Nam presses up arrow key, the letter which the text cursor is pointing to will change to the next letter in English alphabet (assuming that alphabet is also cyclic, i. e. after 'z' follows 'a'). The same holds when he presses the down arrow key.

Initially, the text cursor is at position p.

Because Nam has a lot homework to do, he wants to complete this as fast as possible. Can you help him by calculating the minimum number of arrow keys presses to make the string to be a palindrome?

Input

The first line contains two space-separated integers n (1 ≤ n ≤ 105) and p (1 ≤ p ≤ n), the length of Nam's string and the initial position of the text cursor.

The next line contains n lowercase characters of Nam's string.

Output

Print the minimum number of presses needed to change string into a palindrome.

Sample test(s)

input

8 3
aeabcaez

output

6

Note

A string is a palindrome if it reads the same forward or reversed.

In the sample test, initial Nam's string is: 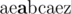 (cursor position is shown bold).

In optimal solution, Nam may do 6 following steps:

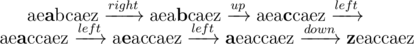
The result, 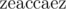, is now a palindrome.

给你一个字符串以及它的长度。而且给你一个指针，指向字符串的初始位置，字符能够+或者-，字符a能够减到z，字符z能够加到a，指针能够向左或者向右移动，0号位置能够移动到最后的位置，最后的位置也能够移动到0号位置。

问最少多少部操作才干使得此字符串成为回文串。

依据指针起始位置。能够推断移动的时候在左、右的哪半部分移动，初始化求出字符须要变化的次数，然后贪心求出指针移动的次数。

//31 ms	 900 KB
#include<stdio.h>
#include<algorithm>
#include<math.h>
using namespace std;
char s[100007];
int x[100007],y[100007];
int main()
{
    int len,p,count=0,num=0,j;
    scanf("%d%d%s",&len,&p,s+1);
    if(len==1)
    {
        printf("0\n");
        return 0;
    }
    if(len&1)j=len/2+2;
    else j=len/2+1;
    for(int i=len/2; i>=0&&j<=len; i--,j++)
        if(s[i]!=s[j])
        {
            int a=s[i]-'a';
            int b=s[j]-'a';
            int minn=min(a,b);
            int maxx=max(a,b);
            count+=min(maxx-minn,minn+26-maxx);
            x[num]=i;
            y[num++]=j;
        }
    sort(x,x+num);
    sort(y,y+num);
    if(p<=len/2)//假设指针在左部分
    {
        if(num!=0)
        if(num==1)count+=fabs(x[0]-p);
        else
        {
            int flag=0;
            int aa=min(fabs(x[num-1]-p),fabs(p-x[0]));
            if(x[0]<=p){flag++;count+=(p-x[0]);}//求最左面元素距离指针的距离
            if(x[num-1]>p){flag++;count+=(x[num-1]-p);}//求最右面元素距离指针的距离
            if(flag==2)count+=aa;//求最小距离
        }
    }
    else//假设指针在右部分
    {
        if(num!=0)
        if(num==1)count+=fabs(y[0]-p);
        else
        {
            int flag=0;
            int aa=min(fabs(y[num-1]-p),fabs(p-y[0]));
            if(y[0]<=p){flag++;count+=(p-y[0]);}
            if(y[num-1]>p){flag++;count+=(y[num-1]-p);}
            if(flag==2)count+=aa;
        }
    }
    printf("%d\n",count);
}