Java基础-时间复杂度计算方式

　　　　　　　　　　　　　Java基础-时间复杂度计算方式

　　　　　　　　　　　　　　　　　　　　　　　　　　　　　作者：尹正杰

版权声明：原创作品，谢绝转载！否则将追究法律责任。

 

 

　　时间复杂度通常是衡量算法的优劣的，衡量算法的时间严格来讲是很难衡量的，由于不同的机器性能不用环境都会造成不同的执行时间。

 

一.什么是时间复杂度

1>.什么是时间频度

　　算法的执行时间和语句的执行次数成正比，因此通过计算执行测试来推断执行时间。算法中语句执行次数称为语句频度或时间频度，记为T(n)，n是问题的规模，T是Time。

2>.什么是时间复杂度

　　当时间频度的n不断变化时，T(n)也在不断变化，为了考察两者变化时呈现什么规律，可以使用时间复杂度来计算。通常操作重复执行的次数是问题规模n的某个函数，用T(n)表示，若有某个辅助函数f(n),存在一个正常数c使得f(n) * c >= T(n)恒成立。记作T(n)=O(f(n)),称O(f(n)) 为算法的渐进时间复杂度，简称时间复杂度。换句话说，只要计算一下语句的执行次数和n之间的关就可以了，去除系数部分，低阶项部分，就是时间复杂度的值了。

　　举个例子，在公式：“xxx = 3N^3 + 2n^2 + 5n”中，其中“2n^2”和“5n”相对于“3N^3 ”来说，属于低阶项部分，我们将其去除，在看“3N^3"中，”3“为系数部分，我们将其也去除，因此只剩下“N^3 ”啦！这个时候我们就说“xxx”的时间复杂度为“O(n^3)”。

 

二.时间复杂度的计算

1>.给定任意长度数组，读取数组第一个元素的值

 　　时间复杂度为：无论数组长度n为多少，代码执行一次。因此，时间复杂度为O(1)。实现代码如下：

/*
@author :yinzhengjie
Blog:http://www.cnblogs.com/yinzhengjie/tag/Java%E5%9F%BA%E7%A1%80/
EMAIL:y1053419035@qq.com
*/
package cn.org.yinzhengjie.java.timeComplexity;

public class Demo {
    public static void main(String[] args) {
        String[] names = {"尹正杰","yinzhengjie","YinZhengJie"};
        String firstElement = getFirstElement(names);
        System.out.println(firstElement);
    }

    public static String getFirstElement(String[] names){
        //无论数组的长度n为多少，代码只执行一次。
        return names[0];
    }
}

/*
以上代码输出结果如下：
尹正杰
 */

2>.循环n次，输出“尹正杰（yinzhengjie）”

　　随着n的增大，打印函数逐渐增多。打印代码执行的次数是n，因此时间复杂度为O(n)。

/*
@author :yinzhengjie
Blog:http://www.cnblogs.com/yinzhengjie/tag/Java%E5%9F%BA%E7%A1%80/
EMAIL:y1053419035@qq.com
*/
package cn.org.yinzhengjie.java.timeComplexity;

public class Demo {
    public static void main(String[] args) {
        String[] names = {"尹正杰","yinzhengjie","YinZhengJie"};
        printArray(5);
    }

    public static void printArray(int n){
        for(int i = 0 ; i < n ; i ++){
            //执行一次。
            System.out.println("尹正杰(yinzhengjie)") ;
        }
    }
}

/*
以上代码输出结果如下：
尹正杰(yinzhengjie)
尹正杰(yinzhengjie)
尹正杰(yinzhengjie)
尹正杰(yinzhengjie)
尹正杰(yinzhengjie)
 */

3>.打印99乘法表

 　　打印99正方乘法表，打印语句输出9 * 9 = 81次。打印语句执行次数：n * n = n^2​，因此时间复杂度是​​O(n^2)。

/*
@author :yinzhengjie
Blog:http://www.cnblogs.com/yinzhengjie/tag/Java%E5%9F%BA%E7%A1%80/
EMAIL:y1053419035@qq.com
*/
package cn.org.yinzhengjie.java.timeComplexity;

public class Demo {
    public static void main(String[] args) {
        printArray(9);
    }

    public static void printArray(int n){
        for(int i = 1 ; i <= n ; i ++){
            for(int j = 1 ; j <= i ; j ++){
                //打印语句的执行次数n * n次。
                System.out.printf("%d x %d = %d\t",j,i,(j*i));
            }
            System.out.println();
        }
    }
}

/*
以上代码输出结果如下：
1 x 1 = 1    
1 x 2 = 2    2 x 2 = 4    
1 x 3 = 3    2 x 3 = 6    3 x 3 = 9    
1 x 4 = 4    2 x 4 = 8    3 x 4 = 12    4 x 4 = 16    
1 x 5 = 5    2 x 5 = 10    3 x 5 = 15    4 x 5 = 20    5 x 5 = 25    
1 x 6 = 6    2 x 6 = 12    3 x 6 = 18    4 x 6 = 24    5 x 6 = 30    6 x 6 = 36    
1 x 7 = 7    2 x 7 = 14    3 x 7 = 21    4 x 7 = 28    5 x 7 = 35    6 x 7 = 42    7 x 7 = 49    
1 x 8 = 8    2 x 8 = 16    3 x 8 = 24    4 x 8 = 32    5 x 8 = 40    6 x 8 = 48    7 x 8 = 56    8 x 8 = 64    
1 x 9 = 9    2 x 9 = 18    3 x 9 = 27    4 x 9 = 36    5 x 9 = 45    6 x 9 = 54    7 x 9 = 63    8 x 9 = 72    9 x 9 = 81    
 */

4>.冒泡排序

 　　冒泡排序是经典的排序算法是每次比较相邻的两个元素进行对调，进行多轮，知道数列有序。对于n个元素的冒泡排序来说，共需要比较比较次数的公式如下：

　　因此时间复杂度为：​​O(n^2)，这是冒泡排序最坏的情况下的时间复杂度。具体实现代码如下：

/*
@author :yinzhengjie
Blog:http://www.cnblogs.com/yinzhengjie/tag/Java%E5%9F%BA%E7%A1%80/
EMAIL:y1053419035@qq.com
*/
package cn.org.yinzhengjie.java.timeComplexity;

public class Demo {
    public static void main(String[] args) {
        char[] arr = {'i','f','c','b','d','g','e','a','h'};
        printArray(arr);
        bubbleSort(arr);
        printArray(arr);
    }
    //进行冒泡排序
    public static void bubbleSort(char[] arr){
        //外层比较n-1次
        for(int i = 0 ; i < arr.length - 1 ; i ++){
            //比较n-1-i次
            for(int j = 0 ; j < arr.length -1 - i ; j ++){
                char temp = arr[j] ;
                if(arr[j] > arr[j + 1]){
                    arr[j] = arr[j + 1] ;
                    arr[j + 1] = temp ;
                }
            }
        }
    }
    //遍历数组
    public static void printArray(char[] arr){
        for (int i = 0; i < arr.length; i++) {
            char c = arr[i];
            System.out.print(c+" ");
        }
        System.out.println();
    }

}

/*
以上代码输出结果如下：
i f c b d g e a h
a b c d e f g h i 
 */

　　冒泡排序可以进行优化，在最好的情况下，复杂度为O(n)，具体代码如下：

/*
@author :yinzhengjie
Blog:http://www.cnblogs.com/yinzhengjie/tag/Java%E5%9F%BA%E7%A1%80/
EMAIL:y1053419035@qq.com
*/
package cn.org.yinzhengjie.java.timeComplexity;

public class Demo {
    public static void main(String[] args) {
        char[] arr = {'i','f','c','b','d','g','e','a','h'};
        printArray(arr);
        bubbleSort(arr);
        printArray(arr);
    }
    //进行冒泡排序
    public static void bubbleSort(char[] arr){
        //外层比较n-1次
        for(int i = 0 ; i < arr.length - 1 ; i ++){
            boolean flag = true ;
            //比较n-1-i次
            for(int j = 0 ; j < arr.length -1 - i ; j ++){
                char temp = arr[j] ;
                if(arr[j] > arr[j + 1]){
                    //发生交换，就设置标志位为false，即数列无序。
                    flag = false ;
                    arr[j] = arr[j + 1] ;
                    arr[j + 1] = temp ;
                }
            }
            //判断如果是数列有序，则终止循环。
            if(flag){
                break ;
            }
        }
    }
    //遍历数组
    public static void printArray(char[] arr){
        for (int i = 0; i < arr.length; i++) {
            char c = arr[i];
            System.out.print(c+" ");
        }
        System.out.println();
    }

}

/*
以上代码输出结果如下：
i f c b d g e a h
a b c d e f g h i
 */

　　设置标志位flag=1，表示整个数列已经有序，就不需要再排序了，在里层循环中，如果发现没有进行过数据交换，就所说数列已经有序。在最好情况下，就是数列本身已经有序，只需要执行外层的n-1次循环即可，因此复杂度为O(n)。

5>.折半查找

　　 折半查找也叫二分查找，是高效的查找方式，前提条件是数列需要时有序数列。实现代码如下：

/*
@author :yinzhengjie
Blog:http://www.cnblogs.com/yinzhengjie/tag/Java%E5%9F%BA%E7%A1%80/
EMAIL:y1053419035@qq.com
*/
package cn.org.yinzhengjie.java.timeComplexity;

public class Demo {
    public static void main(String[] args) {
        char[] arr = {'a','b','c','d','e','f','g'};
        int tag = 'n';
        int res = halfSearch(arr, tag);
        System.out.println(res);
        tag = 'd';
        int res2 = halfSearch(arr, tag);
        System.out.println(res2);

    }
    //进行冒泡排序
    public static int halfSearch(char[] arr, int tag){
        int min = arr[0] ;
        int max = arr[0] ;
        int mid = arr[arr.length/2] ;
        for( min = 0 , max = arr.length - 1 ; min <= max  ; ){
             mid = (min + max) / 2 ;
            //命中了
            if(arr[mid] == tag){
                return mid ;
            }
            //落在右侧范围
            else if(arr[mid] < tag){
                min = mid + 1 ;
            }
            //落在左侧范围
            else{
                max = mid - 1 ;
            }
        }
        return -1;
    }
}

/*
以上代码输出结果如下：
-1
3
 */

　　折半查找的最好的时间复杂度为n（1），就是第一次折半时就恰好找到我们想要的值，当然，最坏时间复杂度为：以2为底，n的对数。即””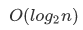

6>.hashmap

　　 哈希map是java中最重要的集合之一，设计非常巧妙，使用通过数组+链表方式组合实现，哈希的目的是让对象在空间内尽可能分散。那么HashMap的时间复杂度是多少呢？

　　　　如果hashmap的每个桶内的元素个数最多不会超过一个常数C，当增加元素时，不断增加桶的数量，而保证每个桶内的元素量固定，因此就可以保证最快的查询速度，则时间复杂度就是O(C*n^2)，去掉系数部分就是O(n^0) = O(1)；

　　　　如果在最坏的情况下就是所有元素进入同一个桶，导致给桶内的链条非常长，则检索数据时，时间复杂度为：O(n)；

7>.矩阵的乘积

矩阵我们按照阶数为n的两个方阵进行计算，矩阵的乘法运算法则为：

 

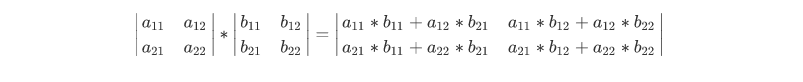
例如：

 

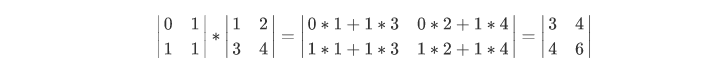
方阵乘法的代码如下：

/*
@author :yinzhengjie
Blog:http://www.cnblogs.com/yinzhengjie/tag/Java%E5%9F%BA%E7%A1%80/
EMAIL:y1053419035@qq.com
*/
package cn.org.yinzhengjie.java.timeComplexity;

public class Demo {
    public static void main(String[] args) {
        int[][] a = {
                {0,1},
                {1,1}
        } ;
        int[][] b = {
                {1,2},
                {3,4}
        } ;

        mulmatrix(a,b);

    }
    /**
     * 两个n阶方阵的乘积
     * @param a
     * @param b
     * @return
     */
    public static void mulmatrix(int[][] a , int[][] b){
        int n = a.length ;
        int[][] r = new int[n][n] ;
        //
        for(int i = 0 ; i < n ; i ++){
            for (int j = 0; j < n; j++) {
                for (int k = 0; k < n; k++) {
                    r[i][j] = r[i][j] + (a[i][k] * b[k][j] ) ;
                }
            }
        }

        for(int i = 0 ; i < n ; i ++){
            for (int j = 0; j < n; j++) {
                System.out.print(r[i][j] + "\t");
            }
            System.out.println();
        }
    }
}

/*
以上代码输出结果如下：
3    4    
4    6
 */

　　方阵的矩阵乘法的计算次数为​，因此时间复杂度为​​O(n^3)。