hdu 3682 10 杭州 现场 C To Be an Dream Architect 容斥 难度:0

C - To Be an Dream Architect

Time Limit:1000MS     Memory Limit:32768KB     64bit IO Format:%I64d & %I64u

Submit Status Practice HDU 3682

Appoint description:  System Crawler  (2014-11-05)

Description

The “dream architect” is the key role in a team of “dream extractors” who enter other’s dreams to steal secrets. A dream architect is responsible for crafting the virtual world that the team and the target will dream into. To avoid the target noticing the world is artificial, a dream architect must have powerful 3D imagination. 

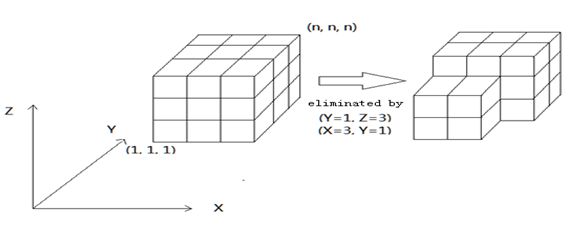
Cobb uses a simple 3D imagination game to test whether a candidate has the potential to be an dream architect. He lets the candidate imagine a cube consisting of n×n×n blocks in a 3D coordinate system as Figure 1. The block at bottom left front corner is marked (1, 1, 1) and the diagonally opposite block is marked (n, n, n). Then he tells the candidate that the blocks on a certain line are eliminated. The line is always parallel to an axis. After m such block eliminations, the candidate is asked to tell how many blocks are eliminated. Note that one block can only be eliminated once even if it is on multiple lines. 

Here is a sample graph according to the first test case in the sample input: 

 

Input

The first line is the number of test cases. 
In each test case, the first line contains two integers n and m( 1 <= n <= 1000, 0 <= m <= 1000).，meaning that the cube is n x n x n and there are m eliminations. 

Each of the following m lines represents an elimination in the following format: 
axis_1=a, axis_2=b 
where axis_i (i=1, 2) is ‘X’ or ‘Y’, or ‘Z’ and axis_1 is not equal to axis_2. a and b are 32-bit signed integers. 

 

Output

For each test case output the number of eliminated blocks.

 

Sample Input

2 3 2 Y=1,Z=3 X=3,Y=1 10 2 X=3,Y=3 Y=3,Z=3

 

Sample Output

5 19

感想:简单的容斥原理,但是统计得头晕眼花,输入也给人找麻烦,姿势还是不好

思路:每次消掉一行,比如消掉的是坐标为(x0,y0),平行z轴的某行,也就是不能有坐标为(x0,y0,*)的三维整数点满足题意,同理,对于(*,y0,z0),(x0,*,z0)也是,(*通配),所以容斥,得到总数

#include <cstdio>
#include <cstring>
using namespace std;
int n,m;
int a,b,c,d;
bool xy[1001][1001],xz[1001][1001],yz[1001][1001];
int xylen[1001],yxlen[1001],yzlen[1001],zxlen[1001],zylen[1001],xzlen[1001];
char buff[100];
int xzheap[1001][2];
int read1(){
    int ans=0;
    for(int i=2;i<=6;i++){
        if(buff[i]>'9'||buff[i]<'0')break;
        ans*=10;
        ans+=buff[i]-'0';
    }
    return ans;
}
int read2(){
    int ans=0;
    int i=6;
    while(buff[i]>'9'||buff[i]<'0'){i++;}
    for(;;i++){
        if(buff[i]>'9'||buff[i]<'0')break;
        ans*=10;
        ans+=buff[i]-'0';
    }
    return ans;
}
void inset(char ch1,char ch2,int num1,int num2){
    if(ch1=='X'){
        if(ch2=='Y'){
            if(!xy[num1][num2]){
                a++;
                xy[num1][num2]=true;
                xylen[num1]++;
                yxlen[num2]++;
            }
        }
        if(ch2=='Z'){
            if(!xz[num1][num2]){
                xzheap[b][0]=num1;
                xzheap[b++][1]=num2;
                xz[num1][num2]=true;
                xzlen[num1]++;
                zxlen[num2]++;
            }
        }
    }
    if(ch1=='Y'){
        if(ch2=='X'){
            if(!xy[num2][num1]){
                a++;
                xy[num2][num1]=true;
                xylen[num2]++;
                yxlen[num1]++;
            }
        }
        if(ch2=='Z'){
            if(!yz[num1][num2]){
                c++;
                yz[num1][num2]=true;
                yzlen[num1]++;
                zylen[num2]++;
            }
        }
    }
    if(ch1=='Z'){
        if(ch2=='X'){
            if(!xz[num2][num1]){
                xzheap[b][0]=num2;
                xzheap[b++][1]=num1;
                xz[num2][num1]=true;
                xzlen[num2]++;
                zxlen[num1]++;
            }
        }
        if(ch2=='Y'){
            if(!yz[num2][num1]){
                c++;
                yz[num2][num1]=true;
                yzlen[num2]++;
                zylen[num1]++;
            }
        }
    }
}
void cl2ear(){
        a=b=c=d=0;
        memset(xy,0,sizeof(xy));
        memset(yz,0,sizeof(yz));
        memset(xz,0,sizeof(xz));
        memset(xylen,0,sizeof(xylen));
        memset(yxlen,0,sizeof(xylen));
        memset(zylen,0,sizeof(xylen));
        memset(yzlen,0,sizeof(xylen));
        memset(xzlen,0,sizeof(xylen));
        memset(zxlen,0,sizeof(xylen));
}
int main(){
    int T;
    scanf("%d",&T);
    while(T--){
        scanf("%d%d",&n,&m);
        cl2ear();
        for(int i=0;i<m;i++){
            scanf("%s",buff);
            bool fl=false;
            char ch1,ch2;int num1=read1(),num2=read2();
            for(int j=0;buff[j];j++){
                if(buff[j]=='X'||buff[j]=='Y'||buff[j]=='Z'){
                    if(!fl){
                        ch1=buff[j];
                        fl=true;
                    }
                    else {
                        ch2=buff[j];
                        break;
                    }
                }
            }
            inset(ch1,ch2,num1,num2);
        }
        for(int i=0;i<b;i++){
            for(int j=1;j<=n;j++){
                if(xy[xzheap[i][0]][j]&&yz[j][xzheap[i][1]])d++;
            }
        }
        int ans=n*a+n*b+n*c+d;
        int a=0;
        for(int i=1;i<=n;i++){
            a+=xylen[i]*xzlen[i]+yzlen[i]*yxlen[i]+zxlen[i]*zylen[i];
        }
        ans-=a;
        printf("%d\n",ans);
    }
    return 0;
}