排书

排书

此题使用 \(IDA*\) 解决。首先是一个迭代加深的 dfs，然后判断条件里是类似于 \(A*\) 的条件，如果当前的估价 \(+\) 当前的实际花费 \(\ge\) 答案就可以返回了。

这里比较显然，无需证明，比 \(A*\) 的证明简单。

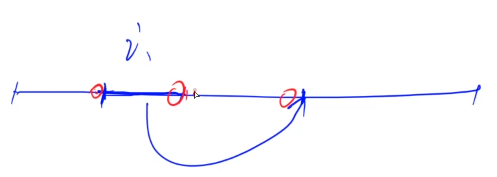
考虑每次都会更改如上图所示的三个数的后继关系，所以每次最多消除 \(3\) 个错误的后继关系。设满足 \(a_{i+1}=a_i+1\) 的数对有 \(tot\) 个，那么至少需要 \(\lceil \dfrac{tot}{3}\rceil=\lfloor \dfrac{tot+2}{3}\rfloor\) 次。

#include<bits/stdc++.h>
using namespace std;
const int N=15;
int n,q[N],w[5][N],depth;
int f(){
	int cnt=2;
	for(int i=0;i<n-1;++i)
		cnt+=q[i+1]!=q[i]+1;
	return cnt/3;
}
bool dfs(int u){
	if(u+f()>depth)return 0;
	if(!f())return 1;
	for(int len=1;len<=n;++len)
		for(int l=0;l+len-1<n;++l){
			int r=l+len-1;
			for(int k=r+1;k<n;++k){
				memcpy(w[u],q,sizeof q);
				int x,y;
				for(x=r+1,y=l;x<=k;++x,++y)q[y]=w[u][x];
				for(x=l;x<=r;++x,++y)q[y]=w[u][x];
				if(dfs(u+1))return 1;
				memcpy(q,w[u],sizeof q);
			}
		}
	return 0;
}
int main(){
	int T;
	cin>>T;
	while(T--){
		cin>>n;
		for(int i=0;i<n;++i)cin>>q[i];
		for(depth=0;depth<5&&!dfs(0);++depth);
		if(depth>=5)puts("5 or more");
		else printf("%d\n",depth);
	}
	return 0;
}