POJ 2553 The Bottom of a Graph

Description

We will use the following (standard) definitions from graph theory. Let V be a nonempty and finite set, its elements being called vertices (or nodes). Let E be a subset of the Cartesian product V×V, its elements being called edges. ThenG=(V,E) is called a directed graph. 
Let n be a positive integer, and let p=(e₁,...,e_n) be a sequence of length n of edges e_i∈E such that e_i=(v_i,v_i+1) for a sequence of vertices (v₁,...,v_n+1). Then p is called a path from vertex v₁ to vertex v_n+1 in G and we say that v_n+1is reachable from v₁, writing (v₁→v_n+1). 
Here are some new definitions. A node v in a graph G=(V,E) is called a sink, if for every node w in G that is reachable from v, v is also reachable from w. The bottom of a graph is the subset of all nodes that are sinks, i.e.,bottom(G)={v∈V|∀w∈V:(v→w)⇒(w→v)}. You have to calculate the bottom of certain graphs.

Input

The input contains several test cases, each of which corresponds to a directed graph G. Each test case starts with an integer number v, denoting the number of vertices of G=(V,E), where the vertices will be identified by the integer numbers in the set V={1,...,v}. You may assume that 1<=v<=5000. That is followed by a non-negative integer e and, thereafter, e pairs of vertex identifiers v₁,w₁,...,v_e,w_e with the meaning that (v_i,w_i)∈E. There are no edges other than specified by these pairs. The last test case is followed by a zero.

Output

For each test case output the bottom of the specified graph on a single line. To this end, print the numbers of all nodes that are sinks in sorted order separated by a single space character. If the bottom is empty, print an empty line.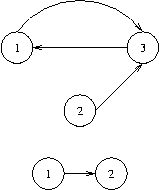

Sample Input

3 3
1 3 2 3 3 1
2 1
1 2
0

Sample Output

1 3
2

Source

Ulm Local 2003

 

题目大意 : 给你一个图，其中有两点u，v如果u能到达v，v也能到达u，那么这种点称为sink，输出所有sink 

 

思路：u可以到达v，v可以到达u，明显是强连通分量，我们可以用tarjan来求。找到所有出度为0的强连通分量输出

 

#include<stack>
#include<cstdio>
#include<cstring>
#include<iostream>
#define MAXN 500005
using namespace std;
int head[MAXN],tot,sum,n,m;
int dfn[MAXN],low[MAXN],belong[MAXN],cnt,f[MAXN];
bool vis[MAXN];
struct node {
    int to;
    int next;
};
node e[MAXN];
stack<int> s;
inline void read(int&x) {
    x=0;int f=1;char c=getchar();
    while(c>'9'||c<'0') {if(c=='-') f=-1;c=getchar();}
    while(c>='0'&&c<='9') {x=(x<<1)+(x<<3)+c-48;c=getchar();}
    x=x*f;
}
inline void MEM() {
    memset(head,0,sizeof head);
    memset(dfn,0,sizeof dfn);
    memset(low,0,sizeof low);
    for(int i=1;i<=n;i++) {
        vis[i]=false;belong[i]=0;f[i]=false;
    }
    sum=0;
}
inline void add(int x,int y) {
    e[++tot].to=y;
    e[tot].next=head[x];
    head[x]=tot;
}
inline void tarjan(int u) {
    dfn[u]=low[u]=++cnt;
    vis[u]=true;s.push(u);
    for(int i=head[u];i;i=e[i].next) {
        int v=e[i].to;
        if(!dfn[v]) {
            tarjan(v);
            low[u]=min(low[u],low[v]);
        }
        else if(vis[v]) low[u]=min(low[u],dfn[v]);
    }
    if(dfn[u]==low[u]) {
        sum++;
        int t;
        do {
            t=s.top();
            s.pop();
            belong[t]=sum;

        }while(u!=t);
    }
}
int main() {
    int x,y;
    while(scanf("%d",&n)&&n) {
        read(m);
        MEM();cnt=0;tot=0;
        for(int i=1;i<=m;i++) {
            read(x);read(y);
            add(x,y);
        }
        for(int i=1;i<=n;i++)
          if(!dfn[i])
            tarjan(i);
        for(int i=1;i<=n;i++) {
            for(int j=head[i];j;j=e[j].next)
              if(belong[e[j].to]!=belong[i]) {
                  f[belong[i]]=true;
                  break;
              }
        }
        for(int i=1;i<=n;i++)
          if(!f[belong[i]]) printf("%d ",i);
        printf("\n");
        while(!s.empty()) s.pop();
    }
    return 0;
}