赫夫曼编码及应用

前言

实验二的代码总体来说比较长的。我用的 C语言 实现的。该代码能够在编译环境 codeblocks 16.01 及 DEV-C++ 5.11 中能够正确运行。关于 cmd gcc 命令行编译或者 Linux 环境下的编译运行。请使用以下命令。

gcc main.c -finput-charset=UTF-8 -fexec-charset=GBK -o main

main.c 是需要编译的文件。

-finput-charset=UTF-8 编译文件对应的编码信息。

-fexec-charset=GBK 指定编译的方式。

main 可执行文件的名字。

注：读取文件为 GBK 格式。

哈夫曼构造全过程

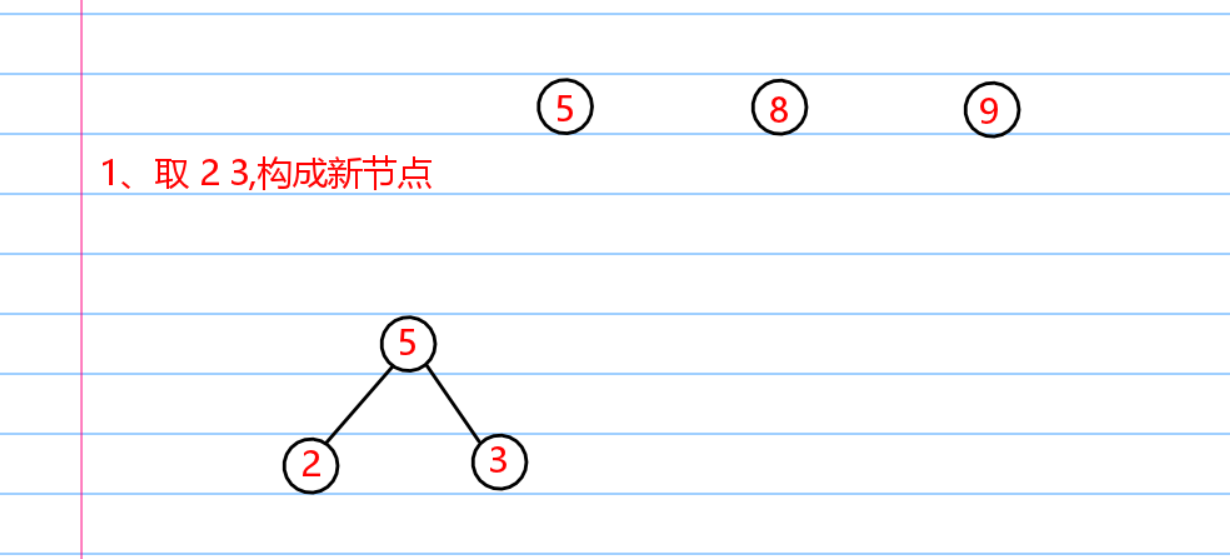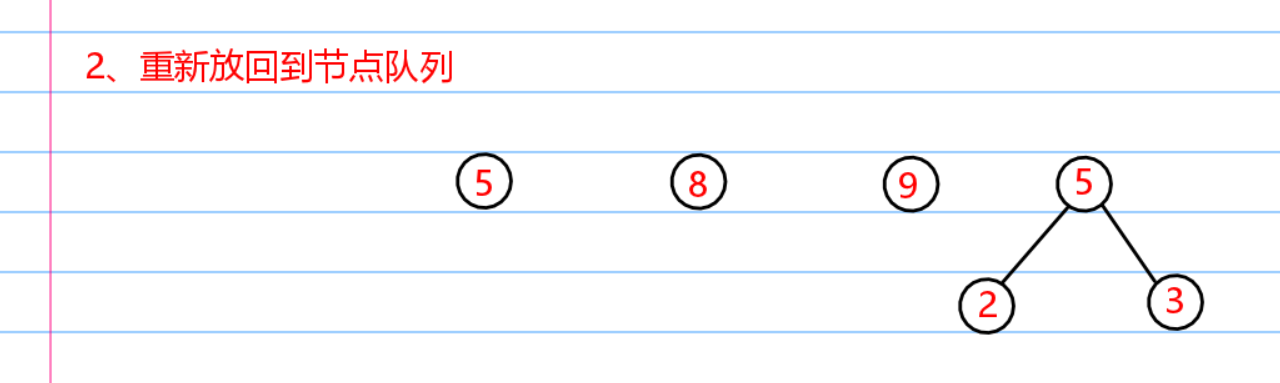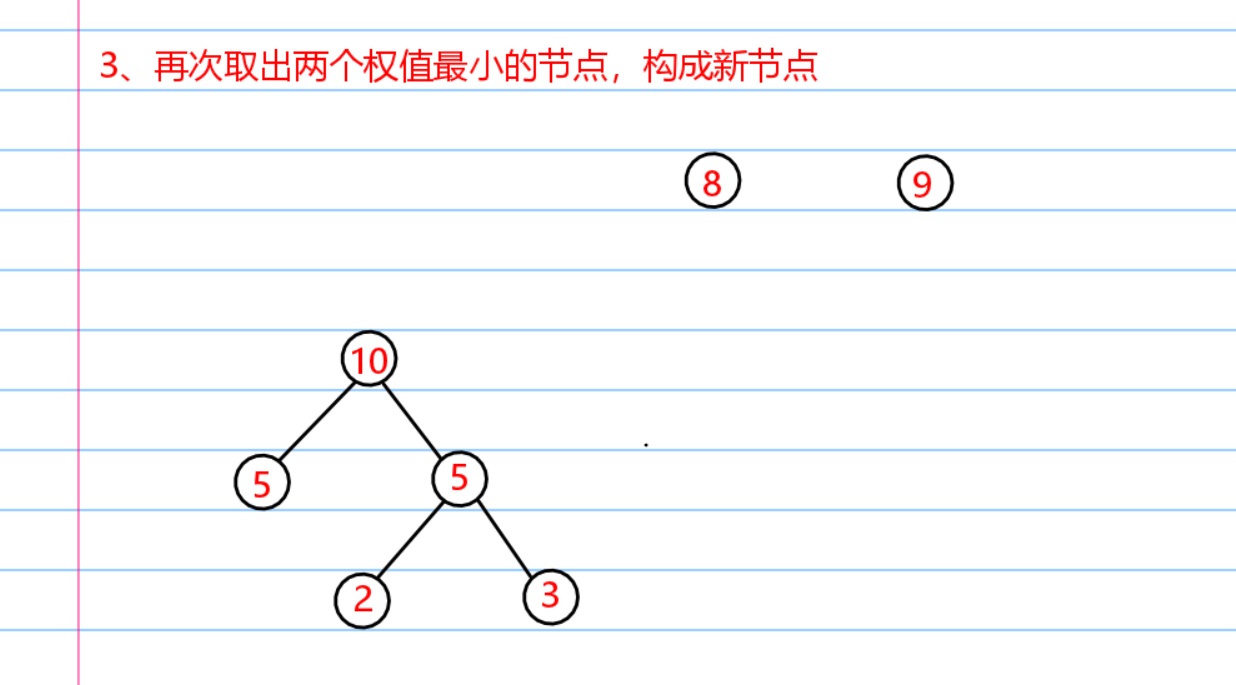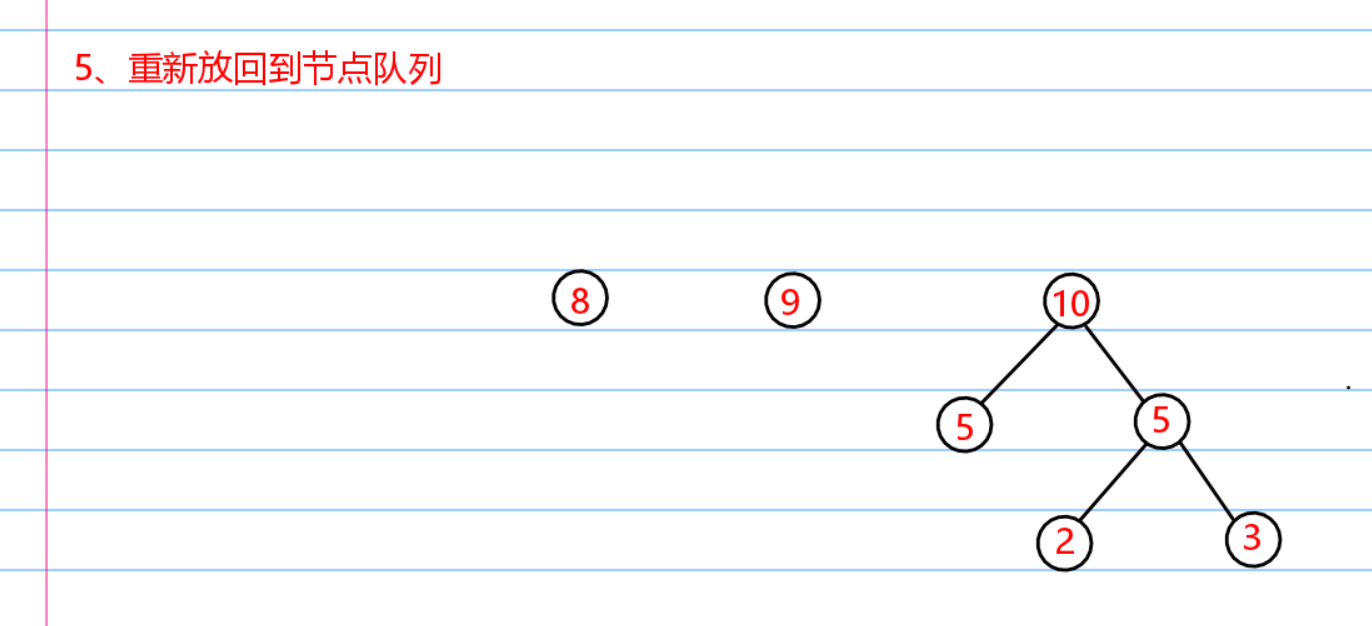img
img
img
img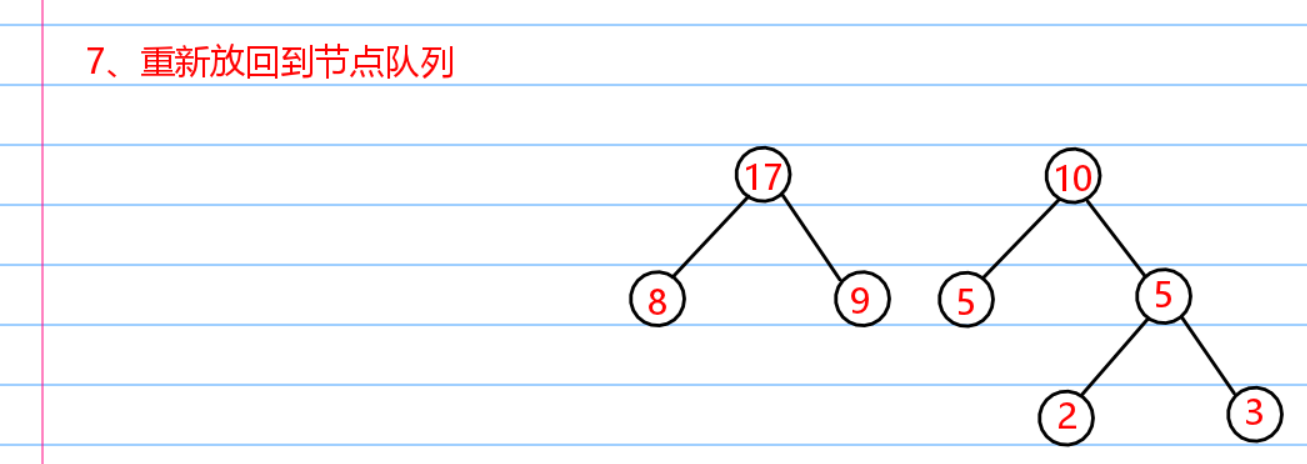
img
img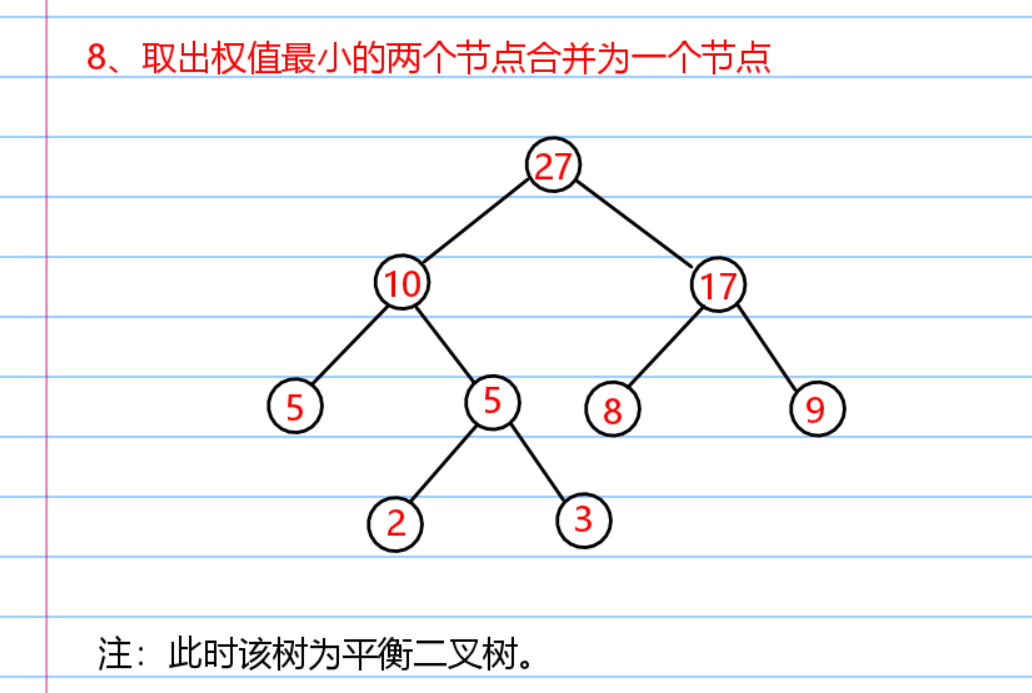
img
img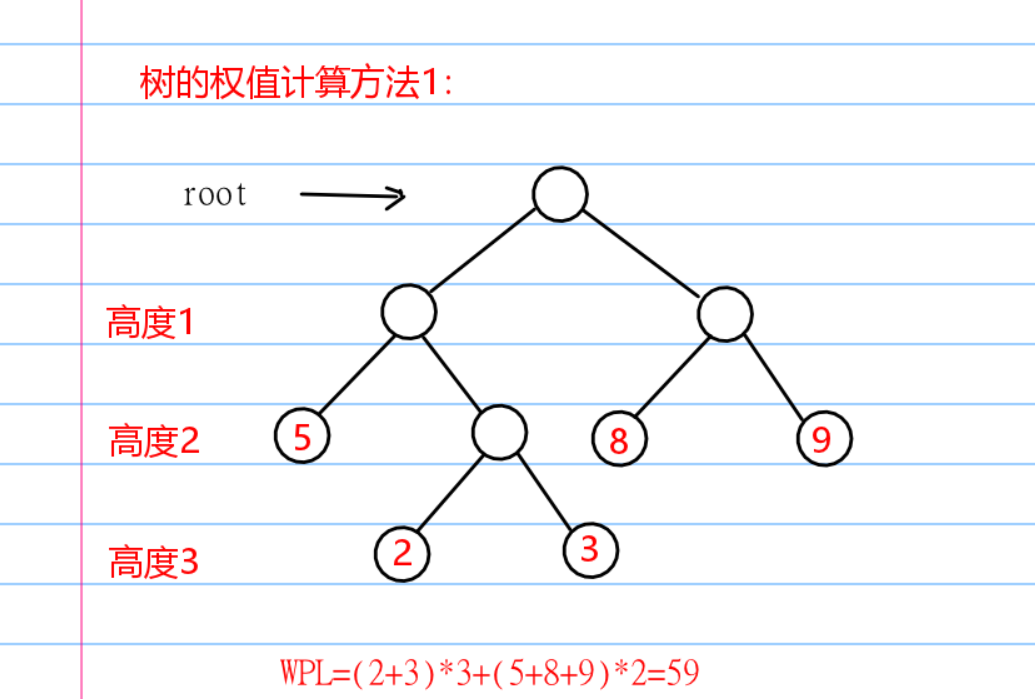
img
img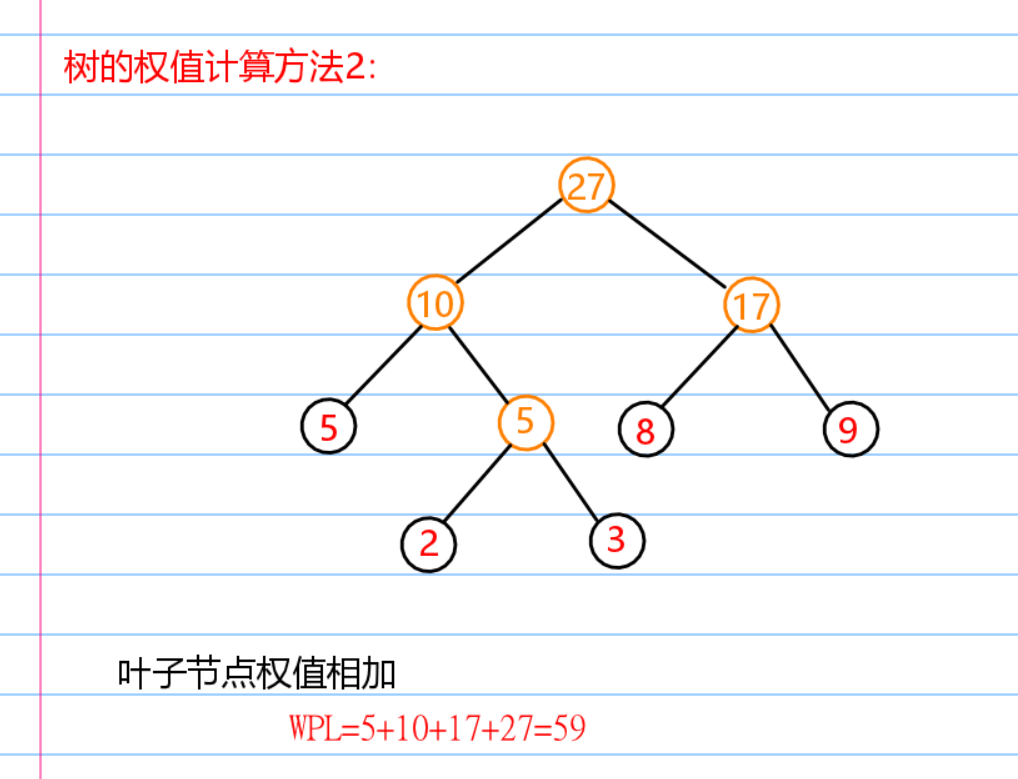
img
img

结果展示

数据文件包含GBK格式下的 中文字符，英文字符，特殊字符等

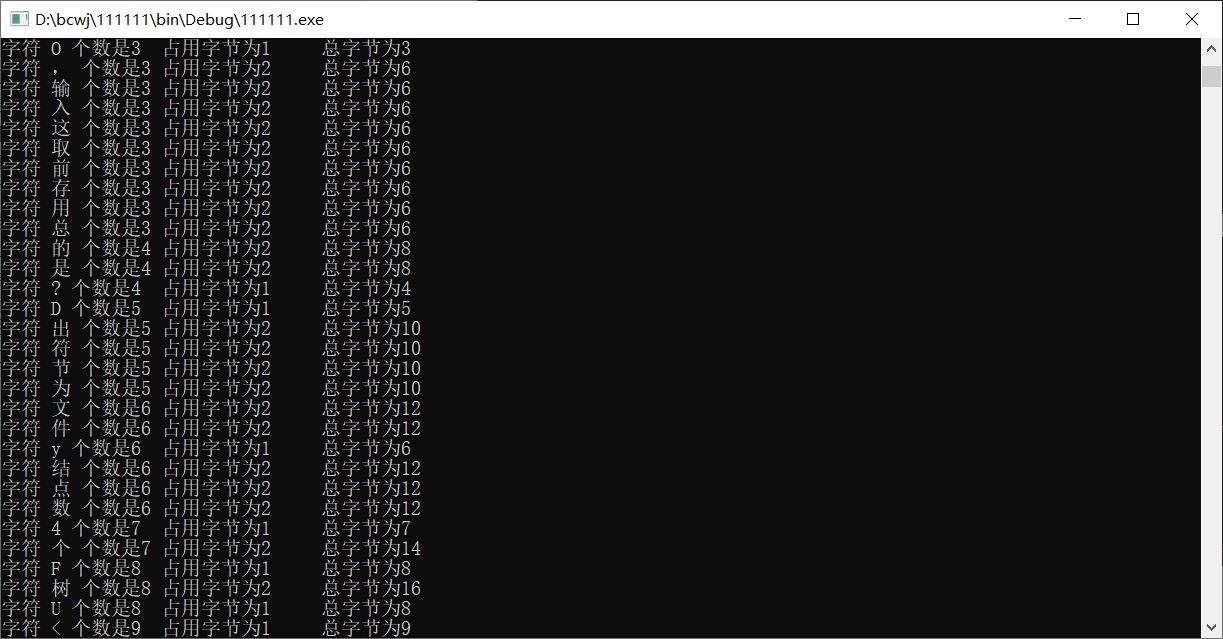
词频统计

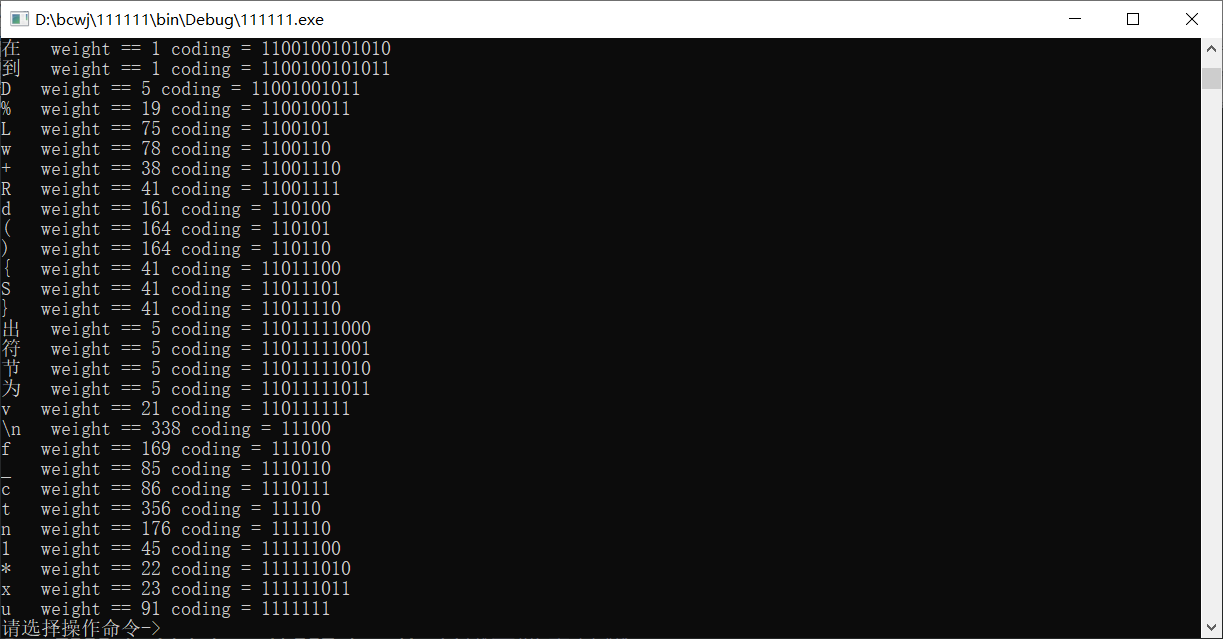
编码展示

文件输出路径信息（在输入的父级目录下，动态改变）

原读取文件展示

加密文件展示

解码文件展示及对比

编码问题

GBK

字符有一字节和双字节编码，00–7F范围内是第一个字节，和ASCII保持一致，此范围内严格上说有96个文字和32个控制符号。

之后的双字节中，前一字节是双字节的第一位。总体上说第一字节的范围是81–FE（也就是不含80和FF），第二字节的一部分领域在40–7E，其他领域在80–FE。

具体来说，定义的是下列字节：

范围	第1字节	第2字节	编码数	字数
水准GBK/1	A1–A9	A1–FE	846	717
水准GBK/2	B0–F7	A1–FE	6,768	6,763
水准GBK/3	81–A0	40–FE (7F除外)	6,080	6,080
水准GBK/4	AA–FE	40–A0 (7F除外)	8,160	8,160
水准GBK/5	A8–A9	40–A0 (7F除外)	192	166
用户定义	AA–AF	A1–FE	564
用户定义	F8–FE	A1–FE	658
用户定义	A1–A7	40–A0 (7F除外)	672
合计：			23,940	21,886

双字节符号可以表达的64K空间如下图所示。绿色和黄色区域是GBK的编码，红色是用户定义区域。没有颜色区域是不正确的代码组合。

UTF-8

• 对于UTF-8编码中的任意字节B，如果B的第一位为0，则B独立的表示一个字符(ASCII码)；
• 如果B的第一位为1，第二位为0，则B为一个多字节字符中的一个字节(非ASCII字符)；
• 如果B的前两位为1，第三位为0，则B为两个字节表示的字符中的第一个字节；
• 如果B的前三位为1，第四位为0，则B为三个字节表示的字符中的第一个字节；
• 如果B的前四位为1，第五位为0，则B为四个字节表示的字符中的第一个字节；

因此，对UTF-8编码中的任意字节，根据第一位，可判断是否为ASCII字符；根据前二位，可判断该字节是否为一个字符编码的第一个字节；根据前四位（如果前两位均为1），可确定该字节为字符编码的第一个字节，并且可判断对应的字符由几个字节表示；根据前五位（如果前四位为1），可判断编码是否有错误或数据传输过程中是否有错误。

确立编码

通过上面可以发现，GBK 编码中文字符少，但是都是双字节，也就是高位和低位。第一个双字节的开始是 81 对应十进制的 129 。

而 UTF-8 编码少数汉字是3个字节，多数汉字是4个字节，统计起来真的不方便，存储都不能存储，怎么统计出现的频率。

于是选择 GBK 编码。

函数和全局变量

函数	功能
void insert(LIST head, LIST tmp)	有序插入结点
LIST find_and_insert(LIST head, LIST tmp)	弹出内部的结点，然后调用insert函数
void output(LIST head)	输出这个读取文件的所有字符统计情况
LIST init_LIST(int high, int low, int weight)	初始化链表信息
TREE tree_node_init(int high, int low, int weight)	初始化哈夫曼树各个结点
TREE build_tree(LIST head)	建立哈夫曼树
void print_huffman_pre(TREE Tree)	前序输出
void update_tree(TREE Tree)	更新树的信息，也即更新编码信息
void save_file(TREE *a, int right, TREE Tree)	保存文件
void to_free_tree(TREE Tree)	释放树
void to_free_list(LIST head)	释放链表
void coding()	编码/加密
void decoding();	解码
TREE queue[1000];	哈夫曼叶子结点信息/密文==密码
int sum_bit_coding = 0;	//编码bit
int sum_bit_decoding = 0;	//解码bit
char file_in[100];	输入文件及路径
char file_out[100];	输出文件及路径
void init_main();	封装/交互

结点详解

typedef struct node *LIST;
typedef struct node *TREE;
struct node
{
    int high;
    int low;
    int weight;
    int code_index;
    char code[100];
    TREE Left;
    TREE Right;
    LIST NEXT;
};

对于每一个需要储存的字符（结点）信息，我们首先标记的是字符对应的十进制整数值。

这里分为高位和低位，主要是为了汉字服务。

高位储存0-127 ASSIC 码的所有字符。

如果高位读取到汉字 > 128 ，那么低位储存下一个字节的信息，也就是说，高位和低位共同储存一个汉字。

weight 储存权重也就是统计字符的个数。

code_index 编码指针

code[100] 编码数组

构建哈夫曼所需要的左右孩子，构建链表需要的NEXT指针

带头结点优化使用

前面的多项式的原理和实现讲解，已经说的很清楚了，头结点有没有的区别和不同之处。

对应博客链接 多项式运算

头结点的二次使用。

head->high 用来存储 ASSIC 0-127 出现的字符总个数 计算输入bits使用。

head->low 用来存储 汉字 出现的字符总个数 计算输入bits使用。

Tree->weight 用来记录不重复的字符个数；即链表的总长度（头结点不算）；又即记录当前构造哈夫曼树的时候的队列剩余的结点个数。

这样的好处是，不用在额外变量记录信息。

文件读取

首先初始化文件指针。

读取方式是按照字符读取，也就是一个一个字节的读取。

但是这个地方我们，需要判断是否是汉字，也就是高位，如果是，读取低位，同存储到这个结点里面，输出的时候同时输出高位和低位就行了。

int ch;
    while((ch = fgetc(P)) != EOF)
    {
        LIST tmp = init_LIST(ch, -1, 1);
        if(ch > 128)
            tmp->low = fgetc(P);
        insert(head, find_and_insert(head, tmp));
    }

关于insert深究

基本的插入方法有头插法和尾插法，这个在之前的实验中说过了。

这次的实验还是采用有序尾插法。

因为要统计权值，也就是字符出现的次数，同时哈夫曼树读取两个结点的时候也是要读取权值最小的，所以，直接采用有序尾插法，如果这个字符出现在这个链表怎么办？我这个地方处理方式是 find_and_insert(head, tmp)，有的话返回该结点，同时权值++，没用的话吗，返回一个初始化的结点，无论哪种结果，都需要重新插入原有的链表。维持链表的有序状态。

LIST find_and_insert(LIST head, LIST tmp)
{
    //统计汉字和其它字符的不重复个数
    if(tmp->low != -1) head->low++;
    else head->high++;
    LIST P = head;
    while(P->NEXT)
    {
        //这个字符相同
        if(P->NEXT->high == tmp->high)
        {
            //汉字相同情况
            if(P->NEXT->low != -1 && tmp->low != -1 && P->NEXT->low == tmp->low)
            {
                //取出当前结点
                LIST found = init_LIST(P->NEXT->high, P->NEXT->low, P->NEXT->weight + 1);
                //删除
                LIST del = P->NEXT;
                P->NEXT = P->NEXT->NEXT;
                del->NEXT = NULL;
                free(del);
                return found;
            }
            if(P->NEXT->low == -1 && tmp->low == -1)
            {
                //取出当前结点
                LIST found = init_LIST(P->NEXT->high, P->NEXT->low, P->NEXT->weight + 1);
                //删除
                LIST del = P->NEXT;
                P->NEXT = P->NEXT->NEXT;
                del->NEXT = NULL;
                free(del);
                return found;
            }
        }
        P = P->NEXT;
    }
    return tmp;
}
void insert(LIST head, LIST tmp)
{
    LIST P = head;
    while(P->NEXT)
    {
        if(tmp->weight < P->NEXT->weight)
            break;
        P = P->NEXT;
    }
    //找不到位置
    if(!P->NEXT)
    {
        P->NEXT = tmp;
        return;
    }
    //insert
    tmp->NEXT = P->NEXT;
    P->NEXT = tmp;
}

建树

上面都是基本的统计情况，包括文件读入，存储，字频统计。

完成之后，就是最重要的环节，建树。

首先把LIST 复制为 TREE（TREE 可以使用链表的所以方法，同时该树，附带头结点，并且权值升序）

基本思路非常明确了，就是不断的取出结点，然后构建成一个树，然后插入到TREE里面。

因为之前我们已经写好了insert函数，所以把结点插入进去还是有序。

同时。之前我们提到 头结点的优化用法 weight 的使用 这个地方就用到了。

记录的是当前队列（森林）（TREE）里面的结点个数（不包含头结点）。

当结点树为1，说明该队列（森林）（TREE）只有一棵树，也就是要求的哈夫曼树。

void coding(TREE Tree)
{
    while(Tree->weight > 1)
    {
        TREE t1 = Tree->NEXT;
        Tree->NEXT = Tree->NEXT->NEXT;
        TREE t2 = Tree->NEXT;
        Tree->NEXT = Tree->NEXT->NEXT;
        //add t1 and t2 to t; 合并树
        TREE t = tree_node_init(-1, -1, t1->weight + t2->weight);
        //左树
        t->Left = t1;
        //右树
        t->Right = t2;
        insert(Tree, t);
        Tree->weight--;
    }
}

编码

树建好了，怎么样才能编码啊，毫无疑问是 层序遍历 编码，因为这样比较简单。

如果从该节点向左，则左孩子的编码为该节点编码 + '0'

如果从该节点向右，则右孩子的编码为该节点编码 + '1'

void update_tree(TREE Tree)
{
    TREE a[1000];
    int left = 0, right = 0;
    if(!Tree) return;
    a[right++] = Tree->NEXT;
    while(left < right)
    {
        //左
        if(a[left]->Left)
        {
            a[right++] = a[left]->Left;
            strcpy(a[left]->Left->code, a[left]->code);
            a[left]->Left->code_index = strlen(a[left]->code);
            a[left]->Left->code[a[left]->Left->code_index++] = '0';
            //a[left]->Left->code[a[left]->Left->code_index] = '\0';
        }
        //右
        if(a[left]->Right)
        {
            a[right++] = a[left]->Right;
            strcpy(a[left]->Right->code, a[left]->code);
            a[left]->Right->code_index = strlen(a[left]->code);
            a[left]->Right->code[a[left]->Right->code_index++] = '1';
            //a[left]->Right->code[a[left]->Right->code_index] = '\0';
        }
        left++;
    }
    save_file(a, right, Tree);
}

加密

直接读取字符，然后写入该字符的密文。

解密

因为是前缀码，该二进制信息唯一，所以不断向后读取字符，然后对比编码信息就行。

后记

window 换行符 \r\n

所以需要特判 if ch == '\n';printf("\r\n")

建树的时候，树是Tree->NEXT开始。（含头结点）。

完整代码

#include <stdio.h>
#include<string.h>
#include<stdlib.h>
typedef struct node *LIST;
typedef struct node *TREE;
struct node
{
    int high;
    int low;
    int weight;
    int code_index;
    char code[100];
    TREE Left;
    TREE Right;
    LIST NEXT;
};
void insert(LIST head, LIST tmp);//有序插入结点
LIST find_and_insert(LIST head, LIST tmp);//弹出内部的结点，然后调用insert函数
void output(LIST head);//输出这个读取文件的所有字符统计情况
LIST init_LIST(int high, int low, int weight);//初始化链表信息
TREE tree_node_init(int high, int low, int weight);//初始化哈夫曼树各个结点
TREE build_tree(LIST head);//建立哈夫曼树
void print_huffman_pre(TREE Tree, int flag);//前序输出,flag 控制是否打印信息
void update_tree(TREE Tree);//更新树的信息，也即更新编码信息
void save_file(TREE *a, int right, TREE Tree);//保存文件
void to_free_tree(TREE Tree);//释放树
void to_free_list(LIST head);//释放链表
void coding();//编码
void decoding();
TREE queue[1000];
int queue_index = 0;
int sum_bit_coding = 0;//编码bit
int sum_bit_decoding = 0;//解码bit
char file_in[100] = "D:\\file.txt";//路径
char file_out[100];
void init_main();
void update_file_out(char file_in[])
{
    int i;
    for(i = strlen(file_in) - 1; i >= 0; i--)
        if(file_in[i] == '\\')
            break;
    int j;
    for(j = 0; j <= i; j++)
        file_out[j] = file_in[j];
}
int main()
{
    init_main();
    return 0;
}
void init_main()
{
    LIST head = init_LIST(0, 0, 0);
    FILE *P;
    while(1)
    {
        printf("为了结果的准确性，请输入GBK格式的txt文件\n");
        printf("请输入需要执行的文件及路径\n例如\nD:\\file.txt\n");
        gets(file_in);
        P = fopen(file_in,"r");
        if (P==NULL)
        {
            printf("文件打开失败\n请重新输入\n");
            continue;
        }
        else
        {
            printf("打开成功\n");
            break;
        }
    }
    update_file_out(file_in);
    int ch;
    while((ch = fgetc(P)) != EOF)
    {
        LIST tmp = init_LIST(ch, -1, 1);
        if(ch > 128)
            tmp->low = fgetc(P);
        insert(head, find_and_insert(head, tmp));
    }
    //output(head);
    TREE Tree = build_tree(head);
    coding(Tree);
    print_huffman_pre(Tree->NEXT, 0);
    update_tree(Tree);
    queue_index = 0;
    print_huffman_pre(Tree->NEXT, 0);
    decoding();
    fclose(P);
    while(1)
    {
        int flag;
        printf("请选择操作命令-> \n \t1：统计信息；\n\t2：词频统计；\n\t3：编码详情；\n\t4：文件输出信息；\n\t5：return\n");
        scanf("%d", &flag);
        switch (flag)
        {
        case 1:
            printf("-----------------------------------\n");
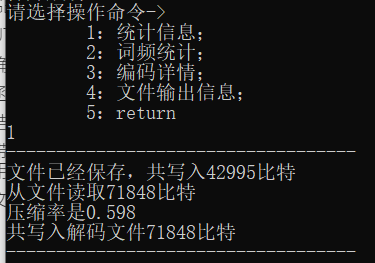
            printf("文件已经保存，共写入%d比特\n", sum_bit_coding);
            printf("从文件读取%d比特\n", 8 * (Tree->high + Tree->low * 2));
            printf("压缩率是%.3f\n",1.0 * sum_bit_coding / 8 / (Tree->high + Tree->low * 2));
            printf("共写入解码文件%d比特\n", sum_bit_decoding);
            printf("-----------------------------------\n");
            break;
        case 2:
            output(head);
            break;
        case 3:
            print_huffman_pre(Tree->NEXT, 1);
            break;
        case 4:
        {
            //路径coding
            char coding_file_name[100];
            strcpy(coding_file_name, file_out);
            strcat(coding_file_name, "coding.txt");
            //路径encoding
            char encoding_file_name[100];
            strcpy(encoding_file_name, file_out);
            strcat(encoding_file_name, "encoding.txt");
            printf("输入文件的路径为%s\n", file_in);
            printf("加密文件的路径为%s\n", coding_file_name);
            printf("解码文件的路径为%s\n", encoding_file_name);
            break;
        }
        case 5:
            return;
        }
    }

    to_free_tree(Tree);
    to_free_list(head);
}
void decoding()
{
    sum_bit_decoding = 0;
    //路径coding
    char coding_file_name[100];
    strcpy(coding_file_name, file_out);
    strcat(coding_file_name, "coding.txt");
    //路径encoding
    char encoding_file_name[100];
    strcpy(encoding_file_name, file_out);
    strcat(encoding_file_name, "encoding.txt");

    FILE *in = fopen(coding_file_name, "r");
    FILE *out = fopen(encoding_file_name, "wb");
    int ch;
    int str_index = 0, left;
    char str[100];
    while((ch = fgetc(in)) != EOF)
    {
        str[str_index++] = ch;
        str[str_index] = '\0';
        for(left = 0; left < queue_index; left++)
        {
            if(strcmp(queue[left]->code, str) == 0)
            {
                //bits
                if(queue[left]->high > 128) sum_bit_decoding += 16;
                else sum_bit_decoding += 8;
                if((char)queue[left]->high == '\n')
                {
                    fprintf(out, "\r\n");
                }
                else
                {
                    fprintf(out, "%c", queue[left]->high);
                    if(queue[left]->high > 128) fprintf(out, "%c", queue[left]->low);
                }
                str_index = 0;
                break;
            }
        }
    }
    fclose(in);
    fclose(out);
}
void to_free_list(LIST head)
{
    LIST P = head;
    while(head->NEXT)
    {
        P = head->NEXT;
        head->NEXT = head->NEXT->NEXT;
        free(P);
    }
    free(head);
}
void to_free_tree(TREE Tree)
{
    if(!Tree) return;
    to_free_tree(Tree->Left);
    to_free_tree(Tree->Right);
    free(Tree);
}
void save_file(TREE *a, int right, TREE Tree)
{
    int left;
    sum_bit_coding = 0;
    FILE *P = fopen(file_in,"r");
    //路径
    char coding_file_name[100];
    strcpy(coding_file_name, file_out);
    strcat(coding_file_name, "coding.txt");

    FILE *out = fopen(coding_file_name, "wb");
    if (P==NULL)
        printf("文件打开失败\n");
    int ch;
    while((ch = fgetc(P)) != EOF)
    {
        LIST tmp = init_LIST(ch, -1, 1);
        if(ch > 128)
            tmp->low = fgetc(P);
        // 查找
        for(left = 0; left < right; left++)
        {
            if(a[left]->high == tmp->high)
            {
                if(tmp->high > 128 && tmp->low == a[left]->low)
                {
                    fprintf(out, "%s", a[left]->code);
                    sum_bit_coding += strlen(a[left]->code);
                }
                if(tmp->high <= 128)
                {
                    fprintf(out, "%s", a[left]->code);
                    sum_bit_coding += strlen(a[left]->code);
                }
            }
        }
        free(tmp);
    }
    fclose(P);
    fclose(out);
}
void update_tree(TREE Tree)
{
    TREE a[1000];
    int left = 0, right = 0;
    if(!Tree) return;
    a[right++] = Tree->NEXT;
    while(left < right)
    {
        //左
        if(a[left]->Left)
        {
            a[right++] = a[left]->Left;
            strcpy(a[left]->Left->code, a[left]->code);
            a[left]->Left->code_index = strlen(a[left]->code);
            a[left]->Left->code[a[left]->Left->code_index++] = '0';
            //a[left]->Left->code[a[left]->Left->code_index] = '\0';
        }
        //右
        if(a[left]->Right)
        {
            a[right++] = a[left]->Right;
            strcpy(a[left]->Right->code, a[left]->code);
            a[left]->Right->code_index = strlen(a[left]->code);
            a[left]->Right->code[a[left]->Right->code_index++] = '1';
            //a[left]->Right->code[a[left]->Right->code_index] = '\0';
        }
        left++;
    }
    save_file(a, right, Tree);
}
TREE tree_node_init(int high, int low, int weight)
{
    TREE tmp = (TREE)malloc(sizeof(struct node));
    tmp->high = high;
    tmp->low = low;
    tmp->weight = weight;
    strcpy(tmp->code, "\0");
    tmp->code_index = 0;
    tmp->Right = NULL;
    tmp->Left = NULL;
    tmp->NEXT = NULL;
    return tmp;
}
TREE build_tree(LIST head)
{
    //copy head
    TREE Tree = tree_node_init(head->high, head->low, 0);
    TREE T = Tree;
    LIST P = head->NEXT;
    while(P)
    {
        T->NEXT = tree_node_init(P->high, P->low, P->weight);
        T = T->NEXT;
        //结点数
        Tree->weight++;
        P = P->NEXT;
    }
    //output(Tree);
    return Tree;
}
void coding(TREE Tree)
{
    while(Tree->weight > 1)
    {
        TREE t1 = Tree->NEXT;
        Tree->NEXT = Tree->NEXT->NEXT;
        TREE t2 = Tree->NEXT;
        Tree->NEXT = Tree->NEXT->NEXT;
        //add t1 and t2 to t; 合并树
        TREE t = tree_node_init(-1, -1, t1->weight + t2->weight);
        //左树
        t->Left = t1;
        //右树
        t->Right = t2;
        insert(Tree, t);
        Tree->weight--;
    }
}
void print_huffman_pre(TREE Tree, int flag)
{
    //遍历
    if(!Tree) return;
    if((char)Tree->high == '\n')
    {
        queue[queue_index++] = Tree;
        if(flag)
            printf("\\n   weight == %d coding = %s\n", Tree->weight, Tree->code);
    }
    else if(Tree->high > 128)
    {
        queue[queue_index++] = Tree;
        if(flag)
        {
            putchar(Tree->high);
            putchar(Tree->low);
            printf("   weight == %d coding = %s\n", Tree->weight, Tree->code);
        }
    }
    else if(Tree->high != -1)
    {
        queue[queue_index++] = Tree;
        if(flag)
        {
            putchar(Tree->high);
            printf("   weight == %d coding = %s\n", Tree->weight, Tree->code);
        }
    }
    print_huffman_pre(Tree->Left, flag);
    print_huffman_pre(Tree->Right, flag);
}
LIST find_and_insert(LIST head, LIST tmp)
{
    //统计汉字和其它字符的不重复个数
    if(tmp->low != -1) head->low++;
    else head->high++;
    LIST P = head;
    while(P->NEXT)
    {
        //这个字符相同
        if(P->NEXT->high == tmp->high)
        {
            //汉字相同情况
            if(P->NEXT->low != -1 && tmp->low != -1 && P->NEXT->low == tmp->low)
            {
                //取出当前结点
                LIST found = init_LIST(P->NEXT->high, P->NEXT->low, P->NEXT->weight + 1);
                //删除
                LIST del = P->NEXT;
                P->NEXT = P->NEXT->NEXT;
                del->NEXT = NULL;
                free(del);
                return found;
            }
            if(P->NEXT->low == -1 && tmp->low == -1)
            {
                //取出当前结点
                LIST found = init_LIST(P->NEXT->high, P->NEXT->low, P->NEXT->weight + 1);
                //删除
                LIST del = P->NEXT;
                P->NEXT = P->NEXT->NEXT;
                del->NEXT = NULL;
                free(del);
                return found;
            }
        }
        P = P->NEXT;
    }
    return tmp;
}
void insert(LIST head, LIST tmp)
{
    LIST P = head;
    while(P->NEXT)
    {
        if(tmp->weight < P->NEXT->weight)
            break;
        P = P->NEXT;
    }
    //找不到位置
    if(!P->NEXT)
    {
        P->NEXT = tmp;
        return;
    }
    //insert
    tmp->NEXT = P->NEXT;
    P->NEXT = tmp;
}
void output(LIST head)
{
    LIST P = head->NEXT;
    while(P)
    {
        if((char)P->high == '\n')
        {
            printf("字符 \\n 个数是%d\t占用字节为%d\t总字节为%d\n", P->weight, P->low == -1 ? 1 : 2, P->weight * (P->low == -1 ? 1 : 2));
            P = P->NEXT;
            continue;
        }
        printf("字符 ");
        putchar(P->high);
        if(P->high > 128)
            putchar(P->low);
        printf(" 个数是%d\t占用字节为%d\t总字节为%d\n", P->weight, P->low == -1 ? 1 : 2, P->weight * (P->low == -1 ? 1 : 2));
        P = P->NEXT;
    }
    printf("总字节数为%d\n", head->high + head->low * 2);
}
LIST init_LIST(int high, int low, int weight)
{
    LIST tmp = (LIST)malloc(sizeof(struct node));
    tmp->high = high;
    tmp->low = low;
    tmp->weight = weight;
    tmp->NEXT = NULL;
    return tmp;
}