baidu2

cf

red and blue

题目链接
题目大意：

思路:
将数组先按颜色，再按大小升序排序所得形式类似于

其中根据贪心只要能出现1~n的序列即可
在颜色B下，\(a[i] >= i + 1\) 因\(a[i]\)只能取不大于自身的数
在颜色R下，\(a[i] <= i + 1\) 因\(a[i]\)只能取不小于自身的数

#include <iostream>
#include <cstring>
#include <queue>
#include <algorithm>
#include <cmath>
#include <stack>
#include <vector>
#include <map>
#include <set>
#include <array>
using namespace std;
#define x first
#define y second
typedef pair<int, int> PII;
typedef long long LL;
const int N = 2e5 + 10;
int n,T;
struct Node{
    int num;
    char color;
};
Node node[N];
bool cmp(Node e1, Node e2) {
    if(e1.color != e2.color) {
        return e1.color < e2.color;
    } else {
        return e1.num < e2.num;
    }
}
bool check(Node node[], int len) {
    for(int i = 0; i < n; i++) {
        if(node[i].color != 'B') {
            break;
        }
        if(node[i].num < i + 1) {
            return false;
        }
    }
    for(int i = n - 1; i >=0; i--) {
        if(node[i].color != 'R') {
            break;
        }
        if(node[i].num > i + 1) {
            return false;
        }
    }
    return true;
}
int main() {
    cin>>T;
    while(T--) {
        cin>>n;
        for(int i = 0; i < n; i++) {
            cin>>node[i].num;
        }
        string s;
        cin>>s;
        for(int i = 0; i < n; i++) {
            node[i].color=s[i];
        }
        sort(node, node + n, cmp);
        // for(int i = 0; i < n; i++) {
        //     cout<<node[i].num<<" "<<node[i].color<<endl;
        // }
        if(check(node, n)) {
            cout<<"YES"<<endl;
        } else {
            cout<<"NO"<<endl;
        }
    }

    return 0;
}

正反卡牌

题目链接
题目大意：

思路:
在一张卡牌中假设有\(a[i] > b[i]\)，将卡牌按照\(a[i] + b[i]\)排序，可以证明在两张卡牌中有\(b[j]-a[i]<b[i]-a[j]\)

\[\begin{equation} \begin{aligned} a[i] + b[i] < a[j] + b[j] \\ 有 a[j] - b[i] < a[i] - b[j] \space (1)\\ b[j] - a[i] < b[i] - a[j] \space (2) \\ 可以证明不等式左侧(2)<(1) \\ a[j] > b[j] \\ -b[i] > -a[i] \end{aligned} \end{equation} \]

因此可以将\(a[i] + b[i] < a[j] + b[j]\)中选\(b[j] - a[i]\)来获得最优解
此时将正反面的和最大的一半序列取b[j]最小的一半取-a[i]来获得最优解

#include <iostream>
#include <cstring>
#include <queue>
#include <algorithm>
#include <cmath>
#include <stack>
#include <vector>
#include <map>
#include <set>
#include <array>
using namespace std;
#define x first
#define y second
typedef pair<int, int> PII;
typedef long long LL;
const int N = 5e5+10;
struct Node {
    int a;
    int b;
};
Node card[N];
int n;

bool cmp(Node e1, Node e2) {
    return e1.a + e1.b < e2.a + e2.b;
}
int main() {
    int n;
    cin>>n;
    for(int i = 0; i < n; i++) {
        int x,y;
        cin>>x>>y;
        card[i].a = max(x, y);
        card[i].b = min(x, y);
    }
    sort(card, card + n, cmp);
    LL ans = 0;
    for(int i = 0; i < (n >> 1); i++) {
        ans += card[i].b;
    }
    for(int i = (n >> 1); i < n; i++) {
        ans -= card[i].a;
    }
    cout<<ans<<endl;

    return 0;
}

战神小码哥

题目链接
题目大意：

思路
一种贪心的思路是尽可能按照时间边界来杀敌，但是在一下反例不成立

3
1 5
2 10
2 10

按照以上贪心思路最优解是15，但实际杀两个i=2的收益最高
此时在杀i = 1 时要求有反悔的选择，此时使用堆来存

将敌人按照时间界限进行升序排列，若此时堆内的元素小于当前时间界限那么可以杀这个敌人
如果大于等于当前时间界限那么就要考虑反悔，当堆中最小的价值小于当前考虑的敌人的价值，当然要反悔之前的操作。

#include <iostream>
#include <cstring>
#include <queue>
#include <algorithm>
#include <cmath>
#include <stack>
#include <vector>
#include <map>
#include <set>
#include <array>
using namespace std;
#define x first
#define y second
typedef pair<int, int> PII;
typedef long long LL;
const int N = 1e5 + 10;
priority_queue<int, vector<int>, greater<int>> q;
struct Node{
    int t;
    int val;
};
int n;
Node enemy[N];
LL res;
bool cmp(Node e1, Node e2) {
    return e1.t < e2.t;
}
int main() {
    cin>>n;
    for(int i = 1 ; i <= n; i++) {
        cin>>enemy[i].t>>enemy[i].val;
    }
    sort(enemy + 1, enemy + n + 1, cmp);
    for(int i = 1; i <= n; i++) {
        if(q.size() < enemy[i].t) {
            q.push(enemy[i].val);
        } else {
            if(enemy[i].val > q.top()) {
                q.pop();
                q.push(enemy[i].val);
            }
        }
    }
    while(!q.empty()) {
        res += q.top();
        // cout<<q.top();
        q.pop();
    }
    cout<<res<<endl;

    return 0;
}

天梯赛

题目链接
题目大意：

思路
同样的反悔贪心，但这题在考虑一个人是否纳入时可能要多步操作(如样例)
如果考虑保存最优解的各个部分(选具体的能力为Ai的人会TLE)
此时只保留最优的结果即可

#include <iostream>
#include <cstring>
#include <queue>
#include <algorithm>
#include <cmath>
#include <stack>
#include <vector>
#include <map>
#include <set>
#include <array>
using namespace std;
#define x first
#define y second
typedef pair<int, int> PII;
typedef long long LL;
const int N = 1e5+10;
struct Node {
    int val;
    int limit;
};
Node stu[N];
int tmp[N];
int n;
priority_queue<int, vector<int>, greater<int>> q;
bool cmp(Node e1, Node e2) {
    return e1.limit > e2.limit;
}
int main() {
    cin>>n;
    for(int i = 0; i < n; i++) {
        cin>>stu[i].val>>stu[i].limit;
    }
    sort(stu, stu + n,cmp);
    LL sum = 0;
    LL ans = 0;
    for(int i = 0; i < n; i++) {
        q.push(stu[i].val);
        sum += stu[i].val;
        while(q.size() > stu[i].limit) {
            sum -= q.top();
            q.pop();  
        }
        ans = max(ans, sum);
    }
    cout<<ans<<endl;

    return 0;
}

硬币塔

题目链接
题目大意：

这里指从下到上一共有几个金币
思路
看清金币，银币。一个k级从下到上共有五级: 1银币，k-1级，n金币，k-1级，银币。按照分级递归即可。

#include <iostream>
#include <cstring>
#include <queue>
#include <algorithm>
#include <cmath>
#include <stack>
#include <vector>
#include <map>
#include <set>
#include <array>
using namespace std;
#define x first
#define y second
typedef pair<int, int> PII;
typedef long long LL;
const int N = 45;
LL n, k;
LL coin[N], gold[N];
void init(){
    coin[0] = gold[0] = 1;
    for(int i = 1; i <= n; i++) {
        coin[i] = (coin[i - 1] << 1) + i + 2;
        gold[i] = (gold[i - 1] << 1) + i;
    }
}
LL fd(int n, LL k) {
    if(k == 0) return 0;
    if(n == 0) return 1;
    if(k <= 1) return 0;
    else if(k > 1 && k <= 1 + coin[n - 1]) return fd(n - 1, k - 1);
    else if(k > coin[n - 1] + 1 && k <= 1 + coin[n - 1] + n) return gold[n - 1] + k - (coin[n - 1] + 1);
    else if(k > 1 + coin[n - 1] + n && k <= (coin[n - 1] << 1) + n + 1) return gold[n - 1] + n + fd(n - 1, k - (coin[n - 1] + 1 + n));
    else return gold[n];
}
int main() {
    cin>>n>>k;
    init();
    // for(int i = 0; i < n; i++) {
    //     cout<<coin[i]<<" "<<gold[i]<<endl;
    // }
    cout<<fd(n, k);

    return 0;
}

三角形个数

题目链接
题目大意：

思路
将三角形分为倒立和正三角形。

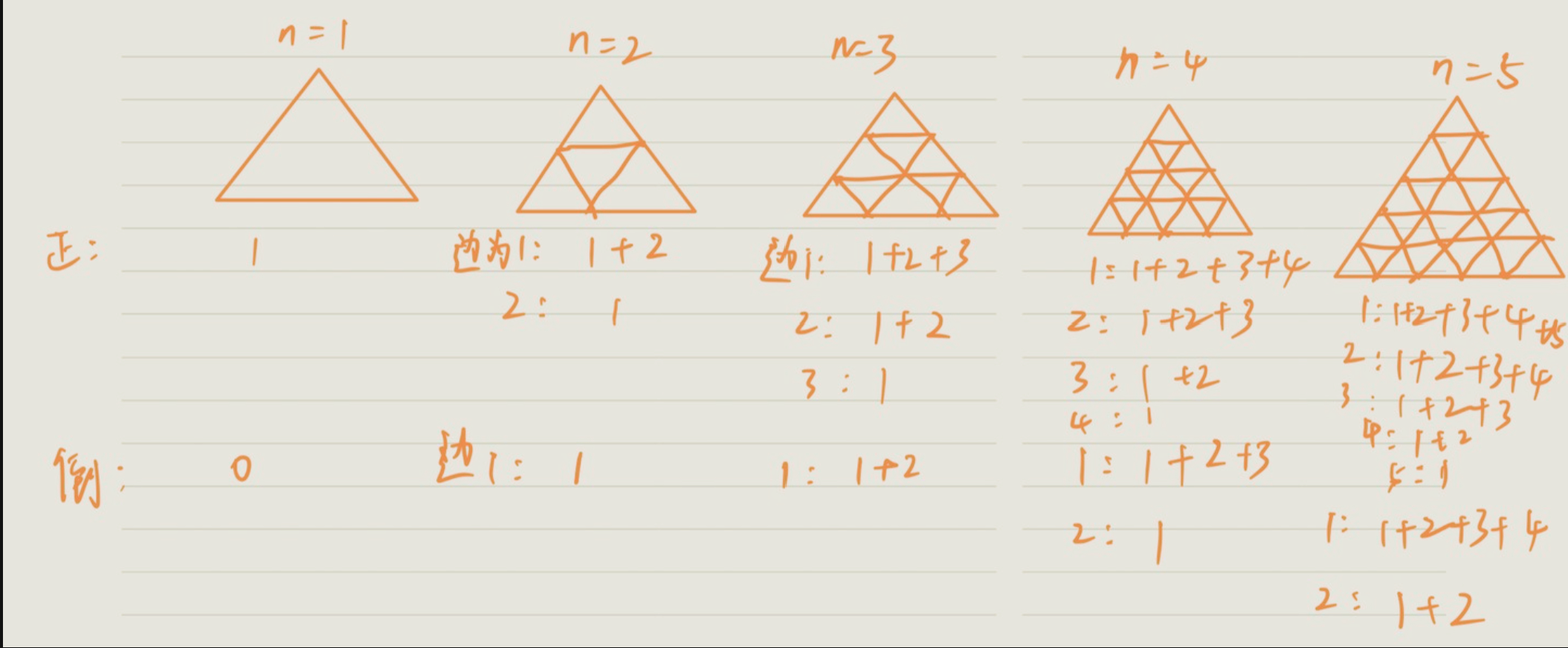
\[\begin{equation} \begin{aligned} sum(n等分) = sum(正) + sum(倒)\\ sum(正) = \sum^{n}_{j =1}\sum^{j}_{i = 1}i \\ sum(倒) = f(n) = f(n - 2) + \sum_{i =1}^{n-1}i \end{aligned} \end{equation} \]

#include <iostream>
#include <cstring>
#include <queue>
#include <algorithm>
#include <cmath>
#include <stack>
#include <vector>
#include <map>
#include <set>
#include <array>
using namespace std;
#define x first
#define y second
typedef pair<int, int> PII;
typedef long long LL;
const int N = 510;
int T;
int s[N],tol[N];
void init() {
    for(int i = 1; i < N; i++) {
        s[i] = s[i - 1] + i;
        tol[i] = tol[i - 1] + i;
    }
    for(int i = 1; i < N; i++) {
        tol[i] = tol[i - 1] + tol[i];
    }
}
int dw(int n) {
    if(n == 1) return 0;
    if(n == 2) return 1;
    return s[n - 1] + dw(n - 2);
}
int main() {
    init();
    cin>>T;
    while(T --) {
        int n;
        cin>>n;
        cout<<(tol[n] + dw(n))<<endl;
    }

    return 0;
}