移位密码（Shift Cipher）体制的加密、解密和破解

移位密码的理论基础是数论中的模运算。

模运算的基本定义：假设a和b均为整数，m是一正整数。若m整除a-b，则可将其表示为a≡b(mod m)，正整数m称为模数。

模运算m上的算术运算定义：令表示集合{0,1,...,m-1}，在其上定义两个运算，加法(+)和乘法(x) —— 运算类似于普通的实数域上的加法和乘法，所不同的只是所得的值是取模以后的余数。

 

因为英文有26个字母，故其一般定义在，则容易验证移位密码满足所定义的密码体制1.1的条件，即对任意的x∈，都有。

若取K=3，则次密码体制通常叫做凯撒密码(Caesar Cipher)，因为它首先被儒勒·凯撒所使用。

 

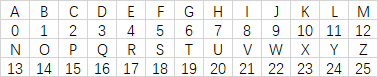
使用移位密码来加密普通的英文句子，首先必须要建立英文字母和模26剩余之间的一一对应关系：如A=1,B=2,...Z=25，如下表所示：

例如，假设移位密码的密钥为K=11，明文为wewillmeetatmidnight。

首先将明文中的字母对应于其相应的整数，得到如下数字串：

22 04 22 08 11 11 12 04 04 19

00 19 12 08 03 13 08 06 07 19

然后，将每个数都与11相加，再对其和取模26运算，可得：

07 15 07 19 22 22 23 15 15 04

11 04 23 19 14 24 19 17 18 04

最后，再将其转换为相应的字符串，即得到密文为HPHTWWXPPELEXTOYTRSE。

要对密文进行解密，只需执行相应的逆过程即可。

 

但是，移位密码（模26）是不安全的，可用密钥穷尽搜索方法来破译，因为密钥空间太小，只有26种可能的情况，可以穷举所有的可能密钥，得到我们所希望的有意义的明文来。 

 

代码实现（Python 3）

'''暴力破解移位密码'''
def hack_shift_cipher(text :str):
    SYMBOLS = 'abcdefghijklmnopqrstuvwxyz'
    for key in range(27):
        translated = ''
        for symbol in text:
            symbol = symbol.lower()
            if symbol in SYMBOLS:
                symbolIndex = SYMBOLS.find(symbol)
                translatedIndex = (symbolIndex - key ) %26
                translated = translated + SYMBOLS[translatedIndex]
            else:
                translated = translated + symbol
        print(f'key={key}: {translated}')


'''移位密码体制加密'''
def shift_cipher_encrypt(text: str, key = 13):
    SYMBOLS = 'ABCDEFGHIJKLMNOPQRSTUVWXYZ'
    translated = ''
    for symbol in text:
        symbol = symbol.upper()
        if symbol in SYMBOLS:
            symbolIndex = SYMBOLS.find(symbol)
            translatedIndex = (symbolIndex + key ) %26
            translated = translated + SYMBOLS[translatedIndex]
        else:
            translated = translated + symbol
    print(translated)


'''移位密码体制解密'''
def shift_cipher_decrypt(text: str, key = 13):
    SYMBOLS = 'abcdefghijklmnopqrstuvwxyz'
    translated = ''
    for symbol in text:
        symbol = symbol.lower()
        if symbol in SYMBOLS:
            symbolIndex = SYMBOLS.find(symbol)
            translatedIndex = (symbolIndex - key ) %26
            translated = translated + SYMBOLS[translatedIndex]
        else:
            translated = translated + symbol
    print(translated)