Codeforces-Round#569 Div.2
前面考试周，抱歉拖了这么久。
A题 Alex and a Rhombus
基本思路
递推解决，n=1时，Count(1)=1；n>1时，Count(n)=Count(n-1)+（n-1）*4
#include<bits/stdc++.h>
using namespace std;
int main()
{
int n;cin>>n;
long long res=1;
for(int i=1;i<=n;i++)
{
res+=4*(i-1);
}
cout<<res;
return 0;
}
B题 Nick and Array
基本思路
按绝对值，负数>正数，因此只要把所有的数转成负数,若n被2整除，则结果为正，若n%2余1，则将最小的负数转成正数。
注意题目要求按顺序输出
#include<bits/stdc++.h>
using namespace std;
int num[100005];
int MINN = 99999,pos=0;
int main()
{
int n,ncount=0;cin>>n;
for(int i=0;i<n;i++)
{
cin >> num[i];
if(num[i]>=0)
num[i] = -num[i] - 1;
if(num[i]<=MINN&&num[i]!=-1)
{
pos=i;
MINN=num[i];
}
}
if(n%2!=0)
{
num[pos]=-num[pos]-1;
}
for(int i=0;i<n;i++)
{
cout<<num[i]<<" ";
}
return 0;
}
C题
基本思路
当双端队列q[0]为最大值时会陷入循环，因此，需要递推的步数不超过\(10^5\)，后面可以用模数组下标来选择输出的第二个数。（记得先pop_front再模n-1）
#include<bits/stdc++.h>
#include<deque>
using namespace std;
struct{
int a,b;
}qu[100010];
int main()
{
deque<int>q;int n,query,maxn=-99999;
cin>>n>>query;
for(int i=0;i<n;i++)
{
int num;cin>>num;
q.push_back(num);
maxn=max(maxn,num);
}
long long cnt=0;
while(q.front()!=maxn)
{
cnt++;
int n1=q.front();
q.pop_front();int n2=q.front();q.pop_front();
q.push_front(max(n1,n2));
q.push_back(min(n1,n2));
qu[cnt].a=n1;qu[cnt].b=n2;
}
q.pop_front();
for(int i=0;i<query;i++)
{
long long m;
cin>>m;
if(m<=cnt)
{
cout<<qu[m].a<<" "<<qu[m].b<<endl;
}
else
{
int pos=(m-cnt-1)%(n-1);
cout<<maxn<<" "<<q[pos]<<endl;
}
}
return 0;
}
D题 Tolik and His Uncle
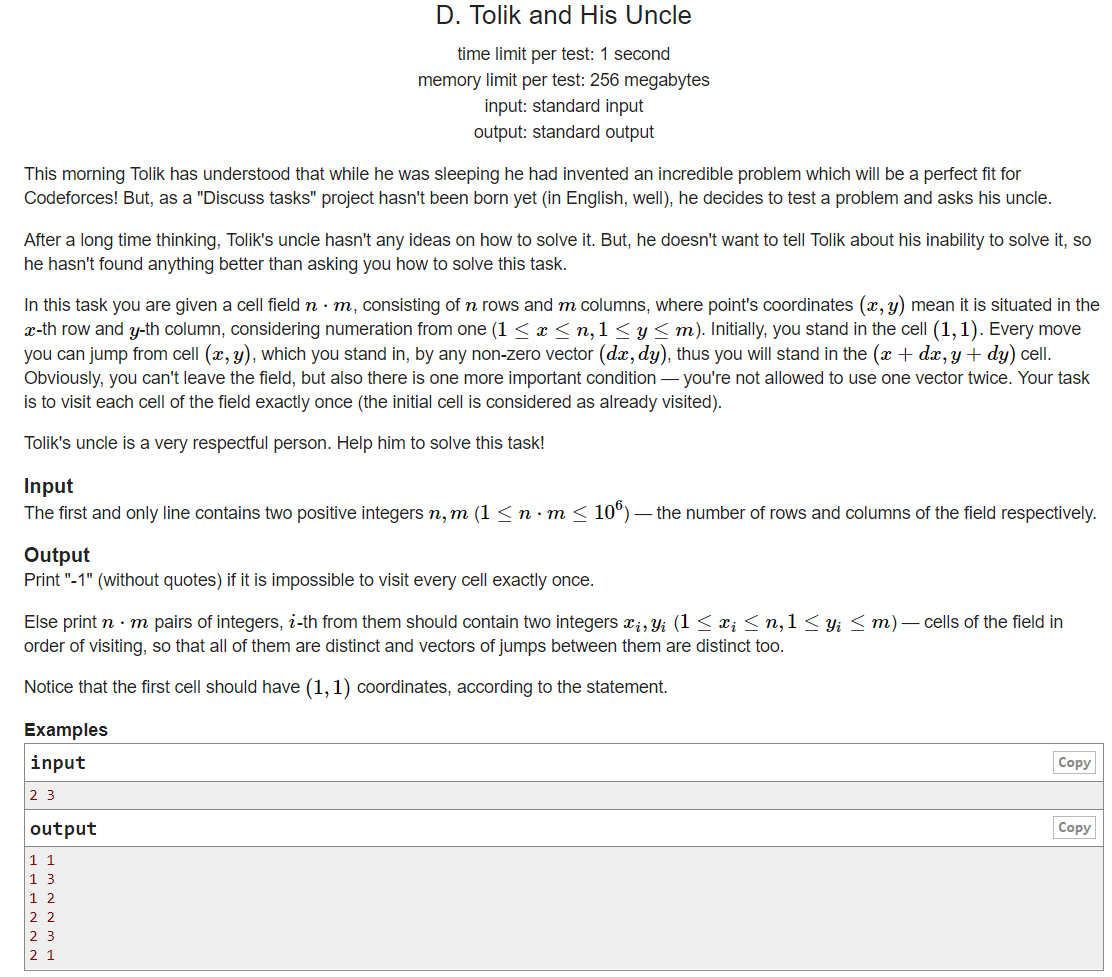
基本思路：
采用对称的思想走，路径就不会重复，假设棋盘为N*M，第一次从（1,1）>（n,m），第二次从（n,m）>（1,2），以此类推。
#include<bits/stdc++.h>
using namespace std;
int main()
{
std::ios::sync_with_stdio(false);
cin.tie(NULL);
int m,n;
cin>>n>>m;
for(int i=1;i<=n/2;i++)
{
for(int j=1;j<=m;j++)
{
cout<<i<< ' '<<j<<'\n';
cout << n-i+1 << ' ' << m-j+1 << '\n';
}
}
if(n%2){
for(int i = 1;i <= m/2;i++){
cout << n/2+1 << ' ' << i << '\n';
cout << n/2+1 << ' ' << m-i+1 << '\n';
}
if(m%2){
cout << n/2+1 << ' ' << m/2+1 << '\n';
}
}
return 0;
}
E题 Serge and Dining Room
基本思路：
采用线段树区间前缀和思想实现查询和修改，建立一个节点包含两组信息：菜肴价格和学生的钱数
//E_std.cpp
#include<bits/stdc++.h>
#define clr(a,b) memset(a,b,sizeof(a))
using namespace std;
typedef long long ll;
const int maxn=1000010;
int n,m,q;
int sum[maxn<<2],maxx[maxn<<2];
int a[maxn],b[maxn];
void update(int o,int l,int r,int p,int val){
if(l==r){
sum[o]+=val;
maxx[o]+=val;
return;
}
int mid=(l+r)>>1;
if(p<=mid)update(o<<1,l,mid,p,val);
else update(o<<1|1,mid+1,r,p,val);
sum[o]=sum[o<<1]+sum[o<<1|1];
maxx[o]=max(maxx[o<<1|1],maxx[o<<1]+sum[o<<1|1]);
//这里的每一个maxx，都是对应的 区间最大后缀和 但不是整根数轴的最大后缀和。
}
struct node{
int max,sum;
};
int query(int o,int l,int r,node tep){
if(l==r){
return l;
}
int mid=(l+r)>>1;
node tep2;
tep2.sum=tep.sum+sum[o<<1|1];
tep2.max=maxx[o<<1|1]+tep.sum;//将 标号为o这段区间的后面一段（o+1）的影响合并到o区间中
if(tep2.max>0){
return query(o<<1|1,mid+1,r,tep);
}
else {
return query(o<<1,l,mid,tep2);
}
}
int main(){
while(cin>>n>>m){
clr(sum,0),clr(maxx,0);
for(int i=1;i<=n;i++){
scanf("%d",&a[i]);
update(1,1,maxn-1,a[i],1);
}
for(int i=1;i<=m;i++){
scanf("%d",&b[i]);
update(1,1,maxn-1,b[i],-1);
}
cin>>q;
while(q--){
int op,pos,val;
scanf("%d%d%d",&op,&pos,&val);
if(op==1){
update(1,1,maxn-1,a[pos],-1);
update(1,1,maxn-1,a[pos]=val,1);
}else{
update(1,1,maxn-1,b[pos],1);
update(1,1,maxn-1,b[pos]=val,-1);
}
if(maxx[1]<=0)puts("-1");
else printf("%d\n",query(1,1,maxn-1,{0,0}));
}
}
}
