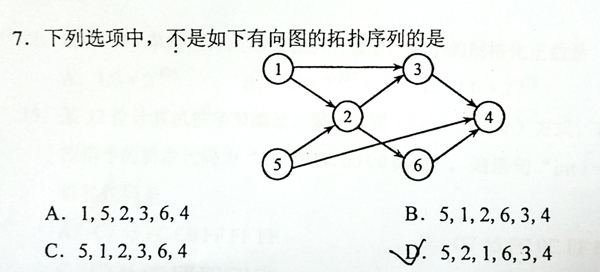
This is a problem given in the Graduate Entrance Exam in 2018: Which of the following is NOT a topological order obtained from the given directed graph? Now you are supposed to write a program to test each of the options.
Input Specification:
Each input file contains one test case. For each case, the first line gives two positive integers N (≤ 1,000), the number of vertices in the graph, and M (≤ 10,000), the number of directed edges. Then M lines follow, each gives the start and the end vertices of an edge. The vertices are numbered from 1 to N. After the graph, there is another positive integer K (≤ 100). Then K lines of query follow, each gives a permutation of all the vertices. All the numbers in a line are separated by a space.
Output Specification:
Print in a line all the indices of queries which correspond to "NOT a topological order". The indices start from zero. All the numbers are separated by a space, and there must no extra space at the beginning or the end of the line. It is graranteed that there is at least one answer.
Sample Input:
6 8
1 2
1 3
5 2
5 4
2 3
2 6
3 4
6 4
5
1 5 2 3 6 4
5 1 2 6 3 4
5 1 2 3 6 4
5 2 1 6 3 4
1 2 3 4 5 6
Sample Output:
3 4
#include <stdio.h> #include <algorithm> #include <set> #include <vector> #include <string> #include <iostream> #include <queue> using namespace std; const int maxn=1001; int a[maxn] ; int n,m,k; vector<int> v; vector<int> adj[maxn]; int degree[maxn]={0}; int store[maxn]={0}; int main(){ scanf("%d %d",&n,&m); for(int i=1;i<=m;i++){ int c1,c2; scanf("%d %d",&c1,&c2); degree[c2]++; adj[c1].push_back(c2); } scanf("%d",&k); for(int i=0;i<k;i++){ for(int j=1;j<=n;j++){ store[j]=degree[j]; } int flag=0; for(int j=0;j<n;j++){ int tmp; scanf("%d",&tmp); if(flag==1){ continue; } else{ if(store[tmp]==0){ for(int q=0;q<adj[tmp].size();q++){ store[adj[tmp][q]]--; } } else{ flag=1; } } } if(flag==1)v.push_back(i); } for(int i=0;i<v.size();i++){ printf("%d%s",v[i],i==v.size()-1?"\n":" "); } }
注意点:一开始没头绪要怎么做,翻了翻算法笔记,看了大佬的思路,发现原来这么方便,只要看进来的这个数入度是否为0。有一个坑就是不要一发现一个数不满足条件了就break,这样后面的输入就读不到了,要记录状态continue





