洛谷 P1073 最优贸易
题目描述
C 国有 n 个大城市和 m 条道路,每条道路连接这 n 个城市中的某两个城市。任意两个
城市之间最多只有一条道路直接相连。这 m 条道路中有一部分为单向通行的道路,一部分
为双向通行的道路,双向通行的道路在统计条数时也计为 1 条。
C 国幅员辽阔,各地的资源分布情况各不相同,这就导致了同一种商品在不同城市的价
格不一定相同。但是,同一种商品在同一个城市的买入价和卖出价始终是相同的。
商人阿龙来到 C 国旅游。当他得知同一种商品在不同城市的价格可能会不同这一信息
之后,便决定在旅游的同时,利用商品在不同城市中的差价赚回一点旅费。设 C 国 n 个城
市的标号从 1~ n,阿龙决定从 1 号城市出发,并最终在 n 号城市结束自己的旅行。在旅游的
过程中,任何城市可以重复经过多次,但不要求经过所有 n 个城市。阿龙通过这样的贸易方
式赚取旅费:他会选择一个经过的城市买入他最喜欢的商品――水晶球,并在之后经过的另
一个城市卖出这个水晶球,用赚取的差价当做旅费。由于阿龙主要是来 C 国旅游,他决定
这个贸易只进行最多一次,当然,在赚不到差价的情况下他就无需进行贸易。
假设 C 国有 5 个大城市,城市的编号和道路连接情况如下图,单向箭头表示这条道路
为单向通行,双向箭头表示这条道路为双向通行。
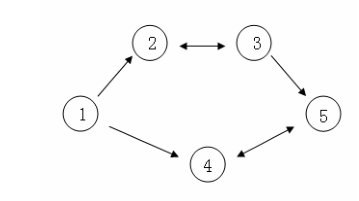
假设 1~n 号城市的水晶球价格分别为 4,3,5,6,1。
阿龙可以选择如下一条线路:1->2->3->5,并在 2 号城市以 3 的价格买入水晶球,在 3
号城市以 5 的价格卖出水晶球,赚取的旅费数为 2。
阿龙也可以选择如下一条线路 1->4->5->4->5,并在第 1 次到达 5 号城市时以 1 的价格
买入水晶球,在第 2 次到达 4 号城市时以 6 的价格卖出水晶球,赚取的旅费数为 5。
现在给出 n 个城市的水晶球价格,m 条道路的信息(每条道路所连接的两个城市的编号
以及该条道路的通行情况)。请你告诉阿龙,他最多能赚取多少旅费。
输入输出格式
输入格式:
第一行包含 2 个正整数 n 和 m,中间用一个空格隔开,分别表示城市的数目和道路的
数目。
第二行 n 个正整数,每两个整数之间用一个空格隔开,按标号顺序分别表示这 n 个城
市的商品价格。
接下来 m 行,每行有 3 个正整数,x,y,z,每两个整数之间用一个空格隔开。如果 z=1,
表示这条道路是城市 x 到城市 y 之间的单向道路;如果 z=2,表示这条道路为城市 x 和城市
y 之间的双向道路。
输出格式:
输出文件 trade.out 共 1 行,包含 1 个整数,表示最多能赚取的旅费。如果没有进行贸易,
则输出 0。
输入输出样例
5 5 4 3 5 6 1 1 2 1 1 4 1 2 3 2 3 5 1 4 5 2
5
说明
【数据范围】
输入数据保证 1 号城市可以到达 n 号城市。
对于 10%的数据,1≤n≤6。
对于 30%的数据,1≤n≤100。
对于 50%的数据,不存在一条旅游路线,可以从一个城市出发,再回到这个城市。
对于 100%的数据,1≤n≤100000,1≤m≤500000,1≤x,y≤n,1≤z≤2,1≤各城市
水晶球价格≤100。
NOIP 2009 提高组 第三题0
1 #include<iostream> 2 #include<cstring> 3 #include<cstdio> 4 #include<vector> 5 #include<queue> 6 #define maxn 100000+5 7 using namespace std; 8 struct Edges{ 9 int from,to,next; 10 }e[maxn*10]; 11 int n,m,dis[maxn],ru[maxn],chu[maxn],head[maxn],tot; 12 bool vis[maxn]; 13 inline void addedge(int u,int v){ 14 /*Edges e;e.from=u;e.to=v; 15 edge.push_back(e);int m=edge.size(); 16 G[u].push_back(m-1);*/ 17 e[++tot].from=u;e[tot].to=v; 18 e[tot].next=head[u];head[u]=tot; 19 } 20 int spfa(int U,int V){ 21 for(int i=1;i<=n;i++)dis[i]=-maxn; 22 dis[U]=0;vis[U]=1;queue<int>q; 23 q.push(U); 24 int u; 25 while(!q.empty()){ 26 u=q.front();q.pop();vis[u]=0; 27 for(int i=head[u];i;i=e[i].next){ 28 bool mark=0; 29 if(dis[e[i].to]<chu[e[i].to]-ru[e[i].from]){ 30 dis[e[i].to]=chu[e[i].to]-ru[e[i].from]; 31 ru[e[i].to]=min(ru[e[i].to],ru[e[i].from]); 32 mark=1; 33 } 34 if(dis[e[i].to]<dis[e[i].from]){ 35 dis[e[i].to]=dis[e[i].from]; 36 ru[e[i].to]=min(ru[e[i].to],ru[e[i].from]); 37 mark=1; 38 } 39 if(mark) 40 if(!vis[e[i].to]) q.push(e[i].to); 41 } 42 } 43 if(dis[V]>0) 44 return dis[V]; 45 return 0; 46 } 47 int main(){ 48 scanf("%d%d",&n,&m); 49 for(int i=1;i<=n;i++){ 50 scanf("%d",&ru[i]); 51 chu[i]=ru[i]; 52 } 53 for(int i=1,x,y,z;i<=m;i++){ 54 scanf("%d%d%d",&x,&y,&z); 55 addedge(x,y); 56 if(z==2) addedge(y,x); 57 } 58 printf("%d",spfa(1,n)); 59 }
莫名的RE-_- -_-

我的思路是ru维护到当前点始最低买入价格、chu维护到当前点时最高卖出价格
来自神犇的AC代码:
1 #include<iostream> 2 #include<cstdio> 3 #include<cstring> 4 #include<algorithm> 5 #include<queue> 6 using namespace std; 7 const int maxn = 100010; 8 const int maxe = 500050; 9 int n,m,tot1,tot2,dis[maxn]; 10 int fst1[maxn],fst2[maxn],minn[maxn],maxx[maxn]; 11 bool vis1[maxn],vis2[maxn]; 12 struct edge{ 13 int to,next; 14 }es1[maxe],es2[maxe]; 15 16 void build1(int ff,int tt) 17 { 18 es1[tot1].to = tt; 19 es1[tot1].next = fst1[ff]; 20 fst1[ff] = tot1++; 21 } 22 23 void build2(int ff,int tt) 24 { 25 es2[tot2].to = tt; 26 es2[tot2].next = fst2[ff]; 27 fst2[ff] = tot2 ++; 28 } 29 30 void spfa1() 31 { 32 queue<int>q; 33 q.push(1); 34 vis1[1] = 1; 35 minn[1] = dis[1]; 36 while(!q.empty()) 37 { 38 int u = q.front(); 39 q.pop(); 40 for(int i = fst1[u];i;i = es1[i].next) 41 { 42 int v = es1[i].to; 43 minn[v] = min(minn[u],dis[v]); 44 if(!vis1[v]) 45 { 46 q.push(v); 47 vis1[v] = 1; 48 } 49 } 50 } 51 } 52 void spfa2() 53 { 54 queue<int>q; 55 q.push(n); 56 vis2[n] = 1; 57 maxx[n] = dis[n]; 58 while(!q.empty()) 59 { 60 int u = q.front(); 61 q.pop(); 62 for(int i = fst2[u];i;i = es2[i].next) 63 { 64 int v = es2[i].to; 65 maxx[v] = max(dis[v],maxx[u]); 66 if(!vis2[v]) 67 { 68 q.push(v); 69 vis2[v] = 1; 70 } 71 } 72 } 73 } 74 int main() 75 { 76 scanf("%d%d",&n,&m); 77 for(int i = 1;i <= n;i ++) 78 { 79 scanf("%d",&dis[i]); 80 vis1[i] = 0; 81 vis2[i] = 0; 82 fst1[i] = 0; 83 fst2[i] = 0; 84 minn[i] = 2147483647; 85 maxx[i] = 0; 86 } 87 for(int i = 1;i <= m;i ++) 88 { 89 int x,y,z; 90 scanf("%d%d%d",&x,&y,&z); 91 if(z == 1) 92 { 93 build1(x,y); 94 build2(y,x); 95 } 96 else 97 { 98 build1(x,y);build1(y,x); 99 build2(x,y);build2(y,x); 100 } 101 } 102 spfa1(); 103 spfa2(); 104 int ans = 0; 105 for(int i = 1;i <= n;i ++) 106 ans = max(ans,maxx[i] - minn[i]); 107 printf("%d",ans); 108 return 0; 109 }
不懂为什么要建反边~~
还有来自神犇的暴力代码:
1 #include<cstdio> 2 #include<iostream> 3 #include<vector> 4 #include<algorithm> 5 using namespace std; 6 #define N 100002 7 int n,m,s[N],maxn[N],minn[N],ans; 8 vector<int>a[N],b[N]; 9 void dfs1(int g ,int h){//g为城市编号,h为当前城市的价格 10 minn[g]=min(h,minn[g]);//因为双向边可以来回,买价可以更低 11 for(int i=0;i<a[g].size();i++) 12 if(h<minn[a[g][i]]) 13 dfs1(a[g][i],min(s[a[g][i]],h)); 14 } 15 void dfs2(int g ,int h){//同理 16 maxn[g]=max(h,maxn[g]); 17 for(int i=0;i<b[g].size();i++) 18 if(h>maxn[b[g][i]]) 19 dfs2(b[g][i],max(s[b[g][i]],h)); 20 } 21 int main(){ 22 scanf("%d%d",&n,&m); 23 for(int i=1;i<=n;i++) 24 scanf("%d",s+i);//每个城市的价格 25 for(int i=1,x,y,z;i<=m;i++){ 26 scanf("%d%d%d",&x,&y,&z); 27 if(z==1) 28 a[x].push_back(y),b[y].push_back(x);//单边 29 else 30 a[x].push_back(y),a[y].push_back(x),b[x].push_back(y),b[y].push_back(x);//双边 31 } 32 for(int i=1;i<=n;i++) 33 maxn[i]=-1e9,minn[i]=1e9; 34 dfs1(1,s[1]);//低价买 35 dfs2(n,s[n]);//高价卖 36 for(int i=1;i<=n;i++) 37 if(maxn[i]!=-1e9&&minn[i]!=1e9) 38 ans=max(ans,maxn[i]-minn[i]); 39 printf("%d\n",ans); 40 return 0; 41 }


