摘要:
  阅读全文
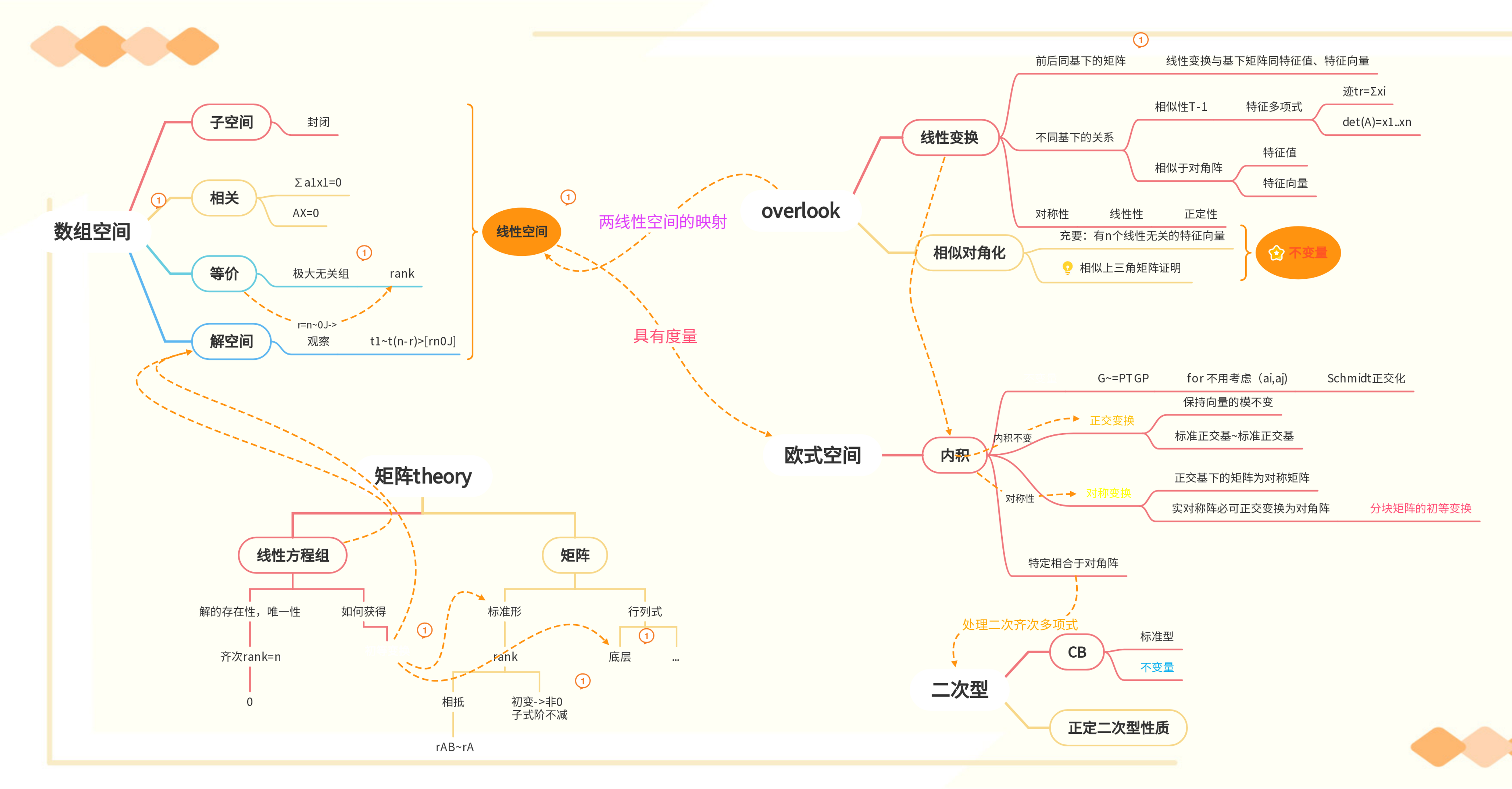
摘要:
代数结构讲述布尔代数与布尔环,布尔表达式,基础是布尔格——有补分配格&模格,还定义了直接积,同态映射相似, 阅读全文
摘要:
2022年3月28日 23:42 施-刘定理的作用——可数性——解为可数无限集合(自然数集为势最小的可数无限集合) 非负性——λ>=0;λ=0——q(x)=0,不取13 正交性——线性无关解yn两两正交 完备性——任意f(X)=Σ0fnyn——fn=Nn(x)^-1∫ρ(X)yn(x)f(x)dx 阅读全文
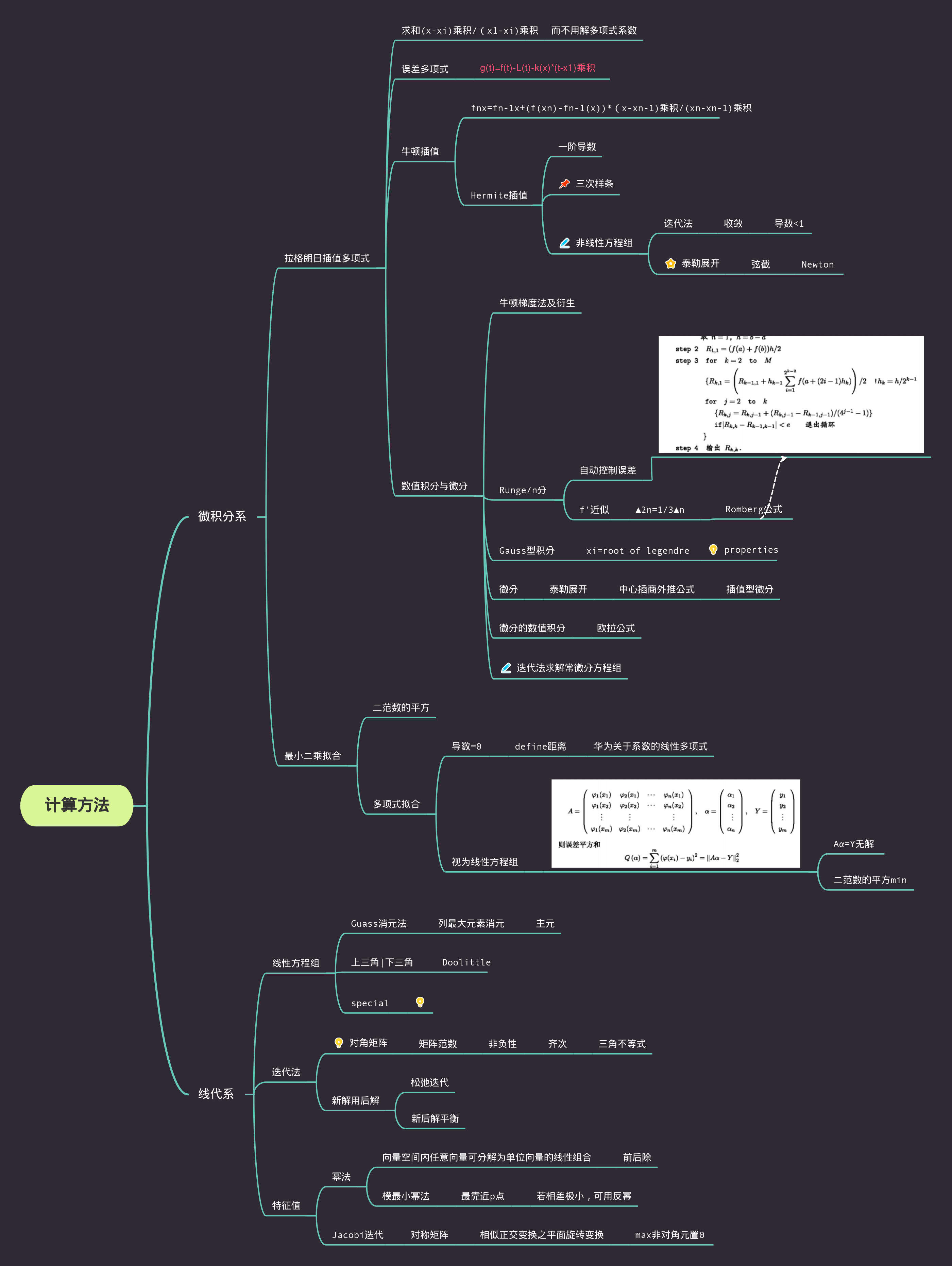
摘要:
   阅读全文
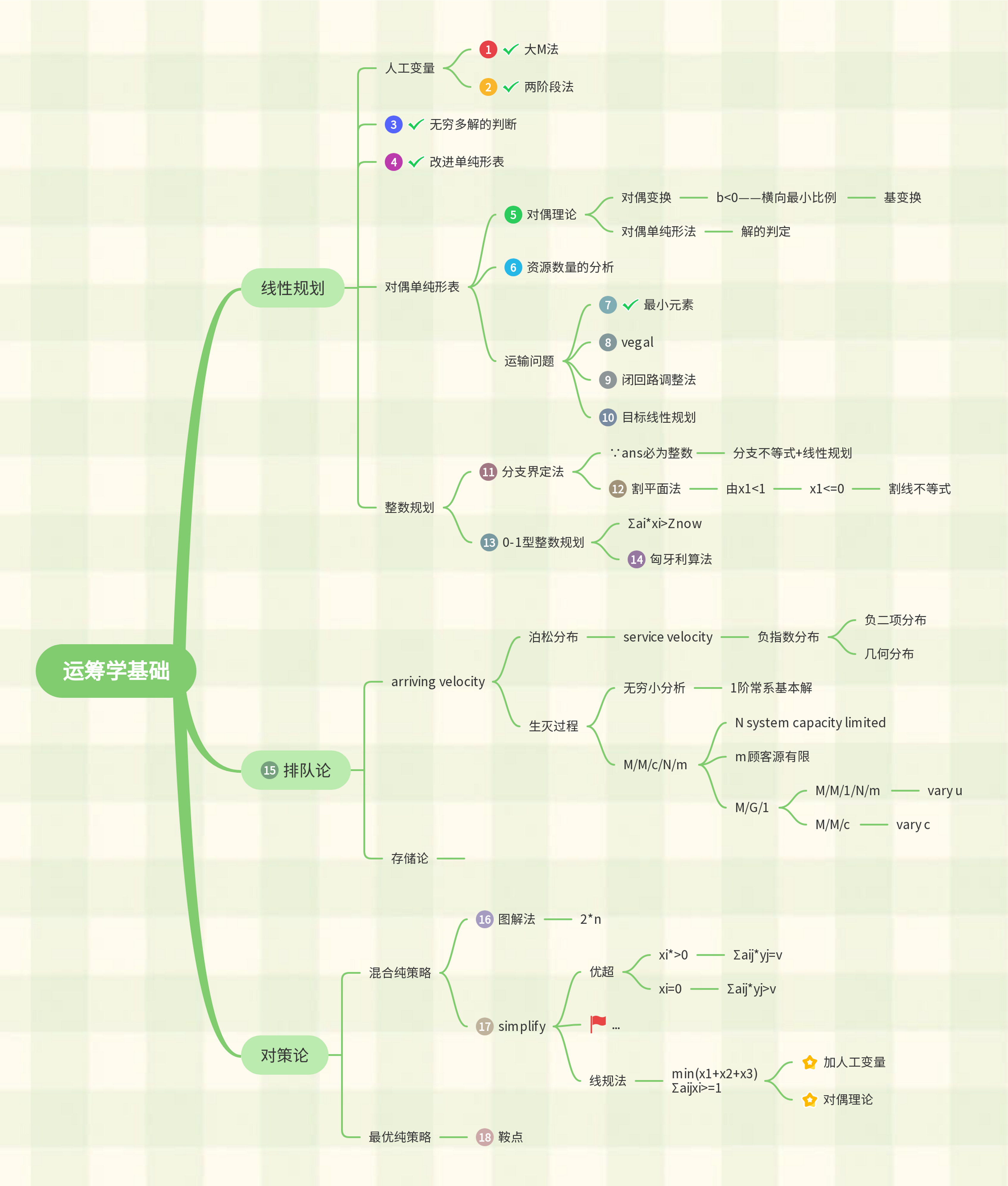
摘要:
 阅读全文
摘要:
随机过程 1.Brown过程为一种特殊的 markov过程,变体为偏移过程Xt=ut+B(t),可以从 a. 无穷小分析,p(t,x)=概率密度, b. 随机变量和,X(t)为正正态分布两角度考虑 符合扩散方程,由向前方程与中心极限定理两种推导可得正态分布N(0,c^2*t) 与markov过程类似 阅读全文

