跳跃表skiplist
本文将总结一种数据结构:跳跃表。前半部分跳跃表性质和操作的介绍直接摘自《让算法的效率跳起来--浅谈“跳跃表”的相关操作及其应用》上海市华东师范大学第二附属中学 魏冉。之后将附上跳跃表的源代码,以及本人对其的了解。难免有错误之处,希望指正,共同进步。谢谢。
跳跃表(Skip List)是1987年才诞生的一种崭新的数据结构,它在进行查找、插入、删除等操作时的期望时间复杂度均为O(logn),有着近乎替代平衡树的本领。而且最重要的一点,就是它的编程复杂度较同类的AVL树,红黑树等要低得多,这使得其无论是在理解还是在推广性上,都有着十分明显的优势。
首先,我们来看一下跳跃表的结构

跳跃表由多条链构成(S0,S1,S2 ……,Sh),且满足如下三个条件:
- 每条链必须包含两个特殊元素:+∞ 和 -∞(其实不需要)
- S0包含所有的元素,并且所有链中的元素按照升序排列。
- 每条链中的元素集合必须包含于序数较小的链的元素集合。
操作
一、查找
目的:在跳跃表中查找一个元素x
在跳跃表中查找一个元素x,按照如下几个步骤进行:
1. 从最上层的链(Sh)的开头开始
2. 假设当前位置为p,它向右指向的节点为q(p与q不一定相邻),且q的值为y。将y与x作比较
(1) x=y 输出查询成功及相关信息
(2) x>y 从p向右移动到q的位置
(3) x<y 从p向下移动一格
3. 如果当前位置在最底层的链中(S0),且还要往下移动的话,则输出查询失败

二、插入
目的:向跳跃表中插入一个元素x
首先明确,向跳跃表中插入一个元素,相当于在表中插入一列从S0中某一位置出发向上的连续一段元素。有两个参数需要确定,即插入列的位置以及它的“高度”。
关于插入的位置,我们先利用跳跃表的查找功能,找到比x小的最大的数y。根据跳跃表中所有链均是递增序列的原则,x必然就插在y的后面。
而插入列的“高度”较前者来说显得更加重要,也更加难以确定。由于它的不确定性,使得不同的决策可能会导致截然不同的算法效率。为了使插入数据之后,保持该数据结构进行各种操作均为O(logn)复杂度的性质,我们引入随机化算法(Randomized Algorithms)。
我们定义一个随机决策模块,它的大致内容如下:
- 产生一个0到1的随机数r r ← random()
- 如果r小于一个常数p,则执行方案A, if r<p then do A
- 否则,执行方案B else do B
初始时列高为1。插入元素时,不停地执行随机决策模块。如果要求执行的是A操作,则将列的高度加1,并且继续反复执行随机决策模块。直到第i次,模块要求执行的是B操作,我们结束决策,并向跳跃表中插入一个高度为i的列。
我们来看一个例子:
假设当前我们要插入元素“40”,且在执行了随机决策模块后得到高度为4
步骤一:找到表中比40小的最大的数,确定插入位置
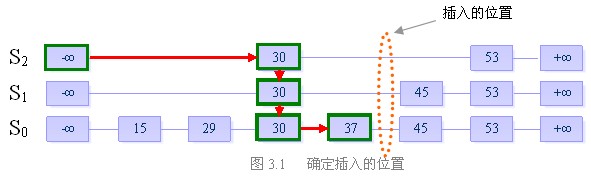
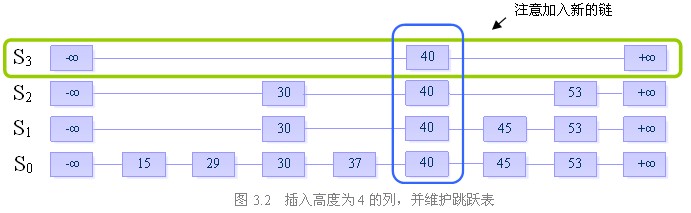
步骤二:插入高度为4的列,并维护跳跃表的结构
三、删除
目的:从跳跃表中删除一个元素x
删除操作分为以下三个步骤:
- 在跳跃表中查找到这个元素的位置,如果未找到,则退出
- 将该元素所在整列从表中删除
- 将多余的“空链”删除
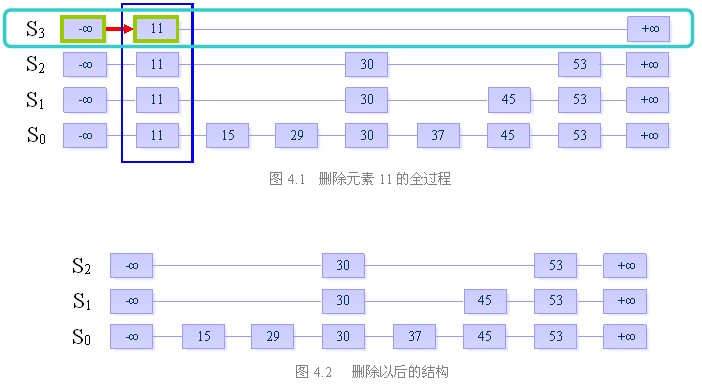
我们来看一下跳跃表的相关复杂度:
空间复杂度: O(n) (期望)
跳跃表高度: O(logn) (期望)
相关操作的时间复杂度:
查找: O(logn) (期望)
插入: O(logn) (期望)
删除: O(logn) (期望)
之所以在每一项后面都加一个“期望”,是因为跳跃表的复杂度分析是基于概率论的。有可能会产生最坏情况,不过这种概率极其微小。
以下是自己学习时碰到的一些问题
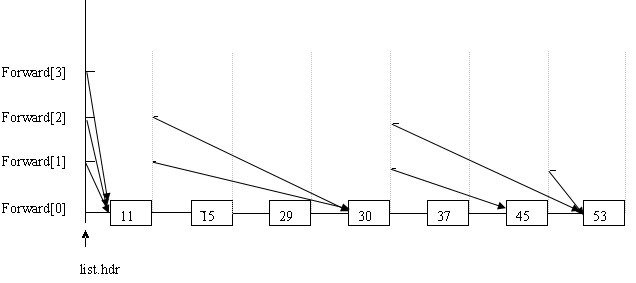
首先分配一个链表,用list.hdr指向,长度为跳跃表规定的最高层,说是链表,在以下代码中只是分配了一段连续的空间,用来指向每一层的开始位置。我们看到结构体nodeType中,有一个key,一个rec(用户数据),还有一个指向结构体的指针数组。
一开始的那些图容易给人误解。如上图所示,例如每个节点的forward[2],就认为是跳跃表的第3层。List.hdr的forward[2]指向11,11的forward[2]指向30,30的forward[2]指向53。这就是跳跃表的第3层:11---30-----53。(准确的说每个forward都指向新节点,新节点的同层forward又指向另一个节点,从而构成一个链表,而数据只有一个,并不是像开始途中所画的那样有N个副本)。本人天资愚钝,看了挺长时间才把它在内存里的结构看清楚了,呵呵。
以下是在网上搜到的一个实现代码
代码中主要注释了insert函数,剩下的两个函数差不多,就不一一注释了

1 /* skip list */ 2 3 #include <stdio.h> 4 #include <stdlib.h> 5 6 /* implementation dependent declarations */ 7 typedef enum { 8 STATUS_OK, 9 STATUS_MEM_EXHAUSTED, 10 STATUS_DUPLICATE_KEY, 11 STATUS_KEY_NOT_FOUND 12 } statusEnum; 13 14 typedef int keyType; /* type of key */ 15 16 /* user data stored in tree */ 17 typedef struct { 18 int stuff; /* optional related data */ 19 } recType; 20 21 #define compLT(a,b) (a < b) 22 #define compEQ(a,b) (a == b) 23 24 /* levels range from (0 .. MAXLEVEL) */ 25 #define MAXLEVEL 15 26 27 typedef struct nodeTag { 28 keyType key; /* key used for searching */ 29 recType rec; /* user data */ 30 struct nodeTag *forward[1]; /* skip list forward pointer */ 31 } nodeType; 32 33 /* implementation independent declarations */ 34 typedef struct { 35 nodeType *hdr; /* list Header */ 36 int listLevel; /* current level of list */ 37 } SkipList; 38 39 SkipList list; /* skip list information */ 40 41 #define NIL list.hdr 42 static int count = 0; 43 statusEnum insert(keyType key, recType *rec) { 44 int i, newLevel; 45 nodeType *update[MAXLEVEL+1]; 46 nodeType *x; 47 count++; 48 /*********************************************** 49 * allocate node for data and insert in list * 50 ***********************************************/ 51 52 /* find where key belongs */ 53 /*从高层一直向下寻找,直到这层指针为NIL,也就是说 54 后面没有数据了,到头了,并且这个值不再小于要插入的值。 55 记录这个位置,留着向其后面插入数据*/ 56 x = list.hdr; 57 for (i = list.listLevel; i >= 0; i--) { 58 while (x->forward[i] != NIL 59 && compLT(x->forward[i]->key, key)) 60 x = x->forward[i]; 61 update[i] = x; 62 } 63 64 65 /*现在让X指向第0层的X的后一个节点*/ 66 x = x->forward[0]; 67 68 69 /*如果相等就不用插入了*/ 70 if (x != NIL && compEQ(x->key, key)) 71 return STATUS_DUPLICATE_KEY; 72 73 /*随机的计算要插入的值的最高level*/ 74 for ( 75 newLevel = 0; 76 rand() < RAND_MAX/2 && newLevel < MAXLEVEL; 77 newLevel++); 78 /*如果大于当前的level,则更新update数组并更新当前level*/ 79 if (newLevel > list.listLevel) { 80 for (i = list.listLevel + 1; i <= newLevel; i++) 81 update[i] = NIL; 82 list.listLevel = newLevel; 83 } 84 85 /* 给新节点分配空间,分配newLevel个指针,则这个 86 节点的高度就固定了,只有newLevel。更高的层次将 87 不会再有这个值*/ 88 if ((x = malloc(sizeof(nodeType) + newLevel*sizeof(nodeType *))) == 0) 89 return STATUS_MEM_EXHAUSTED; 90 x->key = key; 91 x->rec = *rec; 92 93 /* 给每层都加上这个值,相当于往链表中插入一个数*/ 94 for (i = 0; i <= newLevel; i++) { 95 x->forward[i] = update[i]->forward[i]; 96 update[i]->forward[i] = x; 97 } 98 return STATUS_OK; 99 } 100 101 statusEnum delete(keyType key) { 102 int i; 103 nodeType *update[MAXLEVEL+1], *x; 104 105 /******************************************* 106 * delete node containing data from list * 107 *******************************************/ 108 109 /* find where data belongs */ 110 x = list.hdr; 111 for (i = list.listLevel; i >= 0; i--) { 112 while (x->forward[i] != NIL 113 && compLT(x->forward[i]->key, key)) 114 x = x->forward[i]; 115 update[i] = x; 116 } 117 118 119 x = x->forward[0]; 120 121 122 if (x == NIL || !compEQ(x->key, key)) return STATUS_KEY_NOT_FOUND; 123 124 /* adjust forward pointers */ 125 for (i = 0; i <= list.listLevel; i++) { 126 if (update[i]->forward[i] != x) break; 127 update[i]->forward[i] = x->forward[i]; 128 } 129 130 free (x); 131 132 /* adjust header level */ 133 while ((list.listLevel > 0) 134 && (list.hdr->forward[list.listLevel] == NIL)) 135 list.listLevel--; 136 137 return STATUS_OK; 138 } 139 140 statusEnum find(keyType key, recType *rec) { 141 int i; 142 nodeType *x = list.hdr; 143 144 /******************************* 145 * find node containing data * 146 *******************************/ 147 148 for (i = list.listLevel; i >= 0; i--) { 149 while (x->forward[i] != NIL 150 && compLT(x->forward[i]->key, key)) 151 x = x->forward[i]; 152 } 153 x = x->forward[0]; 154 if (x != NIL && compEQ(x->key, key)) { 155 *rec = x->rec; 156 return STATUS_OK; 157 } 158 return STATUS_KEY_NOT_FOUND; 159 } 160 161 void initList() { 162 int i; 163 164 /************************** 165 * initialize skip list * 166 **************************/ 167 168 if ((list.hdr = malloc( 169 sizeof(nodeType) + MAXLEVEL*sizeof(nodeType *))) == 0) { 170 printf ("insufficient memory (initList)/n"); 171 exit(1); 172 } 173 for (i = 0; i <= MAXLEVEL; i++) 174 list.hdr->forward[i] = NIL; 175 list.listLevel = 0; 176 } 177 178 int main(int argc, char **argv) { 179 int i, maxnum, random; 180 recType *rec; 181 keyType *key; 182 statusEnum status; 183 184 185 /* command-line: 186 * 187 * skl maxnum [random] 188 * 189 * skl 2000 190 * process 2000 sequential records 191 * skl 4000 r 192 * process 4000 random records 193 * 194 */ 195 196 maxnum = 20; 197 random = argc > 2; 198 199 initList(); 200 201 if ((rec = malloc(maxnum * sizeof(recType))) == 0) { 202 fprintf (stderr, "insufficient memory (rec)/n"); 203 exit(1); 204 } 205 if ((key = malloc(maxnum * sizeof(keyType))) == 0) { 206 fprintf (stderr, "insufficient memory (key)/n"); 207 exit(1); 208 } 209 210 if (random) { 211 /* fill "a" with unique random numbers */ 212 for (i = 0; i < maxnum; i++) key[i] = rand(); 213 printf ("ran, %d items/n", maxnum); 214 } else { 215 for (i = 0; i < maxnum; i++) key[i] = i; 216 printf ("seq, %d items/n", maxnum); 217 } 218 219 for (i = 0; i < maxnum; i++) { 220 status = insert(key[i], &rec[i]); 221 if (status) printf("pt1: error = %d/n", status); 222 } 223 224 for (i = maxnum-1; i >= 0; i--) { 225 status = find(key[i], &rec[i]); 226 if (status) printf("pt2: error = %d/n", status); 227 } 228 229 for (i = maxnum-1; i >= 0; i--) { 230 status = delete(key[i]); 231 if (status) printf("pt3: error = %d/n", status); 232 } 233 return 0; 234 }
转自:http://blog.csdn.net/topcoder1234/article/details/5841119




