题目:https://pintia.cn/problem-sets/1268384564738605056/problems/1274008636207132672
给定两棵树T1和T2。如果T1可以通过若干次左右孩子互换就变成T2,则我们称两棵树是“同构”的。例如图1给出的两棵树就是同构的,因为我们把其中一棵树的结点A、B、G的左右孩子互换后,就得到另外一棵树。而图2就不是同构的。
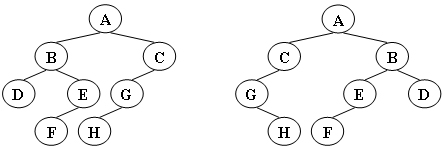
图1
图2
现给定两棵树,请你判断它们是否是同构的。
输入格式:
输入给出2棵二叉树树的信息。对于每棵树,首先在一行中给出一个非负整数N (≤10),即该树的结点数(此时假设结点从0到N−1编号);随后N行,第i行对应编号第i个结点,给出该结点中存储的1个英文大写字母、其左孩子结点的编号、右孩子结点的编号。如果孩子结点为空,则在相应位置上给出“-”。给出的数据间用一个空格分隔。注意:题目保证每个结点中存储的字母是不同的。
输出格式:
如果两棵树是同构的,输出“Yes”,否则输出“No”。
输入样例1(对应图1):
8
A 1 2
B 3 4
C 5 -
D - -
E 6 -
G 7 -
F - -
H - -
8
G - 4
B 7 6
F - -
A 5 1
H - -
C 0 -
D - -
E 2 -
输出样例1:
Yes
输入样例2(对应图2):
8
B 5 7
F - -
A 0 3
C 6 -
H - -
D - -
G 4 -
E 1 -
8
D 6 -
B 5 -
E - -
H - -
C 0 2
G - 3
F - -
A 1 4
输出样例2:
No
题解: https://www.cnblogs.com/zhien-aa/p/6651993.html
代码:
/* Name: hello world.cpp Author: AA Description: 唯代码与你不可辜负 */ #include<bits/stdc++.h> using namespace std; #define LL long long #define maxn 20100 typedef struct Node { char data; int left; int right; } Tree; Tree t1[maxn], t2[maxn]; int build(Tree T[]) { int n, cherk[maxn], root = -1; char cl, cr; scanf("%d", &n); getchar(); if(n) { for(int i = 0; i < n; i++) cherk[i] = 0; for(int i = 0; i < n; i++) { scanf("%c %c %c", &T[i].data, &cl, &cr); getchar(); if(cl != '-') { T[i].left = cl - '0'; cherk[T[i].left] = 1; } else T[i].left = -1; if(cr != '-') { T[i].right = cr - '0'; cherk[T[i].right] = 1; } else T[i].right = -1; } for(int i = 0; i < n; i++) { if(cherk[i] == 0) { root = i; break; } } } return root; } bool Isomorphic(int root1, int root2) { if(root1 == -1 && root2 == -1) return true;//都是空的,符合 else if((root1 == -1 && root2 != -1) || (root1 != -1 && root2 == -1)) return false; //一个空一个不空当然不符合 else if(t1[root1].data != t2[root2].data) return false; //元素不相等不符合 else if(t1[root1].left == -1 && t2[root2].left == -1) //左子树都是空的话,就判断右子树 return Isomorphic(t1[root1].right, t2[root2].right); else if(t1[t1[root1].left].data == t2[t2[root2].left].data)//左子树的元素都相同的话就把他们的左右子树都判断 return (Isomorphic(t1[root1].left, t2[root2].left) && Isomorphic(t1[root1].right, t2[root2].right)); else //最后是把左右子树交换来判断 return (Isomorphic(t1[root1].left, t2[root2].right) && Isomorphic(t1[root1].right, t2[root2].left)); } int main() { int root1, root2; root1 = build(t1); root2 = build(t2); if(Isomorphic(root1, root2)) puts("Yes"); else puts("No"); }




 posted on
posted on

