【leetcode】1557. Minimum Number of Vertices to Reach All Nodes
题目如下:
Given a directed acyclic graph, with
nvertices numbered from0ton-1, and an arrayedgeswhereedges[i] = [fromi, toi]represents a directed edge from nodefromito nodetoi.Find the smallest set of vertices from which all nodes in the graph are reachable. It's guaranteed that a unique solution exists.
Notice that you can return the vertices in any order.
Example 1:
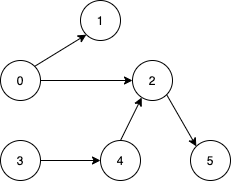
Input: n = 6, edges = [[0,1],[0,2],[2,5],[3,4],[4,2]] Output: [0,3] Explanation: It's not possible to reach all the nodes from a single vertex. From 0 we can reach [0,1,2,5]. From 3 we can reach [3,4,2,5]. So we output [0,3].Example 2:
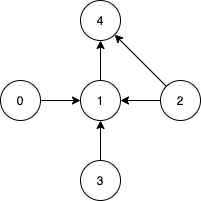
Input: n = 5, edges = [[0,1],[2,1],[3,1],[1,4],[2,4]] Output: [0,2,3] Explanation: Notice that vertices 0, 3 and 2 are not reachable from any other node, so we must include them.
Also any of these vertices can reach nodes 1 and 4.Constraints:
2 <= n <= 10^51 <= edges.length <= min(10^5, n * (n - 1) / 2)edges[i].length == 20 <= fromi, toi < n- All pairs
(fromi, toi)are distinct.
解题思路:因为edges是有向的,所以只需要找出所有edges的end的集合,不在这个集合中的node就是答案。
代码如下:
class Solution(object): def findSmallestSetOfVertices(self, n, edges): """ :type n: int :type edges: List[List[int]] :rtype: List[int] """ dic = {} for start,end in edges: dic[end] = 1 res = [] for i in range(n): if i not in dic:res.append(i) return res