【leetcode】1080. Insufficient Nodes in Root to Leaf Paths

题目如下：

Given the root of a binary tree, consider all root to leaf paths: paths from the root to any leaf.  (A leaf is a node with no children.)

A node is insufficient if every such root to leaf path intersecting this node has sum strictly less than limit.

Delete all insufficient nodes simultaneously, and return the root of the resulting binary tree.

 

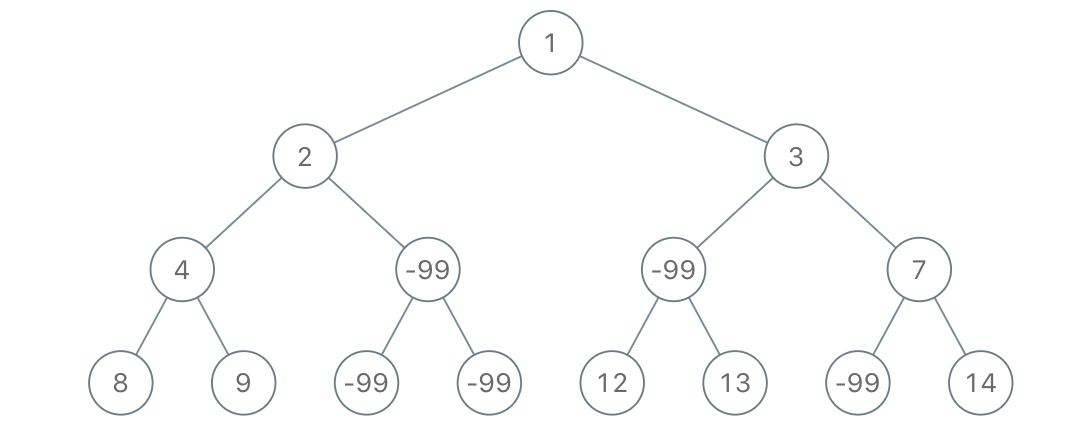
Example 1:

Input: root = [1,2,3,4,-99,-99,7,8,9,-99,-99,12,13,-99,14], limit = 1

Output: [1,2,3,4,null,null,7,8,9,null,14]

Example 2:

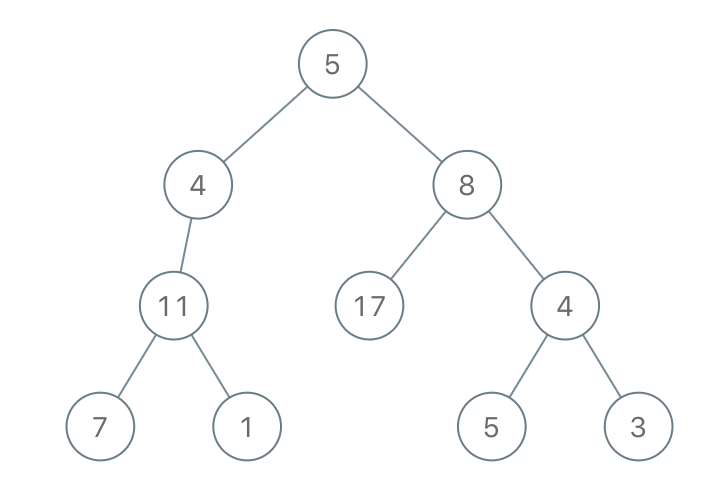
Input: root = [5,4,8,11,null,17,4,7,1,null,null,5,3], limit = 22

Output: [5,4,8,11,null,17,4,7,null,null,null,5]

 

Example 3:

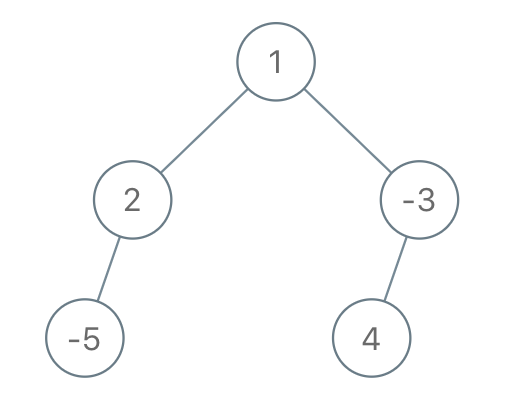Input: root = [1,2,-3,-5,null,4,null], limit = -1

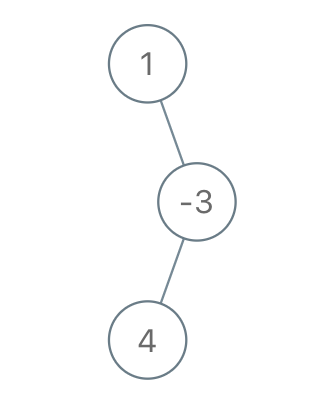Output: [1,null,-3,4]

 

Note:

1. The given tree will have between 1 and 5000 nodes.
2. -10^5 <= node.val <= 10^5
3. -10^9 <= limit <= 10^9

解题思路：我的方法是两次递归，第一次递归求出每个节点所对应路径的最大值并存储在字典中，第二次递归是删除最大值小于limit的节点以及其子树。

代码如下：

# Definition for a binary tree node.
# class TreeNode(object):
#     def __init__(self, x):
#         self.val = x
#         self.left = None
#         self.right = None

class Solution(object):
    dic = {}
    def calculate(self,node,number,amount):
        if node.left == None and node.right == None:
            self.dic[number] = node.val + amount
            return node.val + amount
        left_v = -float('inf')
        right_v = -float('inf')
        if node.left != None:
            left_v = self.calculate(node.left,number*2,node.val + amount)
        if node.right != None:
            right_v = self.calculate(node.right,number*2+1,node.val + amount)
        #node.val = max(node.val,left_v,right_v)
        self.dic[number] = max(left_v,right_v)
        return self.dic[number]

    def recursive(self,node,number,limit):
        if node.left != None :
            if self.dic[number*2] >= limit:
                self.recursive(node.left,number*2,limit)
            else:
                node.left = None
        if node.right != None :
            if self.dic[number*2+1] >= limit:
                self.recursive(node.right,number*2+1, limit)
            else:
                node.right = None

    def sufficientSubset(self, root, limit):
        """
        :type root: TreeNode
        :type limit: int
        :rtype: TreeNode
        """
        self.dic = {}
        self.calculate(root,1,0)
        #print self.dic
        if self.dic[1] < limit:
            return None
        self.recursive(root,1,limit)
        return root