软工作业3:结对编程【四则运算】
学生个人信息
| 姓名 | 学号 |
|---|---|
| 郑耿杭 | 3121004978 |
| 梁鸿俊 | 3121004956 |
作业基本信息
| 这个作业属于哪个课程 | 软件工程 |
|---|---|
| 作业要求 | 结对编程 |
| 作业目标 | 实现一个自动生成小学四则运算的程序 |
Github
https://github.com/lianghongjun/operation
PSP表格
| PSP2.1 | Personal Software Process Stages | 预估耗时(分钟) | 实际耗时(分钟) |
|---|---|---|---|
| Planning | 计划 | 30 | 20 |
| Estimate | 估计这个任务需要多少时间 | 30 | 15 |
| Development | 开发 | 180 | 200 |
| Analysis | 需求分析 | 120 | 90 |
| Design Spec | 生成设计文档 | 10 | 10 |
| Design Review | 设计复审 | 10 | 10 |
| Coding Standard | 代码规范 | 5 | 5 |
| Design | 具体设计 | 50 | 60 |
| Coding | 具体编码 | 80 | 80 |
| Code Review | 代码复审 | 30 | 20 |
| Test | 测试 | 40 | 50 |
| Reporting | 报告 | 10 | 10 |
| Test Report | 测试报告 | 10 | 10 |
| Size Measurement | 计算工作量 | 20 | 20 |
| Postmortem & Process Improvement Plan | 事后总结, 并提出过程改进计划 | 10 | 10 |
| 合计 | 625 | 610 |
设计实现过程
项目目录
1.Arithmetic:算术类(子类:运算符类Operator,操作数类Operand,括号类Bracket)
2.Brackets:括号类
3.Equation:表达式类
4.Operand:操作数类
5。Operator:运算符类
6.FileUtils:文件工具类,包括将内容写入指定路径文件和从指定路径文件读取成表达式数组的方法
代码说明
1.关键代码
filter()
说明:用于过滤重复的表达式
思路:
1.按顺序层层筛选,由于转换成后缀表达式,不用考虑括号
2.先去除运算过程含负数的
3.先比较结果
4.比较表达式是否一样
5.再比较包含的运算符是否相同
6.比较第一次运算的两数是否只是交换位置
点击查看代码
/**
* 用来过滤重复表达式,按顺序层层筛选,由于转换成后缀表达式,不用考虑括号
* 1. 先去除运算过程含负数的
* 2. 先比较结果
* 3. 比较表达式是否一样
* 4. 再比较包含的运算符是否相同
* 5. 比较第一次运算的两数是否只是交换位置
*
* @param list 要过滤的表达式数组
* @return 过滤完成的表达式
*/
public static List<Equation> filter(List<Equation> list) {
for (int i = 0; i < list.size(); i++) {
Equation equation = list.get(i);
// 如果运算过程含负数,则跳过
if (equation.isOf()) {
list.remove(equation);
//remove会整体前移
i--;
continue;
}
// 和整个list比较
// 标签方便下面层层嵌套能直接goto出来
flag:
for (int o = 0; o < list.size(); o++) {
Equation toCompare = list.get(o);
// 删除后有空位,要跳过
if (toCompare == null) {
continue;
}
// 遇到自己就跳过
if (equation == toCompare) {
continue;
}
// 先比较结果
if (Math.abs(equation.getResult() - toCompare.getResult()) < 0.000001) {
// 结果相同,看是否完全一样
if (equation.equals(toCompare)) {
list.remove(equation);
// remove会整体前移
i--;
break flag;
}
// 再比较运算符
List<Arithmetic> postfix1 = equation.getPostfix();
List<Arithmetic> postfix2 = toCompare.getPostfix();
List<Operator> operators1 = equation.getOperators();
List<Operator> operators2 = toCompare.getOperators();
// 有不同运算符就保留
if (operators1.size() != operators2.size()) {
break flag;
}
for (int j = 0; j < operators1.size(); j++) {
if (operators1.get(j) != operators2.get(j)) {
break flag;
}
}
// 运算符相同,只比较第一次计算的两数字是否交换位置
// 找到第一个运算符,取前两个数字
List<Operand> operands1 = new ArrayList<>();
List<Operand> operands2 = new ArrayList<>();
for (int j = 0; j < postfix1.size(); j++) {
if (postfix1.get(j) instanceof Operator) {
operands1.add((Operand) postfix1.get(j - 1));
operands1.add((Operand) postfix1.get(j - 2));
break;
}
}
for (int j = 0; j < postfix1.size(); j++) {
if (postfix2.get(j) instanceof Operator) {
operands2.add((Operand) postfix2.get(j - 1));
operands2.add((Operand) postfix2.get(j - 2));
break;
}
}
// 比较两对数字
if ((operands1.get(0).equals(operands2.get(0)) || operands1.get(0).equals(operands2.get(1))) &&
(operands1.get(1).equals(operands2.get(0)) || operands1.get(1).equals(operands2.get(1)))) {
list.remove(equation);
//remove会整体前移
i--;
}
// 两对数字不相同,保留
break;
} else {
//结果不一样,保留
break;
}
}
}
return list.stream().toList();
}
generate()
说明:用于生成随机表达式
思路:通过传参确定此次生成中包含的操作数数量、运算符数量、括号数量、数的范围,然后随机new出各对象,交替拼接操作数和运算符,最后随机添加括号
点击查看代码
/**
* 用来生成随机表达式
*
* @param operandNo 操作数数量
* @param operatorNo 运算符数量
* @param bracketsNo 括号数量
* @param lowEnd 生成表达式中操作数和真分数分母范围的下限
* @param upEnd 生成表达式中操作数和真分数分母范围的上限
* @return 生成的表达式
*/
public static Equation generate(int operandNo, int operatorNo, int bracketsNo
, int lowEnd, int upEnd) {
Random r = new Random();
int scope = upEnd - lowEnd;
List<Arithmetic> arithmetics = new ArrayList<>();
List<Operand> operands = new ArrayList<>();
List<Operator> operators = new ArrayList<>();
List<Brackets> brackets = new ArrayList<>();
try {
for (int i = 0; i < operandNo; i++) {
// 操作数类型 自然数(0),真分数(1)
int type = r.nextInt(10) % 2;
if (0 == type) {
// 生成随机整数
operands.add(new Operand(type, r.nextInt(scope) + lowEnd + ""));
} else {
// 生成真分数
int denominator = r.nextInt(scope) + lowEnd + 1;
// 分子 > 0
int numerator = r.nextInt(denominator - 1) + 1;
String str = numerator + "/" + denominator;
operands.add(new Operand(type, str));
}
}
for (int i = 0; i < operatorNo; i++) {
// 除去等号
int index = r.nextInt(4) + 1;
operators.add(Operator.getByIndex(index));
}
for (int i = 0; i < bracketsNo; i++) {
brackets.add(Brackets.getByIndex(0));
brackets.add(Brackets.getByIndex(1));
}
for (int i = 0; i < operands.size(); i++) {
if (operands.get(i) != null) {
arithmetics.add(operands.get(i));
}
if (i == operands.size() - 1) {
break;
}
if (operators.get(i) != null) {
arithmetics.add(operators.get(i));
}
}
} catch (Exception e) {
e.printStackTrace();
return null;
}
return new Equation(arithmetics);
}
infixToPostfix()
说明: 将中缀表达式转换成后缀表达式
思路:
无括号:
1.扫描中缀表达式的每一个字符,将数字入列;
2.遇到运算符,栈空时直接进栈,栈顶非空时,运算符优先级大于栈顶元素才进栈,
否则栈顶元素退栈入列,当前运算符再进栈;
3.依次进行直至所有字符操作完毕
有括号:
1.扫描中缀表达式的每一个字符,将数字入列;
2.遇到运算符,栈空时直接进栈,栈顶非空时,运算符优先级大于栈顶元素才进栈,
否则栈顶元素退栈入列,当前运算符再进栈;
3.遇到左括号,直接进栈,左括号后面的运算符直接进栈,直至遇到右括号;
4.遇到右括号时,将栈顶元素依次退栈入列,直到遇到左括号,将左括号退栈,符号操作移动下一位
5.重复以上操作,直至所有字符操作完成。
点击查看代码
/**
* 将中缀表达式转换为后缀表达式
*
* @return 返回转换结果
*/
public List<Arithmetic> infixToPostfix() {
Stack<Arithmetic> stack = new Stack<>();
List<Arithmetic> postfix = new ArrayList<>();
for (int start = 0; start < infix.size(); start++) {
// 如果是运算符
if (infix.get(start).priority > 0) {
// 栈空 或 "(" 或 符号优先级>栈顶符号 且 不为")" 直接进栈
if (stack.isEmpty() || infix.get(start).priority == 3 ||
((infix.get(start).priority > stack.peek().priority) && infix.get(start).priority < 4)) {
stack.push(infix.get(start));
} else if (!stack.isEmpty() && infix.get(start).priority <= stack.peek().priority) {
// 栈非空 且 符号优先级≤栈顶符号, 出栈; 直到 栈为空 或 遇到了"("
while (!stack.isEmpty() && infix.get(start).priority <= stack.peek().priority) {
if (stack.peek().priority == 3) {
stack.pop();
break;
}
postfix.add(stack.pop());
}
stack.push(infix.get(start));
} else if (infix.get(start).priority == 4) {
// ")",依次出栈直到空栈或遇到第一个"(",此时"("出栈
while (!stack.isEmpty()) {
if (stack.peek().priority == 3) {
stack.pop();
break;
}
postfix.add(stack.pop());
}
}
} else if (infix.get(start).priority == -1) {
postfix.add(infix.get(start));
}
}
while (!stack.isEmpty()) {
postfix.add(stack.pop());
}
return postfix;
}
测试结果
代码随机生成10000条计算式
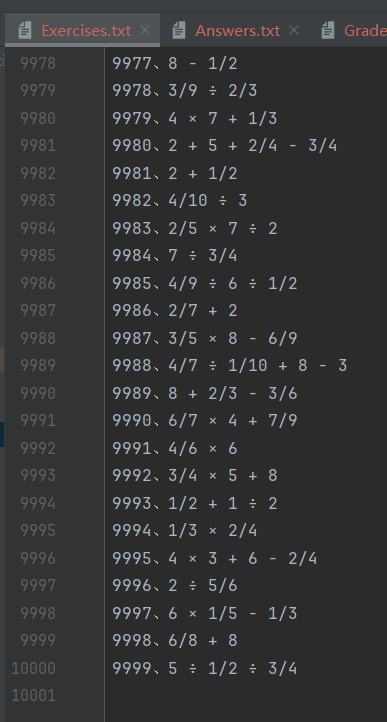
代码随机生成10000条计算式结果

代码随机生成计算式
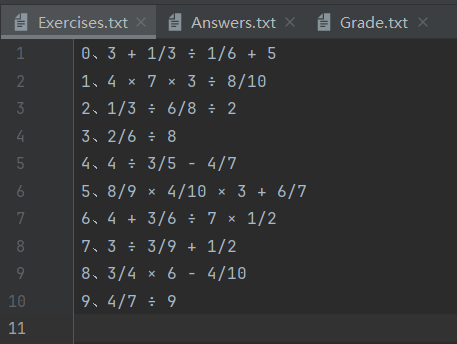
计算式计算结果
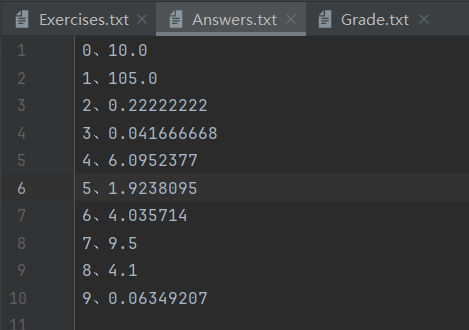
计算结果统计
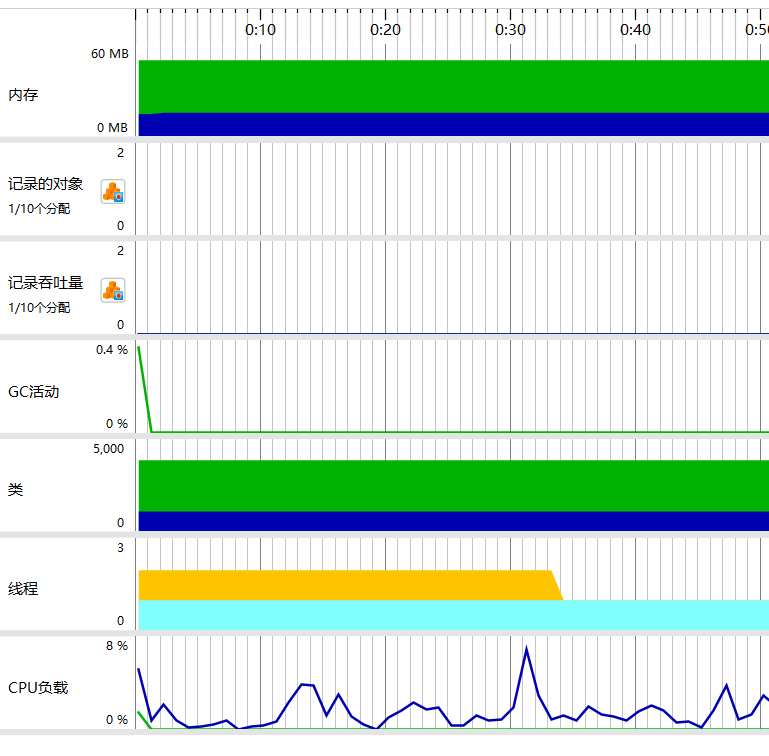
效能分析
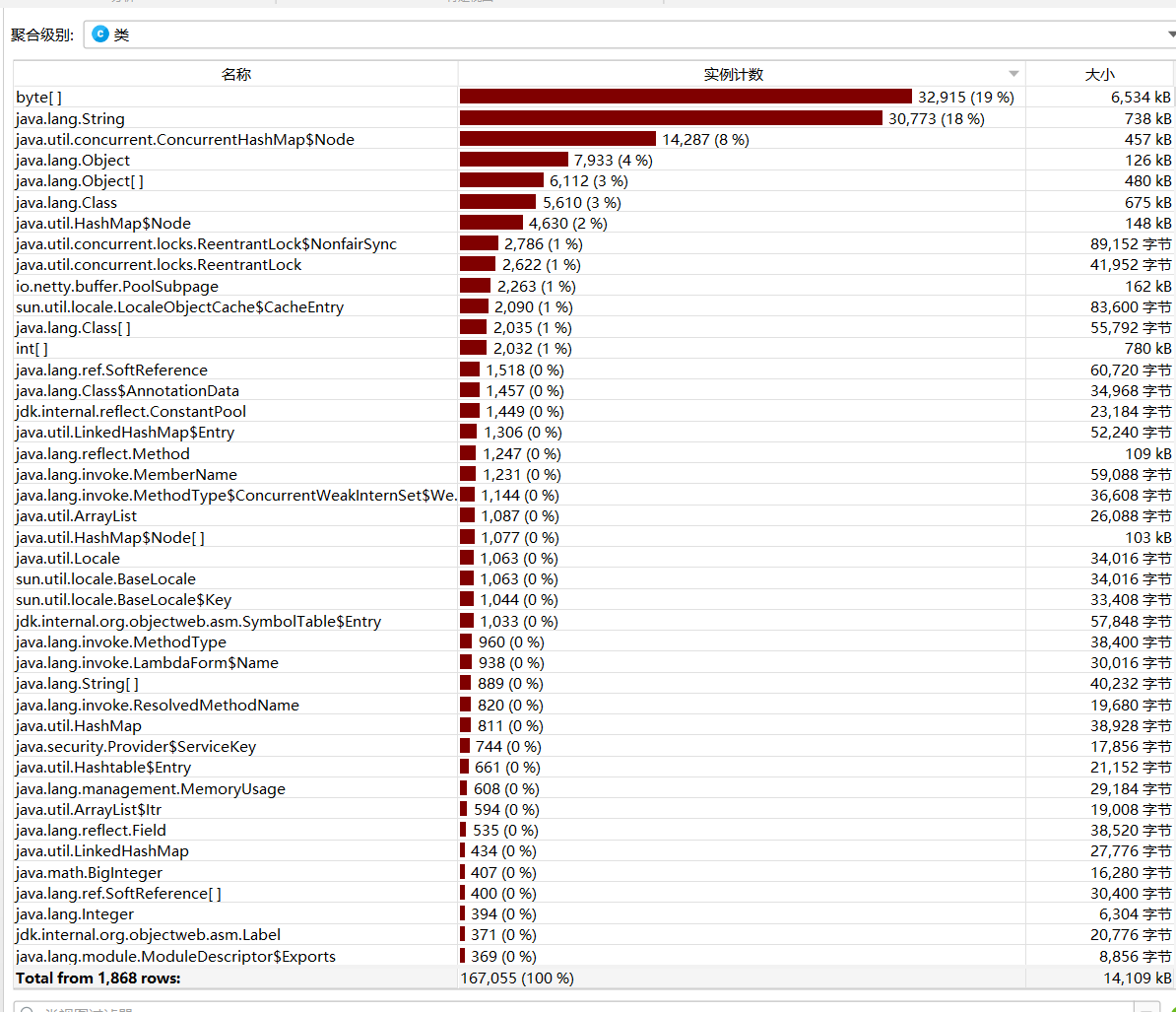
消耗最大的函数
用于去除重复的函数:Equation.filter()
因为里面用了许多逻辑判断和嵌套循环,时间复杂度高,随生成数量提高而呈幂次增长


 浙公网安备 33010602011771号
浙公网安备 33010602011771号