Codeforces Round #339 Div.2 C - Peter and Snow Blower
Peter got a new snow blower as a New Year present. Of course, Peter decided to try it immediately. After reading the instructions he realized that it does not work like regular snow blowing machines. In order to make it work, you need to tie it to some point that it does not cover, and then switch it on. As a result it will go along a circle around this point and will remove all the snow from its path.
Formally, we assume that Peter's machine is a polygon on a plane. Then, after the machine is switched on, it will make a circle around the point to which Peter tied it (this point lies strictly outside the polygon). That is, each of the points lying within or on the border of the polygon will move along the circular trajectory, with the center of the circle at the point to which Peter tied his machine.
Peter decided to tie his car to point P and now he is wondering what is the area of the region that will be cleared from snow. Help him.
The first line of the input contains three integers — the number of vertices of the polygon n ( ), and coordinates of point P.
), and coordinates of point P.
Each of the next n lines contains two integers — coordinates of the vertices of the polygon in the clockwise or counterclockwise order. It is guaranteed that no three consecutive vertices lie on a common straight line.
All the numbers in the input are integers that do not exceed 1 000 000 in their absolute value.
Print a single real value number — the area of the region that will be cleared. Your answer will be considered correct if its absolute or relative error does not exceed 10 - 6.
Namely: let's assume that your answer is a, and the answer of the jury is b. The checker program will consider your answer correct, if 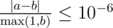 .
.
3 0 0
0 1
-1 2
1 2
12.566370614359172464
4 1 -1
0 0
1 2
2 0
1 1
21.991148575128551812
几何题做的不多呢 这次算是对自己的检验
题意很简单 就是找多边形上距离旋转中心的最远点和最近点 然后大圆面积减小圆面积
最远点必定在点上 最近点则可能在边上
更新最近点时 取相邻两点和旋转中心构成三角形 用余弦定理判断这两个点对应的角是否钝角 换句话说最近点是否在相邻两点间
如果有钝角 直接取两点到中心距离近的那个 如果没有 用海伦公式算高
#include <iostream> #include <string> #include <cstdio> #include <cmath> #include <cstring> #include <queue> #include <map> #include <vector> #include <set> #include <algorithm> #define INF 0x3F3F3F3F #define PI 3.1415926535898 using namespace std; struct Node{ double x, y; }node[100010]; double DISTANCE(int a, int b){ return sqrt((node[a].x - node[b].x) * (node[a].x - node[b].x) + (node[a].y - node[b].y) * (node[a].y - node[b].y)); } int main() { int n; double up, down, s; scanf("%d%lf%lf", &n, &node[0].x, &node[0].y); up = 0; down = 1e20; for(int i = 1; i <= n; i++){ scanf("%lf%lf", &node[i].x, &node[i].y); up = max(DISTANCE(i, 0), up); } for(int i = 1; i < n; i++){ double a = DISTANCE(0, i); double b = DISTANCE(0, i + 1); double c = DISTANCE(i, i + 1); if(a*a + c*c - b*b < 0 || b*b + c*c - a*a < 0){ down = min(down, min(a, b)); } else{ double p = (a + b + c) / 2; down = min(down, 2 * sqrt(p * (p - a) * (p - b) * (p - c)) / c); } } { double a = DISTANCE(0, 1); double b = DISTANCE(0, n); double c = DISTANCE(1, n); if(a*a + c*c - b*b < 0 || b*b + c*c - a*a < 0){ down = min(down, min(a, b)); } else{ double p = (a + b + c) / 2; down = min(down, 2 * sqrt(p * (p - a) * (p - b) * (p - c)) / c); } } s = ((up * up) - (down * down)) * PI; printf("%.16lf\n", s); //printf("up = %lf down = %lf\n", up, down); return 0; }




【推荐】编程新体验,更懂你的AI,立即体验豆包MarsCode编程助手
【推荐】凌霞软件回馈社区,博客园 & 1Panel & Halo 联合会员上线
【推荐】抖音旗下AI助手豆包,你的智能百科全书,全免费不限次数
【推荐】博客园社区专享云产品让利特惠,阿里云新客6.5折上折
【推荐】轻量又高性能的 SSH 工具 IShell:AI 加持,快人一步