Lorenz方程组的MATLAB实现
首先,我们考虑Lorenz方程组
dy1/dt=sigma*(y2-y1),
dy2/dt=r*y1-y2-y1*y3,
dy3/dt=y1*y2-b*y3,
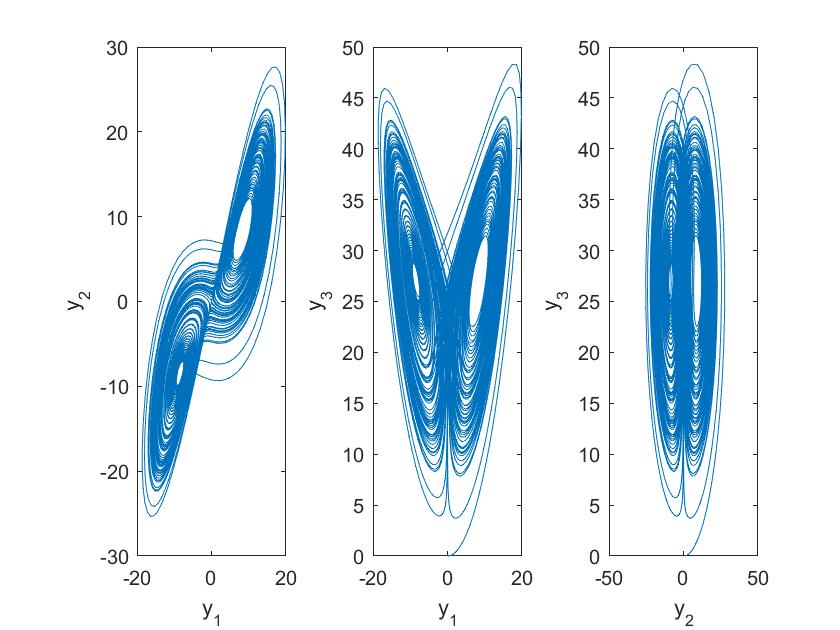
其中 sigma为普朗特数,r为瑞利数,b为非负常数,这是一个混沌系统。当我们取参数 sigma=10,r=28,sigma=8/3,初值为(0,1,0)时,采用四阶显式龙格库塔方法进行求解的MATLAB程序如下:
主程序:
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 | clc,clear;close all;k = 0.01; % constant step sizetf = 100; % final time% given initial conditionsy0(1) = 0; y0(2) = 1; y0(3) = 0;y0 = shiftdim(y0);% integrate[tout,yout] = rk4(tf,k,y0);% plot solution in phase spacefigure (1)subplot(1,3,1)plot(yout(1,:),yout(2,:));xlabel('y_1');ylabel('y_2');subplot(1,3,2)plot(yout(1,:),yout(3,:));xlabel('y_1');ylabel('y_3');subplot(1,3,3)plot(yout(2,:),yout(3,:));xlabel('y_2');ylabel('y_3');figure (2)subplot(1,3,1)plot(tout,yout(1,:));xlabel('t');ylabel('y_1');subplot(1,3,2)plot(tout,yout(2,:));xlabel('t');ylabel('y_2');subplot(1,3,3)plot(tout,yout(3,:));xlabel('t');ylabel('y_3'); |
子程序:显式龙格库塔法
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 | function [tout,yout] = rk4 (tf,k,y0)%% [tout,yout] = rk4 (tf,k,y0)% solves non-stiff IVODEs based on classical 4-stage Runge-Kutta%% y’ = ffun (t,y), y(0) = y0, 0 < t < tf% ffun returns a vector of m components for a given% t and y of m components.%% Input:% tf - final time% k - uniform step size% y0 - initial values y(0) (size (m,1))%% Output:% tout - times where solution is computed% yout - solution vector at times tout.% prepareN = tf / k;tout = [0];fo = ffun (tout(1),y0);yout(:,1) = y0;% loop in timefor n=1:Nt = tout(n);y = yout(:,n);Y1 = y;K1 = ffun(t,Y1);Y2 = y + k/2*K1;t = t + k/2;K2 = ffun(t,Y2);Y3 = y + k/2*K2;K3 = ffun(t,Y3);Y4 = y + k*K3;t = t + k/2;K4 = ffun(t,Y4);tout(n+1) = t;yout(:,n+1) = y + k/6*(K1+2*K2+2*K3+K4);endend |
子程序:求解函数
1 2 3 4 5 6 7 | function f = ffun(t,y)% function defining ODEsigma = 10; b = 8/3; r = 28;f = zeros(3,1);f(1) = sigma *(y(2)-y(1));f(2) = r * y(1) - y(2) - y(1)*y(3);f(3) = y(1)*y(2) - b*y(3); |
结果:

参考文献:
[1] Ascher, Uri M . Numerical Methods for Evolutionary Differential Equations, SIAM, 2008.




【推荐】编程新体验,更懂你的AI,立即体验豆包MarsCode编程助手
【推荐】凌霞软件回馈社区,博客园 & 1Panel & Halo 联合会员上线
【推荐】抖音旗下AI助手豆包,你的智能百科全书,全免费不限次数
【推荐】博客园社区专享云产品让利特惠,阿里云新客6.5折上折
【推荐】轻量又高性能的 SSH 工具 IShell:AI 加持,快人一步
· Java 中堆内存和栈内存上的数据分布和特点
· 开发中对象命名的一点思考
· .NET Core内存结构体系(Windows环境)底层原理浅谈
· C# 深度学习:对抗生成网络(GAN)训练头像生成模型
· .NET 适配 HarmonyOS 进展
· 手把手教你更优雅的享受 DeepSeek
· 腾讯元宝接入 DeepSeek R1 模型,支持深度思考 + 联网搜索,好用不卡机!
· AI工具推荐:领先的开源 AI 代码助手——Continue
· 探秘Transformer系列之(2)---总体架构
· V-Control:一个基于 .NET MAUI 的开箱即用的UI组件库