Data Structure and Algorithm - Day 05

• List: one next

  Tree: multi next, no cycle

  Graph: has cycle

• Binary Search Tree

  left children < root < right children (children! not child!)

  Time Complexity: lookup O(log n), insert O(log n)

• 94. Binary Tree Inorder Traversal

  Given the root of a binary tree, return the inorder traversal of its nodes' values.

  Example 1:

  

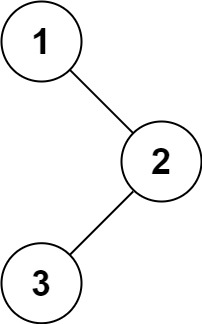
  Input: root = [1,null,2,3]
  Output: [1,3,2]
  

  Example 2:

  Input: root = []
  Output: []
  

  Example 3:

  Input: root = [1]
  Output: [1]
  

  Example 4:

  

  Input: root = [1,2]
  Output: [2,1]
  

  Example 5:

  

  Input: root = [1,null,2]
  Output: [1,2]
  

  Constraints:

  • The number of nodes in the tree is in the range [0, 100].
  • -100 <= Node.val <= 100

  class Solution {
      public List<Integer> inorderTraversal(TreeNode root) {
          List<Integer> res = new ArrayList<>();
          helper(res, root);
          return res;
      }
  
      private void helper(List<Integer> list, TreeNode root) {
          if (root == null) return ;
          helper(list, root.left);
          list.add(root.val);
          helper(list, root.right);
      }
  }
  

• 144. Binary Tree Preorder Traversal

  Given the root of a binary tree, return the preorder traversal of its nodes' values.

  Example 1:

  

  Input: root = [1,null,2,3]
  Output: [1,2,3]
  

  Example 2:

  Input: root = []
  Output: []
  

  Example 3:

  Input: root = [1]
  Output: [1]
  

  Example 4:

  

  Input: root = [1,2]
  Output: [1,2]
  

  Example 5:

  

  Input: root = [1,null,2]
  Output: [1,2]
  

  Constraints:

  • The number of nodes in the tree is in the range [0, 100].
  • -100 <= Node.val <= 100

  class Solution {
      public List<Integer> preorderTraversal(TreeNode root) {
          List<Integer> res = new ArrayList<>();
          helper(res, root);
          return res;
      }
  
      private void helper(List<Integer> list, TreeNode root) {
          if (root == null) return ;
          list.add(root.val);
          helper(list, root.left);
          helper(list, root.right);
      }
  }
  

• 590. N-ary Tree Postorder Traversal

  Given the root of an n-ary tree, return the postorder traversal of its nodes' values.

  Nary-Tree input serialization is represented in their level order traversal. Each group of children is separated by the null value (See examples)

  Example 1:

  

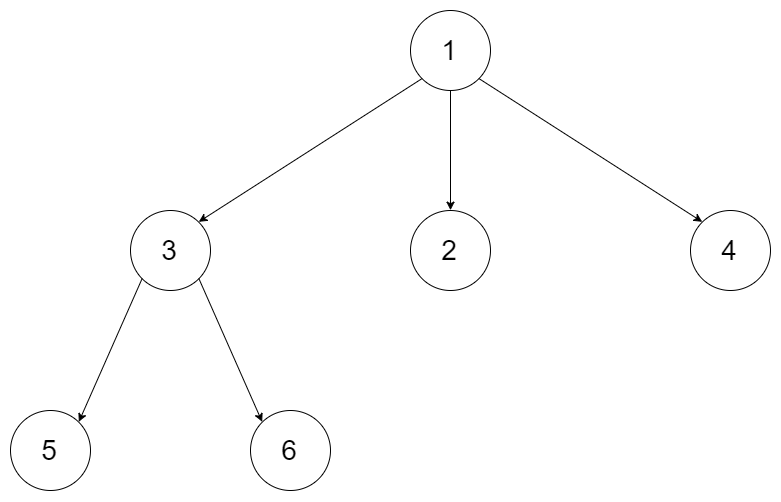
  Input: root = [1,null,3,2,4,null,5,6]
  Output: [5,6,3,2,4,1]
  

  Example 2:

  

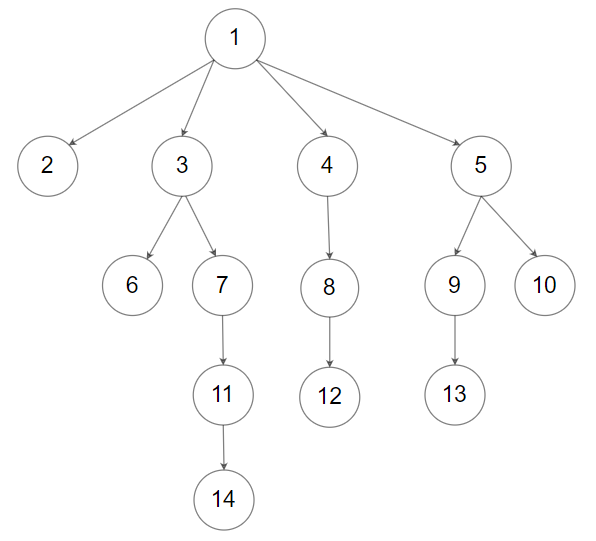
  Input: root = [1,null,2,3,4,5,null,null,6,7,null,8,null,9,10,null,null,11,null,12,null,13,null,null,14]
  Output: [2,6,14,11,7,3,12,8,4,13,9,10,5,1]
  

  Constraints:

  • The number of nodes in the tree is in the range [0, 104].
  • 0 <= Node.val <= 104
  • The height of the n-ary tree is less than or equal to 1000.

  class Solution {
     public List<Integer> postorder(Node root) {
         List<Integer> res = new ArrayList<>();
         helper(res, root);
         return res;
     }
  
     private void helper(List<Integer> list, Node root) {
         if (root == null) return ;
         for (Node child : root.children) {
             helper(list, child);
         }
         list.add(root.val);
     }
  }
  

• 589. N-ary Tree Preorder Traversal

  Given the root of an n-ary tree, return the preorder traversal of its nodes' values.

  Nary-Tree input serialization is represented in their level order traversal. Each group of children is separated by the null value (See examples)

  Example 1:

  

  Input: root = [1,null,3,2,4,null,5,6]
  Output: [1,3,5,6,2,4]
  

  Example 2:

  

  Input: root = [1,null,2,3,4,5,null,null,6,7,null,8,null,9,10,null,null,11,null,12,null,13,null,null,14]
  Output: [1,2,3,6,7,11,14,4,8,12,5,9,13,10]
  

  Constraints:

  • The number of nodes in the tree is in the range [0, 104].
  • 0 <= Node.val <= 104
  • The height of the n-ary tree is less than or equal to 1000.

  class Solution {
      public List<Integer> preorder(Node root) {
          List<Integer> res = new ArrayList<>();
          helper(root, res);
          return res;
      }
  
      private void helper(Node root, List<Integer> list) {
          if (root == null) return ;
          list.add(root.val);
          for (Node child : root.children) {
              helper(child, list);
          }
      }
  }
  

• 429. N-ary Tree Level Order Traversal

  Given an n-ary tree, return the level order traversal of its nodes' values.

  Nary-Tree input serialization is represented in their level order traversal, each group of children is separated by the null value (See examples).

  Example 1:

  

  Input: root = [1,null,3,2,4,null,5,6]
  Output: [[1],[3,2,4],[5,6]]
  

  Example 2:

  

  Input: root = [1,null,2,3,4,5,null,null,6,7,null,8,null,9,10,null,null,11,null,12,null,13,null,null,14]
  Output: [[1],[2,3,4,5],[6,7,8,9,10],[11,12,13],[14]]
  

  Constraints:

  • The height of the n-ary tree is less than or equal to 1000
  • The total number of nodes is between [0, 104]

  class Solution {
      public List<List<Integer>> levelOrder(Node root) {
          List<List<Integer>> res = new ArrayList<>();
          if (root == null) return res;
          Deque<Node> queue = new LinkedList<>();
          queue.addLast(root);
          while (!queue.isEmpty()) {
              List<Integer> list = new ArrayList<>();
              int size = queue.size();
              while (size-- > 0) {
                  Node node = queue.pollFirst();
                  list.add(node.val);
                  for (Node child : node.children) {
                      queue.addLast(child);
                  }
              }
              res.add(list);
          }
          return res;
      }
  }
  

• 239. Sliding Window Maximum

  You are given an array of integers nums, there is a sliding window of size k which is moving from the very left of the array to the very right. You can only see the k numbers in the window. Each time the sliding window moves right by one position.

  Return the max sliding window.

  Example 1:

  Input: nums = [1,3,-1,-3,5,3,6,7], k = 3
  Output: [3,3,5,5,6,7]
  Explanation: 
  Window position                Max
  ---------------               -----
  [1  3  -1] -3  5  3  6  7       3
   1 [3  -1  -3] 5  3  6  7       3
   1  3 [-1  -3  5] 3  6  7       5
   1  3  -1 [-3  5  3] 6  7       5
   1  3  -1  -3 [5  3  6] 7       6
   1  3  -1  -3  5 [3  6  7]      7
  

  Example 2:

  Input: nums = [1], k = 1
  Output: [1]
  

  Example 3:

  Input: nums = [1,-1], k = 1
  Output: [1,-1]
  

  Example 4:

  Input: nums = [9,11], k = 2
  Output: [11]
  

  Example 5:

  Input: nums = [4,-2], k = 2
  Output: [4]
  

  Constraints:

  • 1 <= nums.length <= 105
  • -104 <= nums[i] <= 104
  • 1 <= k <= nums.length

  class Solution {
      public int[] maxSlidingWindow(int[] nums, int k) {
          int len = nums.length;
          int[] res = new int[len - k + 1];
          Deque<Integer> deque = new LinkedList<>();
          for (int i = 0; i < k; i++) {
              while (!deque.isEmpty() && nums[deque.peekLast()] < nums[i]) {
                  deque.pollLast();
              }
              deque.addLast(i);
          }
          for (int i = k; i < len; i++) {
              int front = deque.peekFirst();
              res[i - k] = nums[front];
              if (front + k <= i) {
                  deque.pollFirst();
              }
              while (!deque.isEmpty() && nums[deque.peekLast()] < nums[i]) {
                  deque.pollLast();
              }
              deque.addLast(i);
          }
          res[len - k] = nums[deque.peekFirst()];
          return res;
      }
  }