Houdini Vellum 之 Graph Color 的算法分析
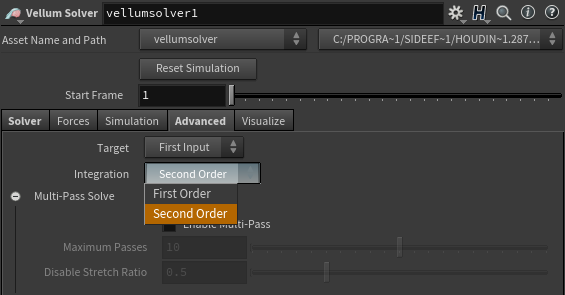
Houdini 18 的Vellum目前使用的是更高级的PBD: XPBD(eXtended Position Based Dynamics) 。XPBD使用了2nd Order Integration ,传统的PBD使用的1nd Order Intergration 。想要查看的话,如下图(默认是2nd Order Integration)。
Vellum Solver中因为要对约束重复的运算(Constraint Iterations 设置迭代的次数),需要比较高的效率。 为了能够让并行运算,引入了Graph Corlor技术。Graph Color说白了, 就是定义一个属性,每个约束的属性和与它相邻的约束的属性值不同。
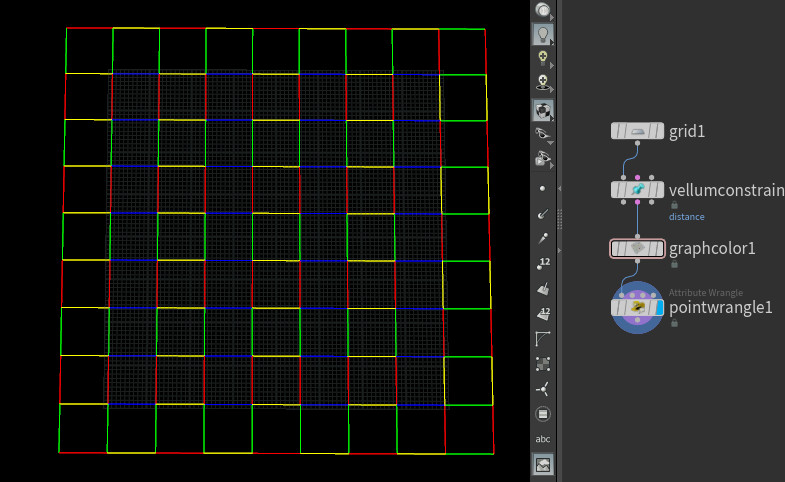
比如用一个Grid,用Vellum Contraints 得到 distant contrainsts ,然后用Graph Color节点进行计算 (结果默认写入在一个整型color属性上),最后用wrangle 显示颜色(相同的color属性颜色相同), 如下图,能够看到,每一条边(primitive)都与相邻的边的颜色不同。 这样让颜色相同的contrainst在同一个Workset。 不同颜色,不同的Workset。这样在对constrait进行迭代计算时(是在opencl里面进行的),Opencl会根据Workset,同一个Workset才会一起平行计算。
当然,Vellum 中Graph Color的计算时在Vellum Solver内部,这里只是为了演示方便。
另个案例了解Vellum Solver 中 的 Graph Color
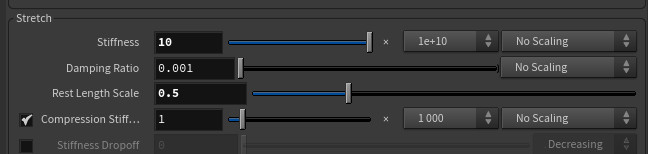
新建个一个Grid,选中两边的点, 给Pin Constraints,把两边定住。加 distant constraints ( Vellum Constraint 选 Distant along edges),设置如下图。注意Stiffness设置为最大(10*1e+10),这种材质现实中是没有弹性的, 但是我们又把Rest Length Scale设置为0.5,Vellum Solver会迫使它压缩(现实中这种材质的布料是不存在的)。然后把Vellum Solver的 Smooth Iteration 设置为0(这步很重要)。 结算。
结算后发现,有的边的长度缩短大致0.5倍,有的反而被拉长了。用Graph Color查看,发现拉伸边长的是同一个颜色,即作为同一个WorkSet在opencl中并行计算的。如下图:
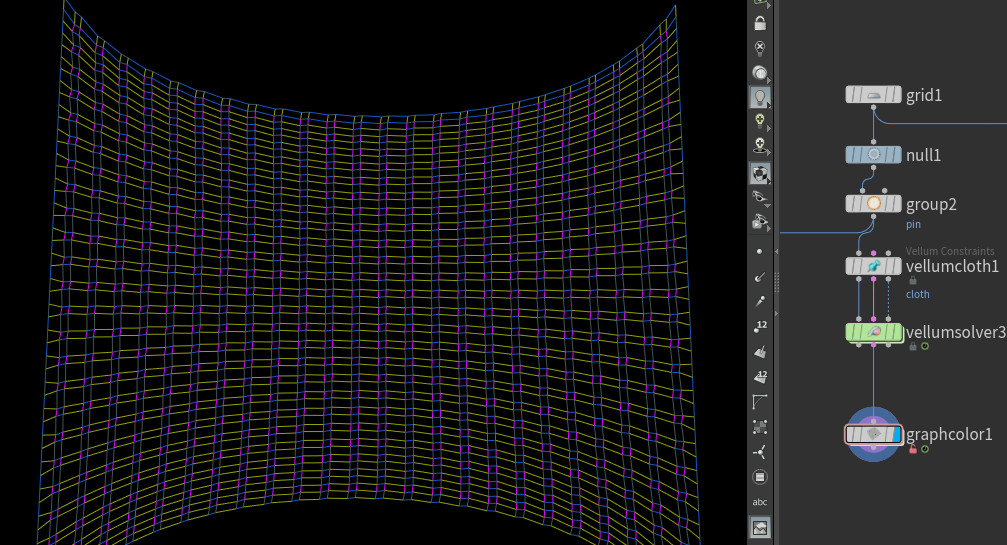
这是因为黄色的边做一个同一个Workset首先在opencl中运算,红色和蓝色在黄色后面分别进行运算,这样导致错误都转嫁到了第一次计算的边上(这里的错误是指,设置了Rest Length Scale为0.5倍,红色和蓝色都正确压缩了,黄色反而拉长了,这是错误的, 不是我们想要的)。
Graph Color 的算法分析
下面分析下Graph Color节点里面的算法。新建一个Grid,Graph Color, Color(random from attributes)。
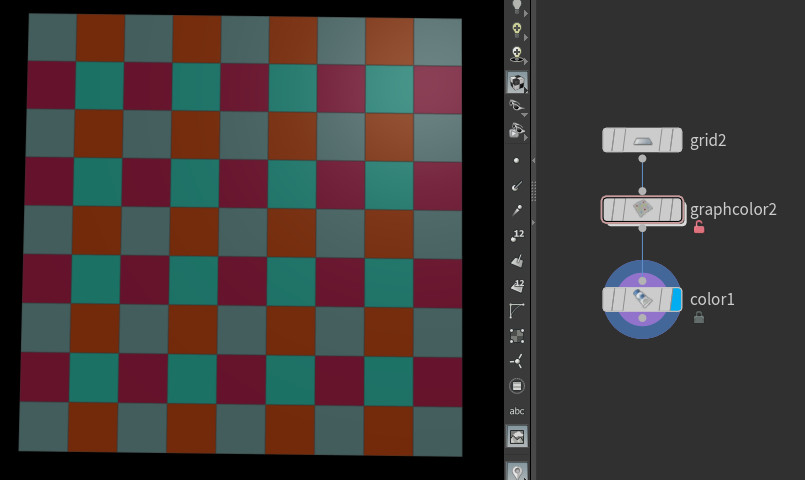
进入Graph Color内部, 主要分析最左边这路(Connectivity 为Primtive),Contraints运算的时候也是以Primtive为单位。
(1)create_color_attrib
创建 color (Integer)primitive属性
(2)find_npts
把当前primitive总共有多少邻居记录在属性 @__npts属性上。
i@__npts = len(primpoints(0, @primnum));
(3)color_high_valence (Run Over Detail )
这步主要是把邻居多的primitive筛选出来(Graph Color 的参数Max Valence设置阈值,默认20),单独计算。因为进入opencl进行并行计算时,需要把邻居primitive写入在一个array数组里,如果相邻的邻居太多,会造成存储和计算时间的极大浪费。挑其中比较重要的代码部分进行分析:
下面的代码把筛选出来的符合条件的primitve(@__npts>maxvalence) 存入在prims数组里面。
int maxvalence = chi("maxvalence"); string grp = sprintf("\@__npts>=%d", maxvalence); int prims[] = expandprimgroup(0, grp);
然后对数组进行排序。npts数组记录prims数组中对应的prim的点的数量乘以-1 。argsort对npts数组排序,它返回的是一个indice的数组。
indice: 比如一个整型数组 int a[] = {0,4,2} 。a[1] = 4 ,中括号里面的1就是indice, 也就是数组下标。
对于一个数组int b[] = {-4,-10,-2,-8} 进行 argsort排序会得到一个数组下标的数组 {1,3,0,2},最后用reorder函数得到最终的排序数组 {b[1],b[3],b[0],b[2]} 即{ -10,-8,-4,-2}。 (argsort 和reorder一般搭档起来用,来对数组排序)。
foreach(int i; int prim; prims) { npts[i] = -len(primpoints(0, prim)); } prims = reorder(prims, argsort(npts));
最终得到一个数组,根据primitive的点的数量由大到小排列。
核心的部分在下面这个foreeach部分
foreach(int i; int prim; prims) { int useNewColor = 1; // 声明新变量,是否使用新颜色,默认1,即使用新染色 int pts[] = primpoints(0, prim); for(int j = 0; j < ncolors; j++) // ncolors记录当前不同color的数量, 遍历所有color { int useColor = 1; // 声明新变量,能否用当前颜色,默认1, 可以使用 foreach(int pt; pts) // 遍历当前primitive的每个点 { if (mapping[j * npt + pt] != 0) //mapping是一个数组,npts是所有点的数量(不止当前primitive) { //比如npts= 14 ,当前primitive2个点,对于j=0,即color值为0 ,mapping[0*14+0]~mappint[0*14+(14-1)] useColor = 0; //对应的所有的点使用的color 0的情况,mapping值若为1即不等于0, 说明当前color值被邻居占用,不能使用 break; // 这是把useColor赋值0, 即当前color值不可用 } } if (useColor) //如果当前颜色可用 { colors[i] = j; // 把当前primitive 的color值记录到colors数组里 foreach(int pt; pts) // 对于当前primitive的所有点pt,color为j,对应mapping[j*npt+pt],设置为1,表示被当前primitive占用
{ mapping[j * npt + pt] = 1; // } useNewColor = 0; // 这种情况就不使用新颜色了 break; } } if (useNewColor) //说明没有可用的color,需要新增加一个color { colors[i] = ncolors; // 新的color值存到colors数组里,colors[i]是prims[i]对应的颜色值 ncolors++; // 总颜色数加1 resize(mapping, ncolors * npt); // mapping数组resize,空间比之前多了npt个 foreach(int pt; pts) { mapping[colors[i] * npt + pt] = 1; //对于新增的color[i],当前primitive的点pt, 把对应的mappint[colors[i]*npt+pt]赋值1, 表示被占用 } } }
这个节点完整的代码如下:

1 // Handle high valence prims in a serial pre-pass, 2 // else the find_neighbors wrangle below ends up creating 3 // extremely large neighbors arrays, which are expensive 4 // in memory and time. 5 int maxvalence = chi("maxvalence"); 6 string grp = sprintf("\@__npts>=%d", maxvalence); 7 int prims[] = expandprimgroup(0, grp); 8 int nprim = len(prims); 9 if (nprim == 0) 10 return; 11 12 // Create mapping for each point to each color and 13 // prims to each color. 14 int npt = npoints(0); 15 int mapping[]; 16 int colors[]; 17 resize(colors, nprim); 18 int ncolors = 0; 19 20 21 // Reverse sort by num pts in constraint 22 // so constraints with more points are first. 23 int npts[]; 24 resize(npts, nprim); 25 foreach(int i; int prim; prims) 26 { 27 npts[i] = -len(primpoints(0, prim)); 28 } 29 prims = reorder(prims, argsort(npts)); 30 31 foreach(int i; int prim; prims) 32 { 33 int useNewColor = 1; 34 int pts[] = primpoints(0, prim); 35 for(int j = 0; j < ncolors; j++) 36 { 37 // See if we can use this color. 38 int useColor = 1; 39 foreach(int pt; pts) 40 { 41 if (mapping[j * npt + pt] != 0) 42 { 43 useColor = 0; 44 break; 45 } 46 } 47 48 if (useColor) 49 { 50 colors[i] = j; 51 foreach(int pt; pts) 52 { 53 mapping[j * npt + pt] = 1; 54 } 55 useNewColor = 0; 56 break; 57 } 58 } 59 if (useNewColor) 60 { 61 colors[i] = ncolors; 62 ncolors++; 63 resize(mapping, ncolors * npt); 64 foreach(int pt; pts) 65 { 66 mapping[colors[i] * npt + pt] = 1; 67 } 68 } 69 } 70 71 // Set prim color attribute. Colored prims will be skipped 72 // by the OpenCL pass. 73 foreach(int i; int prim; prims) 74 setprimattrib(geoself(), chs("colorname"), prim, colors[i]);
(4)copy_color_to_newcolor
把color属性复制一份,新属性名:__newcolor。 这是给opencl用。
(5)find_neighbors
把所有与当前primitiv相邻的primitive记录在数组@__neighbours数组里。
int neighbors[]; int pts[] = primpoints(0, @primnum); foreach(int pt; pts) foreach(int n; pointprims(0, pt)) if (n != @primnum) append(neighbors, n); i[]@__neighbors = neighbors;
(6) create_offsets_sizes
设置opencl 的 workset ,@__sizes是所有primitive的数量,@__offsets={0}。即只有一个Workset,这个workset包含所有的primitves。
i[]@__offsets = {0};
i[]@__sizes = array(nprimitives(0));
可能会有人产生疑问,就一个workset ?这不和不设置workset一样的吗?所有的primitive都并行执行。这是因为在Opencl中,比如设置了迭代次数(Iteration)为1000,当我们迭代到500时,发现已经达到目的了,所有primitive与和它相邻的primitive 的color值都不同,那么此时可以吧@__sizes设置为0,表明workset的大小为0,不需要继续迭代计算了,这样避免了资源的浪费。
(7)assign_colors_sizes
opencl部分,为每个primitive寻找color值。使用的是Gebremedhin-Manne speculative coloring 算法。每一次迭代都分为两部分 (1)寻找color (2) 解决冲突 ,即当某个primitive与它的相邻primitive是相同的color时,需要重新寻找color
(1)寻找color
#define MAXFORBID 18446744073709551615ul 这里定义MAXFORBID为64位二进制能表示的最大值,二进制表示为 11111111 -(中间48个1)- 11111111
if (color[idx] >= 0) // 说明已经找到color了, 比如前面第三部,为邻居多的primitive提前设置了color,这里就不在重新寻找color了 return; int nidx = neighbors_index[idx]; //nidx是指针,指向与当前primitive的相邻的primitives的第一个primitive int nlast = neighbors_index[idx+1]; // nlast也是指针,但实际上它指向与global id 为idx+1的 primitive相邻的第一个primitive int c = -1, offset = 0; // 因为指针是连续存储的,nlast-1 也就指向当前primitive的最后一个primitive // Loop until colored. while(c < 0) // c 小于0说明没找到color ,继续 { // Bit flag to store seen neighbor colors. ulong forbidden = 0; // 64位(二进制)forbidden,每一位表示一个color,详见下面注释1 for(int i = nidx; i < nlast; i++) //遍历所有邻居primitive { // Flag any neighbor colors present in the current bitrange. int n = neighbors[i]; int nc = color[n] - offset; //color[n]是当前邻居的color值 if (nc >= 0 && nc < FORBIDBITS) // 详见注释2 forbidden |= (1ul << nc); //见注释2 } // Check if there's an open color in the current bitrange. if (forbidden ^ MAXFORBID) //forbidden 和 64位全1做二进制与运算 { ulong x = forbidden; //forbidden值赋值给x c = offset; // Find position of first zero bit. x = (~x) & (x + 1); // 见注释3, 找最小的color // Color is log2(x) while(x > 1) //说明找到颜色 { x /= 2; //为方便假设x为八位二进制0000 0100 ,表示成10进制数为22 ,这里要循环2次,最终的得到c值为2 c++; } } else { // Otherwise we need to try again with the next range of colors. offset += FORBIDBITS; // 没找到,说明64位不够用,再加64位 } } // Record speculative coloring. newcolor[idx] = c;
注释1:
为了表示方便, 我们假设forbidden是八位的二进制。那么forbidden值为0000 0101 表示第0个color(最右边1)和第二个color 已经被占用。
注释2:
color[n]是当前邻居的color值,假设为3 。假设我们64个color值够用,那么此时对应offset = 0。为了方便,假设forbidden值为00000000 00000000 00000000 00000000 00000000 00000000 00000000 00010101。
nc = color[n] - offset = 3
1ul << nc :这是二进制左进位, 1ul 值为 00000000 00000000 00000000 00000000 00000000 00000000 00000000 00000001,1ul<<3, 即相左进三位:
00000000 00000000 00000000 00000000 00000000 00000000 00000000 00001000
forbidden |= (1ul << nc): 二进制或运算
forbidden : 00000000 00000000 00000000 00000000 00000000 00000000 00000000 00010101
(1ul << nc) : 00000000 00000000 00000000 00000000 00000000 00000000 00000000 00001000
或运算结果: 00000000 00000000 00000000 00000000 00000000 00000000 00000000 00011101
把或运算的结果复制给forbidden ,表示第0个,第二个,第三个,第四个 color已经被邻居占用了,不能使用了。forbidden中存在0,表示还有可以使用的color
注释3:
这步利用了二进制运算的一个性质。能很快的找到最小的color。
比如当前 x 值 为 00000000 00000000 00000000 00000000 00000000 00000000 00000000 00011101
~x 即二进制取反 : 11111111 11111111 11111111 11111111 11111111 11111111 11111111 11100010
(x + 1)即二进制加1: 00000000 00000000 00000000 00000000 00000000 00000000 00000000 00011110
(~x) & (x + 1) 二进制与运算: 00000000 00000000 00000000 00000000 00000000 00000000 00000000 00000010
二进制与运算的结果表示第1个color可以使用(从右往左数,最右边是第0个)
(2)解决冲突
有部分是与(1)相同的,挑重点分析一下。
if (nc == c) // 如果当前primitive与邻居color相同 { int nnpt = npts[n]; if (nnpt > npt || (nnpt == npt && n < idx)) // 设定去改点数少的primitive,或者 idx大的primitive。 { conflict = 1; // 那么如果邻居的primitive包含的点数比当前的primitive多,或者点数一样多,但是当前primitive break; // 的idx大, 那设定当前primitive的conflicit为1 ,改当前primitive的color值。 } }
完整的代码如下:

1 #define FORBIDBITS 64 2 #define MAXFORBID 18446744073709551615ul 3 4 // Gebremedhin-Manne speculative coloring. 5 kernel void assigncolors( 6 int worksets_begin, 7 int worksets_length, 8 int neighbors_length, 9 global int * neighbors_index, 10 global int * neighbors , 11 int npts_length, 12 global int * npts , 13 int color_length, 14 global int * color , 15 int newcolor_length, 16 global int * newcolor, 17 int sizes_length, 18 global int * sizes_index, 19 global int * sizes 20 ) 21 { 22 int idx = get_global_id(0); 23 if (idx >= worksets_length) 24 return; 25 26 // Set sizes to zero, resolveConflicts will reset 27 // if there's more work to do. 28 if (idx == 0) 29 *sizes = 0; 30 31 // Nothing to do if already colored. 32 if (color[idx] >= 0) 33 return; 34 35 int nidx = neighbors_index[idx]; 36 int nlast = neighbors_index[idx+1]; 37 int c = -1, offset = 0; 38 // Loop until colored. 39 while(c < 0) 40 { 41 // Bit flag to store seen neighbor colors. 42 ulong forbidden = 0; 43 for(int i = nidx; i < nlast; i++) 44 { 45 // Flag any neighbor colors present in the current bitrange. 46 int n = neighbors[i]; 47 int nc = color[n] - offset; 48 if (nc >= 0 && nc < FORBIDBITS) 49 forbidden |= (1ul << nc); 50 } 51 // Check if there's an open color in the current bitrange. 52 if (forbidden ^ MAXFORBID) 53 { 54 ulong x = forbidden; 55 c = offset; 56 // Find position of first zero bit. 57 x = (~x) & (x + 1); 58 // Color is log2(x) 59 while(x > 1) 60 { 61 x /= 2; 62 c++; 63 } 64 } 65 else 66 { 67 // Otherwise we need to try again with the next range of colors. 68 offset += FORBIDBITS; 69 } 70 } 71 // Record speculative coloring. 72 newcolor[idx] = c; 73 } 74 75 kernel void resolveconflicts( 76 int worksets_begin, 77 int worksets_length, 78 int neighbors_length, 79 global int * neighbors_index, 80 global int * neighbors , 81 int npts_length, 82 global int * npts , 83 int color_length, 84 global int * color , 85 int newcolor_length, 86 global int * newcolor, 87 int sizes_length, 88 global int * sizes_index, 89 global int * sizes 90 ) 91 { 92 int idx = get_global_id(0); 93 if (idx >= worksets_length) 94 return; 95 // Nothing to do if already colored. 96 if (color[idx] >= 0) 97 return; 98 99 int nidx = neighbors_index[idx]; 100 int nlast = neighbors_index[idx+1]; 101 // Load speculative color. 102 int c = newcolor[idx]; 103 int conflict = 0; 104 int npt = npts[idx]; 105 for(int i = nidx; i < nlast; i++) 106 { 107 int n = neighbors[i]; 108 // Load neighbor's speculative color, which is also 109 // its final color if already accepted. 110 int nc = newcolor[n]; 111 112 // Check for conflict. 113 if (nc == c) 114 { 115 int nnpt = npts[n]; 116 // Resolution gives preference to primitives with more points, 117 // otherwise the one that comes first. 118 // (NOTE: We color in fewer iterations if we prioritize by number 119 // of graph neighbors, but then assuming we process prims by color, 120 // we're usually farther away from original point order leading to many 121 // cache misses and slower downstream processing.) 122 if (nnpt > npt || 123 (nnpt == npt && n < idx)) 124 { 125 conflict = 1; 126 break; 127 } 128 } 129 } 130 131 // If there's a conflict then reset sizes for more work, 132 // otherewise accept speculative color. 133 if (conflict) 134 *sizes = worksets_length; 135 else 136 color[idx] = c; 137 }
(7)sort_prims_by_color
根据颜色为primnum排序,为了下面的分workset, 做准备 (因此注意,vellum中Contraint primnum有可能会是要重新排序过的)
(8)create_workset_attrs
这步比较简单,根据生成两个array数组, @__size ,@__offset。 @__size记录每个color数值的primitive数量,每个color一个workset,@__offset记录每个workset的起始位置。

int @__offsets[]; int @__sizes[]; int offset = 0; //int @coloridx[]; string colorname = chs("colorname"); int ncolors = nuniqueval(0, "primitive", colorname); resize(@__offsets, ncolors); resize(@__sizes, ncolors); for(int i=0; i < ncolors; i++) { int c = uniqueval(0, "primitive", colorname, i); int n = findattribvalcount(0, "primitive", colorname, c); @__offsets[i] = offset; @__sizes[i] = n; // append(@coloridx, expandprimgroup(0, sprintf("\@color=%d", c))); offset += n; }
(9)rename_workset_attrs
重新命名@__size , @__offset属性 。名字可以在Graph Color上设置,如下图:
@__size ---------> @workset_lengths
@__offset----------> @workset_begins
上面是Graph Color 的算法,比较意外的是里面利用二进制的运算,来加大运算的效率。码字不易,转载请标明出处。






