poj 3233 Matrix Power Series(矩阵快速幂+二分)
Given a n × n matrix A and a positive integer k, find the sum S = A + A2 + A3 + … + Ak.
Input
The input contains exactly one test case. The first line of input contains three positive integers n (n ≤ 30), k (k ≤ 109) and m (m < 104). Then follow n lines each containing n nonnegative integers below 32,768, giving A’s elements in row-major order.
Output
Output the elements of S modulo m in the same way as A is given.
Sample Input
2 2 4
0 1
1 1
Sample Output
1 2
2 3
二分法:
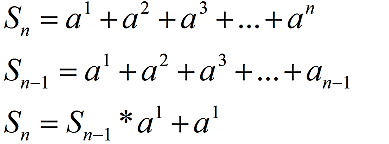
需要注意当n为偶数和奇数的时候。
Talk is cheap.just show the code!
#include<iostream> #include<cstdio> #include<cstring> using namespace std; #define LL long long #define m(s) memset(s,0,sizeof(s)) struct mat { int a[40][40]; }d; int n,m,k,mod; mat mul(mat a,mat b)//矩阵相乘 { mat ret; m(ret.a); for(int i=0;i<n;i++) for(int k=0;k<n;k++) { if(a.a[i][k]) { for(int j=0;j<n;j++) { ret.a[i][j]+=a.a[i][k]*b.a[k][j]; if(ret.a[i][j]>=mod) ret.a[i][j]%=mod; } } } return ret; } mat expo(mat a,int k)//矩阵快速幂 { if(k==1) return a; mat e; m(e.a); for(int i=0;i<n;i++) e.a[i][i]=1; if(k==0) return e; while(k) { if(k&1) e=mul(a,e); a=mul(a,a); k>>=1; } return e; } mat add(mat a,mat b)//矩阵相加 { mat t; for(int i=0;i<n;i++) for(int j=0;j<n;j++) t.a[i][j]=(a.a[i][j]+b.a[i][j])%mod; return t; } mat sum(int k)//矩阵求和 { if(k==1) return d; if(k&1) return add(sum(k-1),expo(d,k)); else { mat tmp=sum(k>>1); return add(tmp,mul(tmp,expo(d,k>>1))); } } int main() { while(cin>>n>>k>>mod) { mat ans; for(int i=0;i<n;i++) for(int j=0;j<n;j++) { cin>>d.a[i][j]; if(d.a[i][j]>=m) d.a[i][j]%=mod; } ans=sum(k); for(int i=0;i<n;i++) { for(int j=0;j<n;j++) { if(j) cout<<" "; cout<<ans.a[i][j]; } cout<<endl; } } return 0; }


