快速幂和大数取模的简单运用(以SPOJ LASTDIG - The last digit为例）

题目描述

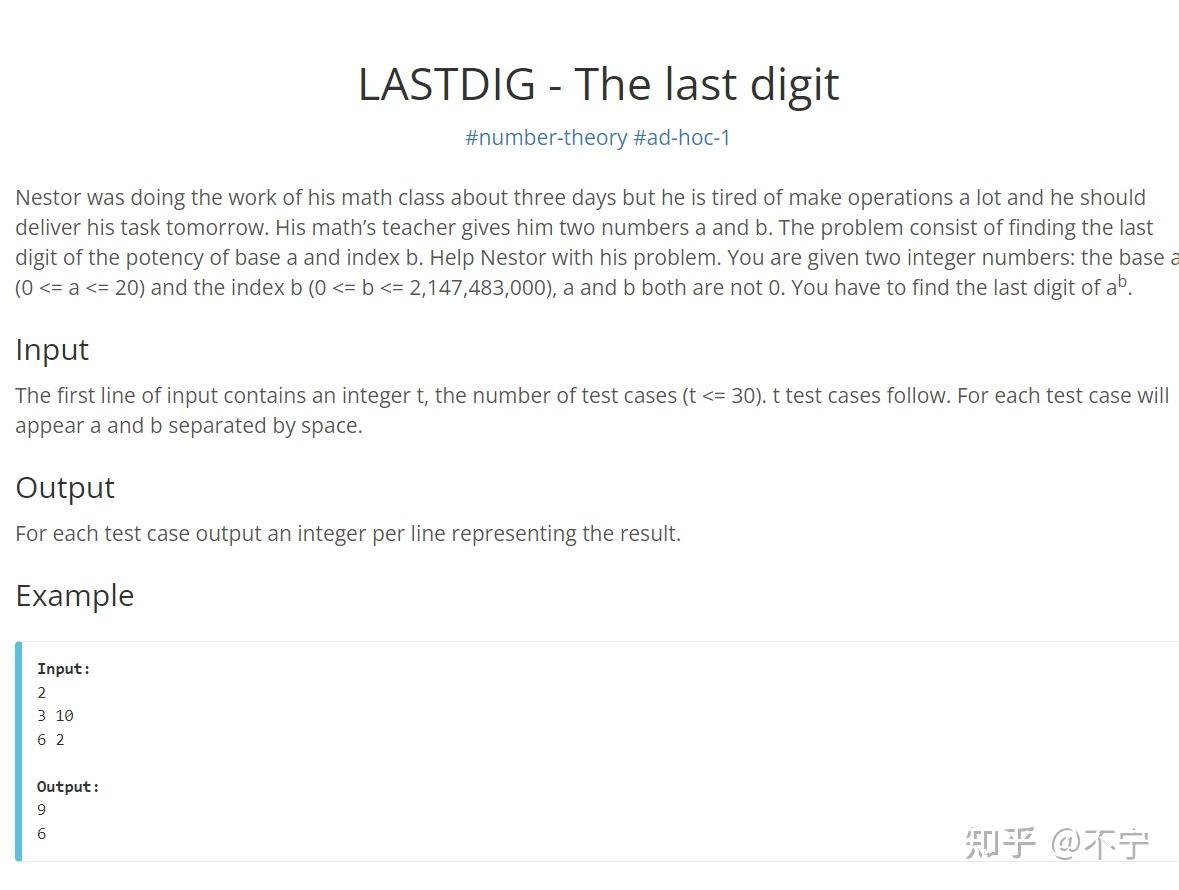
• 原文

Nestor was doing the work of his math class about three days but he is tired of make operations a lot and he should deliver his task tomorrow. His math’s teacher gives him two numbers a and b. The problem consist of finding the last digit of the potency of base a and index b. Help Nestor with his problem. You are given two integer numbers: the base a (0 <= a <= 20) and the index b (0 <= b <= 2,147,483,000), a and b both are not 0. You have to find the last digit of \(a^{b}\) .

• 中文翻译

Nestor 在数学课上做了三天的作业，但他已经厌倦了大量的运算，明天就该完成任务了。数学老师给了他两个数字 a 和 b。问题是找出基数 a 和指数 b 的结果的最后一位数。请帮助 Nestor 解决这个问题。老师给了你两个整数：基数 a（0 <= a <= 20）和指数 b（0 <= b <= 2,147,483,000），a 和 b 都不为 0。您必须找出 \(a^{b}\) 的最后一位数。

Input

The first line of input contains an integer t, the number of test cases (t <= 30). t test cases follow. For each test case will appear a and b separated by space.
第一行输入包含一个整数 t，即测试用例数（t <= 30）。每个测试用例的 a 和 b 用空格隔开。

Output

For each test case output an integer per line representing the result.
每个测试用例每行输出一个整数，代表测试结果。

Example

Input	Output
2 3 10 6 2	9 6

---

题解

一道快速幂&取余运算的模板题(笑^-)。

快速幂

快速幂是一种能迅速把\(a^{b}\) 求出来的算法,可以降低时间复杂度，防止TLE。

这里主要用了二分和递归的思路，把底数进行二分。当指数为偶数时，底数进行相乘，将指数除以2；当指数为奇数的时候，提出来一项底数，用ans进行积累，然后再按照偶数的方法递归。

long long qpow(long a,long n)
{
    long long ans = 1;
    while(n)
    {
        if (n % 2) ans *= a;//指数为奇数
        a = a * a;//底数平方
        n /= 2;//指数减半
    }
    return ans;
}

大数取模

• 原理展示

#include <iostream>
typedef long long ll;
using namespace std;
#define endl '\n'
int main()
{
    ll base,power;
    cin >> base >> power；
    
        if (base == 0 && power == 0) break;
        ll res = 1;
        //执行power次循环,每次结果都乘一个base,即base的power次方
        for (ll i = 1;i <= power;i++)
        {
            res = res * base;
            res = res % 10;
        }
        ll ans = res % 10;
        cout << ans << endl;
    return 0;
}

AC代码

#include <iostream>
typedef long long ll; 
#define endl '\n'
using namespace std;
int t; ll a,b;ll c;
ll qpow(ll a,ll n)
{
    ll ans = 1;
    while(n)
    {
        if (n & 1) ans = ans * a % 10;//位运算
        a = a * a % 10;
        n >>= 1;//n >> 1可将原数减半,n >>= 1等价于n = n / 2
    }
    return ans;
}
void solve()
{
	cin>>a>>b;
	c=qpow(a,b);
	cout<<c<<endl;
}
int main()
{std::ios::sync_with_stdio(false);
     cin.tie(0);
     cout.tie(0);//降低时间复杂度
    cin>>t;
    while(t--)
    {
    	solve();
    }
    return 0;
}