01分数规划POJ2976（简单模板题）

Dropping tests

Time Limit: 1000MS		Memory Limit: 65536K
Total Submissions: 7276		Accepted: 2523

Description

In a certain course, you take n tests. If you get a_i out of b_i questions correct on test i, your cumulative average is defined to be

.

Given your test scores and a positive integer k, determine how high you can make your cumulative average if you are allowed to drop any k of your test scores.

Suppose you take 3 tests with scores of 5/5, 0/1, and 2/6. Without dropping any tests, your cumulative average is 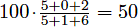. However, if you drop the third test, your cumulative average becomes .

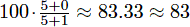Input

The input test file will contain multiple test cases, each containing exactly three lines. The first line contains two integers, 1 ≤ n ≤ 1000 and 0 ≤ k < n. The second line contains n integers indicating a_i for all i. The third line contains n positive integers indicating b_ifor all i. It is guaranteed that 0 ≤ a_i ≤ b_i ≤ 1, 000, 000, 000. The end-of-file is marked by a test case with n = k = 0 and should not be processed.

Output

For each test case, write a single line with the highest cumulative average possible after dropping k of the given test scores. The average should be rounded to the nearest integer.

Sample Input

3 1
5 0 2
5 1 6
4 2
1 2 7 9
5 6 7 9
0 0

Sample Output

83
100

Hint

To avoid ambiguities due to rounding errors, the judge tests have been constructed so that all answers are at least 0.001 away from a decision boundary (i.e., you can assume that the average is never 83.4997).

题意：给定n个二元组(a,b)，删除k个二元组，使得剩下的a元素之和与b元素之和的比率最大，最后的比率乘于100，然后输出跟最大比率最接近的整数

分析：设r=sigma(ai*xi)/sigma(bi*xi);其中xi={0,1},sigma(xi)=n-k,设R为最优值，

即：r<=R

即：sigma(ai*xi)/sigma(bi*xi)<=R

即：sigma(ai*xi)-sigma(R*bi*xi)<=0

也就是说sigma(ai*xi)-sigma(R*bi*xi)的最大值为0，

等价于 sigma((ai-R*bi)*xi)的最大值等于0；

因为h(r)=sigma((ai-R*bi)*xi)为单调递减函数，

所以可以二分求

 

#include"stdio.h"
#include"algorithm"
#include"string.h"
#include"iostream"
#include"queue"
#include"map"
#include"stack"
#include"cmath"
#include"vector"
#include"string"
#define M 1009
#define N 20003
#define eps 1e-7
#define mod 123456
#define inf 100000000
using namespace std;
int a[M],b[M],n,k;
double s[M];
int cmp(double a,double b)
{
    return a>b;
}
double fun(int n,double r)
{
    for(int i=1;i<=n;i++)
        s[i]=a[i]-r*b[i];
    sort(s+1,s+n+1,cmp);
    double sum=0;
    for(int i=1;i<=n-k;i++)
        sum+=s[i];
    return sum;
}
int main()
{
    while(scanf("%d%d",&n,&k),n||k)
    {
        double l=0,r=0,mid=0;
        for(int i=1;i<=n;i++)
        {
            scanf("%d",&a[i]);
            r+=a[i];
        }
        for(int i=1;i<=n;i++)
            scanf("%d",&b[i]);
        while(r-l>eps)
        {
            mid=(l+r)/2;
            double msg=fun(n,mid);
            if(msg<0)
            {
                r=mid;
            }
            else
            {
                l=mid;
            }
        }
        printf("%.0lf\n",r*100);
    }
    return 0;
}