CodeForces 388A Fox and Box Accumulation (模拟)
Fox Ciel has n boxes in her room. They have the same size and weight, but they might have different strength. The i-th box can hold at most xi boxes on its top (we'll call xi the strength of the box).
Since all the boxes have the same size, Ciel cannot put more than one box directly on the top of some box. For example, imagine Ciel has three boxes: the first has strength 2, the second has strength 1 and the third has strength 1. She cannot put the second and the third box simultaneously directly on the top of the first one. But she can put the second box directly on the top of the first one, and then the third box directly on the top of the second one. We will call such a construction of boxes a pile.

Fox Ciel wants to construct piles from all the boxes. Each pile will contain some boxes from top to bottom, and there cannot be more than xi boxes on the top of i-th box. What is the minimal number of piles she needs to construct?
The first line contains an integer n (1 ≤ n ≤ 100). The next line contains n integers x1, x2, ..., xn (0 ≤ xi ≤ 100).
Output a single integer — the minimal possible number of piles.
3
0 0 10
2
5
0 1 2 3 4
1
4
0 0 0 0
4
9
0 1 0 2 0 1 1 2 10
3
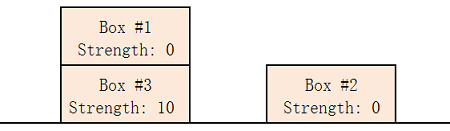
In example 1, one optimal way is to build 2 piles: the first pile contains boxes 1 and 3 (from top to bottom), the second pile contains only box 2.
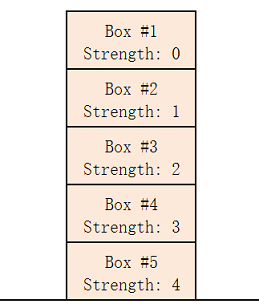
In example 2, we can build only 1 pile that contains boxes 1, 2, 3, 4, 5 (from top to bottom).
题目大意:一些盒子,数字代表其上面最多还能放多少,且上面放的数字不能大于其以下的。问最少堆几堆
题目分析:暴力模拟。从上往下一堆一堆的取,具体见程序凝视
#include <cstdio>
#include <cstring>
#include <algorithm>
using namespace std;
int a[105], hash[105];
int main()
{
int n, ma = -1;
memset(hash, 0, sizeof(hash));
scanf("%d", &n);
for(int i = 0; i < n; i++)
{
scanf("%d", &a[i]);
ma = max(a[i], ma);
hash[a[i]]++;
}
int ans = 0;
while(n)
{
int cnt = 0; //第i堆的个数
for(int i = 0; i <= ma; i++)
{
//有当前重量的,且其压力大于等于上面的个数
//则将其放到以下
while(hash[i] && i >= cnt)
{
hash[i] --; //放了一个,数量减1
cnt++; //这一堆数量加1
n --; //记录总的剩余个数
}
}
ans ++; //记录堆数
}
printf("%d\n", ans);
}

