BZOJ2115：[WC2011]Xor——题解

https://www.lydsy.com/JudgeOnline/problem.php?id=2115

https://www.luogu.org/problemnew/show/P4151

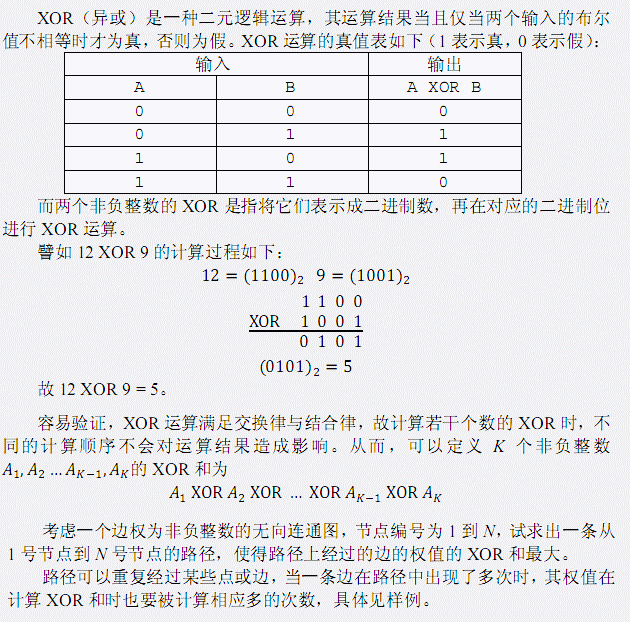
这道题当年还是新题，现在都成线性基套路题了。

参考：https://blog.sengxian.com/algorithms/linear-basis

一个1~n路径值可以拆成一条1~n的路径值^几个环（因为去到环和回来的路的值被异或回去了）。

于是就变成了处理出所有环的异或值和所有1~n的无环路的异或值，然后把环的异或值扔到线性基里面，剩下的就是套路了。

#include<cstdio>
#include<iostream>
#include<cstring>
#include<cmath>
#include<cctype>
#include<algorithm>
using namespace std;
typedef long long ll;
const int N=50010;
const int M=200010;
const int BASE=60;
inline ll read(){
    ll X=0,w=0;char ch=0;
    while(!isdigit(ch)){w|=ch=='-';ch=getchar();}
    while(isdigit(ch))X=(X<<3)+(X<<1)+(ch^48),ch=getchar();
    return w?-X:X;
}
struct node{
    int to,nxt;
    ll w;
}e[M];
int cnt,n,m,head[N],tot,num;
ll a[M],b[BASE+4],s[M],t[M];
bool vis[N];
inline void add(int u,int v,ll w){
    e[++cnt].to=v;e[cnt].w=w;e[cnt].nxt=head[u];head[u]=cnt;
}
void dfs(int u,ll sum){
    vis[u]=1;t[u]=sum;
    for(int i=head[u];i;i=e[i].nxt){
    int v=e[i].to;ll w=e[i].w;
    ll ans=sum^w^t[v];
    if(vis[v]){
        if(ans)a[++tot]=ans;
        continue;
    }
    dfs(v,sum^w);
    }
    if(u==n)s[++num]=sum;
    return;
}
int main(){
    n=read(),m=read();
    for(int i=1;i<=m;i++){
    int u=read(),v=read();ll w=read();
    add(u,v,w);add(v,u,w);
    }
    dfs(1,0);
    for(int i=1;i<=tot;i++){
    for(int j=BASE;j>=0;j--){
        if(a[i]>>j&1){
        if(b[j])a[i]^=b[j];
        else{
            b[j]=a[i];
            break;
        }
        }
    }
    }
    ll ans=0;
    for(int i=1;i<=num;i++){
    ll tmp=s[i];
    for(int j=BASE;j>=0;j--){
        tmp=max(tmp,tmp^b[j]);
    }
    ans=max(ans,tmp);
    }
    printf("%lld\n",ans);
    return 0;
}

+++++++++++++++++++++++++++++++++++++++++++

+本文作者：luyouqi233。 　　　　　　　　　　　　　　+

+欢迎访问我的博客：http://www.cnblogs.com/luyouqi233/+

+++++++++++++++++++++++++++++++++++++++++++