代码随想录算法训练营day16 | leetcode 104. 二叉树的最大深度、559. N 叉树的最大深度、111. 二叉树的最小深度、222. 完全二叉树的节点个数
104.二叉树的最大深度、111.二叉树的最小深度 见博文
代码随想录算法训练营day15 | leetcode 【二叉树的层序遍历十题】、226. 翻转二叉树、101. 对称二叉树、100. 相同的树、572. 另一棵树的子树
题目链接:559. N 叉树的最大深度-简单
题目描述:
给定一个 N 叉树,找到其最大深度。
最大深度是指从根节点到最远叶子节点的最长路径上的节点总数。
N 叉树输入按层序遍历序列化表示,每组子节点由空值分隔(请参见示例)。
示例 1:
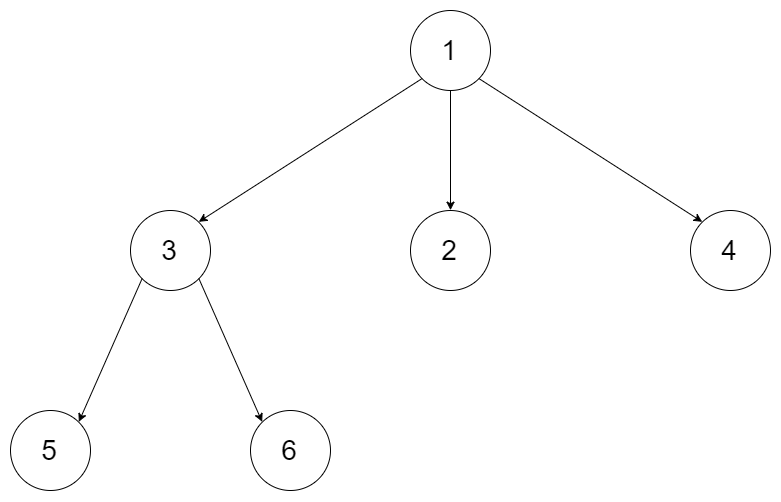
输入:root = [1,null,3,2,4,null,5,6]
输出:3
示例 2:
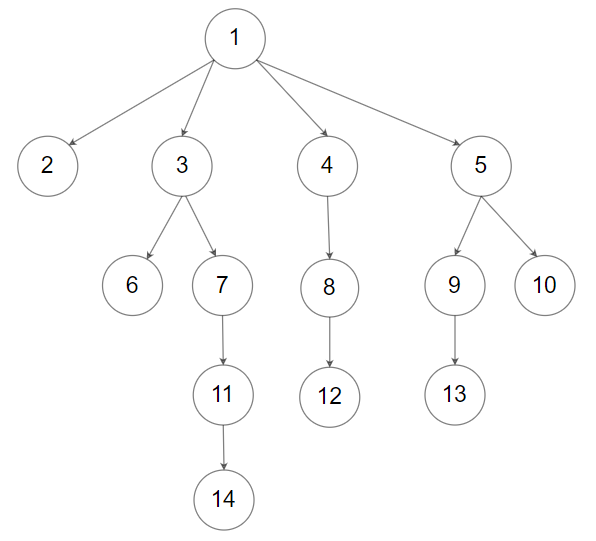
输入:root = [1,null,2,3,4,5,null,null,6,7,null,8,null,9,10,null,null,11,null,12,null,13,null,null,14]
输出:5
提示:
- 树的深度不会超过
1000。 - 树的节点数目位于
[0, 104]之间。
代码注释处报错了。
代码如下:
/*
// Definition for a Node.
class Node {
public:
int val;
vector<Node*> children;
Node() {}
Node(int _val) {
val = _val;
}
Node(int _val, vector<Node*> _children) {
val = _val;
children = _children;
}
};
*/
class Solution {
public:
int maxDepth(Node* root) {
if(root == NULL) return 0;
// vector<int> subDepth(root->children.size());
int depth = 0;
for(int i = 0; i < root->children.size(); ++i){
// subDepth.push_back(maxDepth(root->children[i]));
depth = max(depth, maxDepth(root->children[i]));
}
// return *max_element(subDepth.begin(), subDepth.end()) + 1;
return depth + 1;
}
};
题目链接:222. 完全二叉树的节点个数-简单
题目描述:
给你一棵 完全二叉树 的根节点 root ,求出该树的节点个数。
完全二叉树 的定义如下:在完全二叉树中,除了最底层节点可能没填满外,其余每层节点数都达到最大值,并且最下面一层的节点都集中在该层最左边的若干位置。若最底层为第 h 层,则该层包含 1~ 2h 个节点。
示例 1:

输入:root = [1,2,3,4,5,6]
输出:6
示例 2:
输入:root = []
输出:0
示例 3:
输入:root = [1]
输出:1
提示:
- 树中节点的数目范围是
[0, 5 * 104] 0 <= Node.val <= 5 * 104- 题目数据保证输入的树是 完全二叉树
进阶:遍历树来统计节点是一种时间复杂度为 O(n) 的简单解决方案。你可以设计一个更快的算法吗?
迭代以及递归时间复杂度均为O(n)
代码如下:
// 时间复杂度:O(n)
// 空间复杂度:O(nlogn)
/**
* Definition for a binary tree node.
* struct TreeNode {
* int val;
* TreeNode *left;
* TreeNode *right;
* TreeNode() : val(0), left(nullptr), right(nullptr) {}
* TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
* TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
* };
*/
class Solution {
public:
int countNodes(TreeNode* root) {
if(root == NULL) return 0;
int count = 0;
count += countNodes(root->left);
count += countNodes(root->right);
return count + 1;
}
};
精简后代码如下:
class Solution {
public:
int countNodes(TreeNode* root) {
if (root == NULL) return 0;
return 1 + countNodes(root->left) + countNodes(root->right);
}
};



