排序算法一计数排序

欢迎光临我的博客[http://poetize.cn]，前端使用VUE2，聊天室使用VUE3，后台使用Spring Boot

 

简介

计数排序是一个非基于比较的排序算法，该算法于1954年由 Harold H. Seward 提出。

它的优势在于在对一定范围内的整数排序时，它的复杂度为Ο(n+k)（其中k是整数的范围），快于任何比较排序算法。

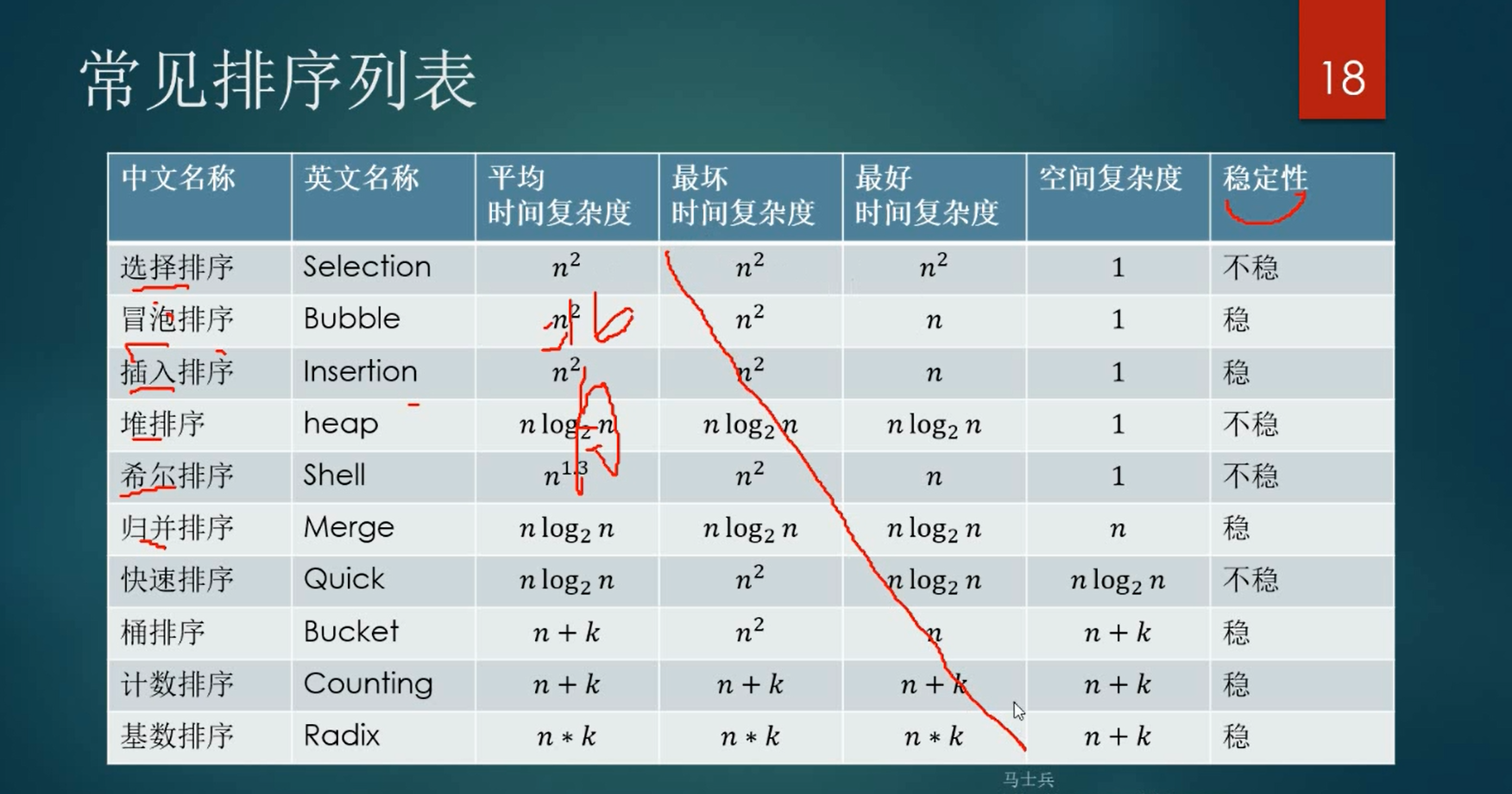
1. count sort，以空间换时间
2. 时间复杂度O（n）
3. 空间复杂度O（max+n）max为要排序的数据中的最大值。

计数排序就是计算相同key的元素各有多少个，然后根据出现的次数累加而获得最终的位置信息。

 

计数排序有两个限制条件，

　　那就是存在一个正整数K，使得数组里面的所有元素的key值都不大于N，

　　key值都是非负整数。

class CountSort {
    public static void main(String[] args) {
        int[] arr = {-2, -32, -1, 5, 0, 3, -1, 6, 4, 5};
        int a = 0;
        int b = arr.length - 1;
        arr=countSort(arr, 0, b);
    }

    private static int[] countSort(int[] arr, int a, int b) {
        if (arr.length <= 1) return arr;
        
        //寻找最大与最小值
        int min = arr[0];
        int max = arr[0];
        for (int i = 1; i < arr.length; i++) {
            if (min > arr[i]) min = arr[i];
            if (max < arr[i]) max = arr[i];
        }
        
        //新数组
        int[] finalarr = new int[arr.length];
        //count储存元素的个数
        int[] count = new int[max - min + 1];
        
        for (int i = 0; i < arr.length; i++) {
            count[arr[i] - min]++;
        }

        //将count中储存的数据转移至finalarr
        int f = 0;
        for (int i = 0; i < count.length; i++) {
            for (int j = 0; j < count[i]; j++) {
                finalarr[f++] = i + min;
            }
        }
        return finalarr;
    }
}