P1827 [USACO3.4]美国血统 American Heritage 题解
一、已知前序+中序，求后序
（1）、二叉树的遍历，就是一个深度优先搜索的过程:
前序
const int N = 1e6 + 10;
//树的结构体+存储数组
struct Node {
int id; // 当前结点ID
int left; // 左结点ID
int right;// 右结点ID
} t[N];
int n;
void pre_order(int x){
printf("%d\n",x);
if(t[x].left) pre_order(t[x].left);
if(t[x].right) pre_order(t[x].right);
}
中序
void in_order(int x){
if(t[x].left) in_order(t[x].left);
printf("%d\n",x);
if(t[x].right) in_order(t[x].right);
}
后序
void post_order(int x){
if(t[x].left) post_order(t[x].left);
if(t[x].right) post_order(t[x].right);
printf("%d\n",x);
}
说白了，套路基本一样，就是三步：输出当前结点，递归左子树，递归右子树。根据输出顺序不同，产生了三种遍历方式。
算法步骤：
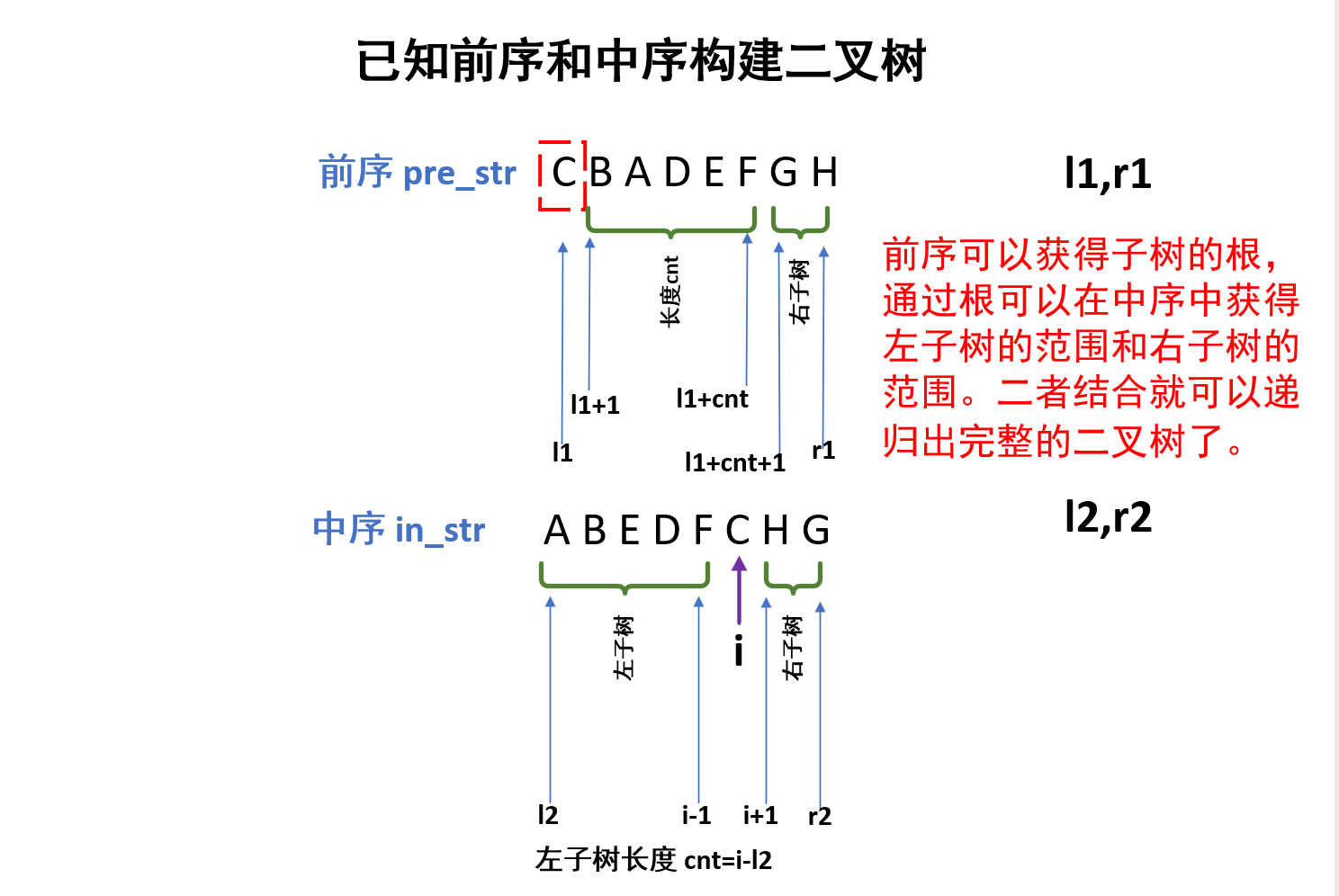
(1)给出一个前序遍历的字符串，那么首字母一定是子树的根。
(2)另外再给出同样子树的中序遍历字符串，那么通过上面查找到的首字母在中序遍历字符串中找到它，它的左边就是左子树，右边就是右子树。
(3)利用中序字符串左子树的范围和右子树的范围，就可以确定在前序字符串中左子树的范围和右子树的范围，通过递归就可以继续遍历左右子树。
很多二叉树的题目，不需要真正的还原创建出二叉树，只是根据字符串，不断的递归子串，在递归过程中输出想要的结果，这样更轻量一些，代码更短。当然，想要还原真正的二叉树也是完全可以的。
代码原理：
#include <bits/stdc++.h>
using namespace std;
string pre_str; //前序
string in_str; //中序
/**
* 功能：对二叉树进行后序遍历.根据四个参数，（1）确定子树的根，(2)确定左右子树的大小范围，（3）按后序进行输出递归
* @param l1 前序遍历的起点
* @param r1 前序遍历的终点
* @param l2 中序遍历的起点
* @param r2 中序遍历的终点
*/
void dfs(int l1, int r1, int l2, int r2) {
if (l1 > r1 || l2 > r2) return;//规定边界条件
int i = in_str.find(pre_str[l1]); //利用根左右的特性来在中序队列中查找
int cnt = i - l2;//左子树的节点个数
//前序：l1是根，左子树是从l1+1开始，到l1+cnt结束
//中序：l2开始，到i-1结束
dfs(l1 + 1, l1 + cnt, l2, i - 1); //递归左子树
dfs(l1 + cnt + 1, r1, i + 1, r2); //递归右子树
cout << pre_str[l1]; //输出根结点
}
/**
ABEDFCHG
CBADEFGH
参考结果：AEFDBHGC
*/
int main() {
//输入中序遍历，前序遍历字符串
cin >> in_str >> pre_str;
int right = in_str.size() - 1; //right索引：因为是0开始，所以要-1
//递归构建还原二叉树
//本题，并没有真正的进行构建二叉树，只是根据二叉树的特点，不断查找过程中，输出后序遍历的字符串
dfs(0, right, 0, right);//每个字符串有两个指针指向头和尾
return 0;
}
二、已知后序+中序，求前序
P1030 [NOIP2001 普及组] 求先序排列
https://www.luogu.com.cn/problem/P1030
姊妹题： https://www.cnblogs.com/littlehb/p/15088998.html
三、已知前序+后序，求中序个数
P1229 遍历问题
https://www.luogu.com.cn/problem/P1229
https://www.cnblogs.com/littlehb/p/15099843.html
