【树】二叉树的应用 I
1. 题目列表
| 序号 | 题目 | 难度 |
|---|---|---|
| 1 | 226. 翻转二叉树 | 简单 |
| 2 | 116. 填充每个节点的下一个右侧节点指针 | 中等 |
| 3 | 114. 二叉树展开为链表 | 中等 |
| 4 | 652. 寻找重复的子树 | 中等 |
2. 应用
2.1. Leetcode 226. 翻转二叉树
2.1.1. 题目
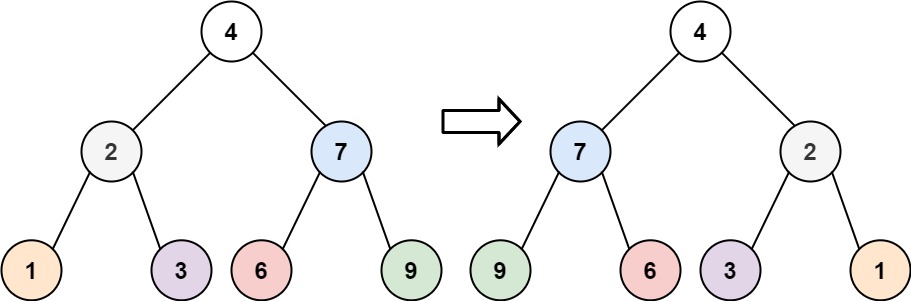
给你一棵二叉树的根节点 root ，翻转这棵二叉树，并返回其根节点。
示例 1：
输入：root = [4,2,7,1,3,6,9]
输出：[4,7,2,9,6,3,1]
2.1.2. 解题思路
2.1.2.1. 方法一：前序遍历
我们可以考虑在进入每个节点之前，在前序的位置交换每个节点的左右子树。
2.1.2.2. 方法二：后序遍历
我们也可以考虑在离开每个节点之后，在后序的位置交换每个节点的左右子树。
2.1.3. 代码实现
- 方法一：前序遍历
class Solution {
public TreeNode invertTree(TreeNode root) {
return dfs(root);
}
private TreeNode dfs(TreeNode root) {
if (root == null) {
return null;
}
TreeNode temp = root.left;
root.left = root.right;
root.right = temp;
dfs(root.right);
dfs(root.left);
return root;
}
}
- 方法二：后序遍历
class Solution {
public TreeNode invertTree(TreeNode root) {
return dfs(root);
}
private TreeNode dfs(TreeNode root) {
if (root == null) {
return null;
}
TreeNode right = dfs(root.right);
TreeNode left = dfs(root.left);
root.left = right;
root.right = left;
return root;
}
}
2.2. Leetcode 116. 填充每个节点的下一个右侧节点指针
2.2.1. 题目
给定一个 完美二叉树 ，其所有叶子节点都在同一层，每个父节点都有两个子节点。二叉树定义如下：
struct Node { int val; Node *left; Node *right; Node *next; }填充它的每个 next 指针，让这个指针指向其下一个右侧节点。如果找不到下一个右侧节点，则将 next 指针设置为 NULL。
初始状态下，所有 next 指针都被设置为 NULL。
示例 1：
输入：root = [1,2,3,4,5,6,7]
输出：[1,#,2,3,#,4,5,6,7,#]
解释：给定二叉树如图 A 所示，你的函数应该填充它的每个 next 指针，以指向其下一个右侧节点，如图 B 所示。序列化的输出按层序遍历排列，同一层节点由 next 指针连接，'#' 标志着每一层的结束。
2.2.2. 解题思路
2.2.2.1. 方法一：广度优先搜索
我们可以使用广度优先搜索的思路，将每一层的元素依次指向右侧的元素即可。
2.2.2.2. 方法二：深度优先搜索
我们使用深度优先搜索的思路，同时搜索一个节点的左右子树，将每一个左子树的根节点指向，右子树的根节点即可。
同时，我们还需要将当前节点的右子树，与同层的相邻节点的左子树相连。
2.2.3. 代码实现
- 方法一：广度优先搜索
class Solution {
public Node connect(Node root) {
if (root == null) {
return null;
}
bfs(root);
return root;
}
private void bfs(Node root) {
Deque<Node> queue = new ArrayDeque<>();
queue.offer(root);
root.next = null;
while (!queue.isEmpty()) {
int size = queue.size();
Node last = null;
for (int i = 0; i < size; i++) {
Node candidate = queue.poll();
if (candidate.left != null) {
queue.offer(candidate.left);
}
if (candidate.right != null) {
queue.offer(candidate.right);
}
if (last != null) {
last.next = candidate;
}
last = candidate;
}
last.next = null;
}
}
}
- 方法二：深度优先搜索
class Solution {
public Node connect(Node root) {
if (root == null) {
return null;
}
dfs(root.left, root.right);
return root;
}
private void dfs(Node p, Node q) {
if (p == null || q == null) {
return ;
}
p.next = q;
dfs(p.left, p.right);
dfs(p.right, q.left);
dfs(q.left, q.right);
}
}
2.3. Leetcode 114. 二叉树展开为链表
2.3.1. 题目
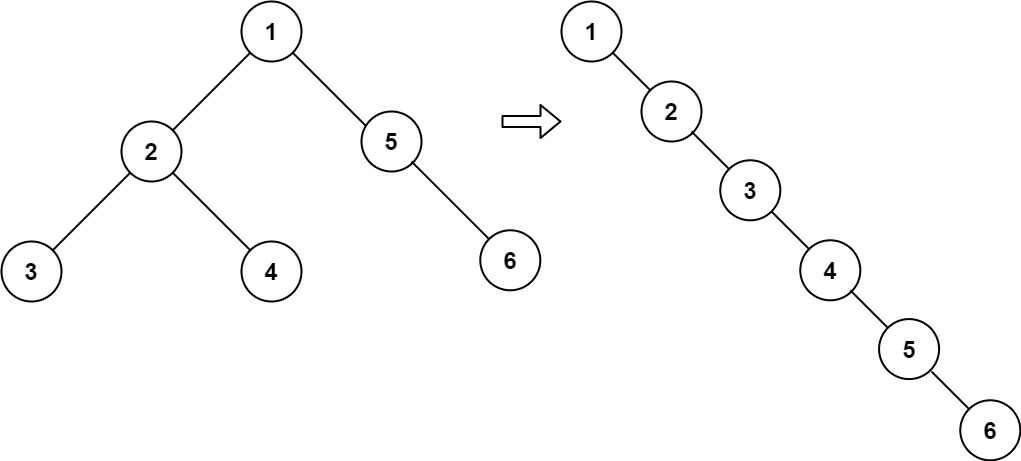
给你二叉树的根结点 root ，请你将它展开为一个单链表：
- 展开后的单链表应该同样使用 TreeNode ，其中 right 子指针指向链表中下一个结点，而左子指针始终为 null 。
- 展开后的单链表应该与二叉树 先序遍历 顺序相同。
示例 1：
输入：root = [1,2,5,3,4,null,6]
输出：[1,null,2,null,3,null,4,null,5,null,6]
2.3.2. 解题思路
比较直观的思路，是在每一个节点的后序遍历的位置，将它的左子树插入到它的右子树的位置，然后将原始的右子树连接到新的右子树尾部。
2.3.3. 代码实现
class Solution {
public void flatten(TreeNode root) {
dfs(root);
}
private TreeNode dfs(TreeNode root) {
if (root == null) {
return null;
}
TreeNode left = dfs(root.left);
TreeNode right = dfs(root.right);
// 将左子树插入到右子树的位置
root.left = null;
root.right = left;
// 将原始的右子树接到新的右子树尾部
TreeNode node = root;
while (node.right != null) {
node = node.right;
}
node.right = right;
return root;
}
}
2.4. Leetcode 652. 寻找重复的子树
2.4.1. 题目
给你一棵二叉树的根节点 root ，返回所有 重复的子树 。对于同一类的重复子树，你只需要返回其中任意 一棵 的根结点即可。如果两棵树具有 相同的结构 和 相同的结点值 ，则认为二者是 重复 的。
示例 1：
输入：root = [1,2,3,4,null,2,4,null,null,4]
输出：[[2,4],[4]]
2.4.2. 解题思路
这里是在求相同的子树，所以，我们很容易想到在后序遍历的位置，在离开每一个节点时，记录当前节点及其子树的状态，这里我们可以考虑使用字符串序列化每一个子树的状态。
同时，为了去重，我们可以使用一个哈希表来记录已有的结果，如果遇到重复的子树，只添加一次即可。
注意，序列化一棵子树的顺序一定要和访问这棵子树的顺序相同。
例如，如下代码实现是按照后序遍历，即访问顺序为：左子树 -> 右子树 -> 根节点，那么，序列化的时候，也要按照相同的方式记录后序遍历的路径。
2.4.3. 代码实现
class Solution {
public List<TreeNode> findDuplicateSubtrees(TreeNode root) {
List<TreeNode> result = new ArrayList<>();
Map<String, Integer> cache = new HashMap<>();
dfs(root, result, cache);
return result;
}
private String dfs(TreeNode root, List<TreeNode> result, Map<String, Integer> cache) {
if (root == null) {
return "#";
}
String left = dfs(root.left, result, cache);
String right = dfs(root.right, result, cache);
String subTree = left + "." + right + "." + root.val;
int count = cache.getOrDefault(subTree, 0);
if (count == 1) {
result.add(root);
}
cache.put(subTree, count + 1);
return subTree;
}
}
参考：
