poj1416 Shredding Company
Shredding Company
| Time Limit: 1000MS | Memory Limit: 10000K | |
| Total Submissions: 5379 | Accepted: 3023 |
Description
You have just been put in charge of developing a new shredder for the Shredding Company Although a "normal" shredder would just shred sheets of paper into little pieces so that the contents would become unreadable, this new shredder needs to have the following unusual basic characteristics.
1.The shredder takes as input a target number and a sheet of paper with a number written on it.
2.It shreds (or cuts) the sheet into pieces each of which has one or more digits on it.
3.The sum of the numbers written on each piece is the closest possible number to the target number, without going over it.
For example, suppose that the target number is 50, and the sheet of paper has the number 12346. The shredder would cut the sheet into four pieces, where one piece has 1, another has 2, the third has 34, and the fourth has 6. This is because their sum 43 (= 1 + 2 + 34 + 6) is closest to the target number 50 of all possible combinations without going over 50. For example, a combination where the pieces are 1, 23, 4, and 6 is not valid, because the sum of this combination 34 (= 1 + 23 + 4 + 6) is less than the above combination's 43. The combination of 12, 34, and 6 is not valid either, because the sum 52 (= 12 + 34 + 6) is greater than the target number of 50.
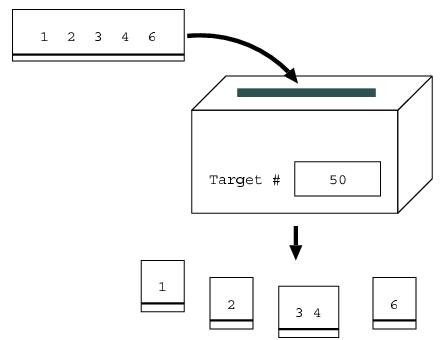
Figure 1. Shredding a sheet of paper having the number 12346 when the target number is 50
There are also three special rules :
1.If the target number is the same as the number on the sheet of paper, then the paper is not cut.
For example, if the target number is 100 and the number on the sheet of paper is also 100, then
the paper is not cut.
2.If it is not possible to make any combination whose sum is less than or equal to the target number, then error is printed on a display. For example, if the target number is 1 and the number on the sheet of paper is 123, it is not possible to make any valid combination, as the combination with the smallest possible sum is 1, 2, 3. The sum for this combination is 6, which is greater than the target number, and thus error is printed.
3.If there is more than one possible combination where the sum is closest to the target number without going over it, then rejected is printed on a display. For example, if the target number is 15, and the number on the sheet of paper is 111, then there are two possible combinations with the highest possible sum of 12: (a) 1 and 11 and (b) 11 and 1; thus rejected is printed. In order to develop such a shredder, you have decided to first make a simple program that would simulate the above characteristics and rules. Given two numbers, where the first is the target number and the second is the number on the sheet of paper to be shredded, you need to figure out how the shredder should "cut up" the second number.
1.The shredder takes as input a target number and a sheet of paper with a number written on it.
2.It shreds (or cuts) the sheet into pieces each of which has one or more digits on it.
3.The sum of the numbers written on each piece is the closest possible number to the target number, without going over it.
For example, suppose that the target number is 50, and the sheet of paper has the number 12346. The shredder would cut the sheet into four pieces, where one piece has 1, another has 2, the third has 34, and the fourth has 6. This is because their sum 43 (= 1 + 2 + 34 + 6) is closest to the target number 50 of all possible combinations without going over 50. For example, a combination where the pieces are 1, 23, 4, and 6 is not valid, because the sum of this combination 34 (= 1 + 23 + 4 + 6) is less than the above combination's 43. The combination of 12, 34, and 6 is not valid either, because the sum 52 (= 12 + 34 + 6) is greater than the target number of 50.

Figure 1. Shredding a sheet of paper having the number 12346 when the target number is 50
There are also three special rules :
1.If the target number is the same as the number on the sheet of paper, then the paper is not cut.
For example, if the target number is 100 and the number on the sheet of paper is also 100, then
the paper is not cut.
2.If it is not possible to make any combination whose sum is less than or equal to the target number, then error is printed on a display. For example, if the target number is 1 and the number on the sheet of paper is 123, it is not possible to make any valid combination, as the combination with the smallest possible sum is 1, 2, 3. The sum for this combination is 6, which is greater than the target number, and thus error is printed.
3.If there is more than one possible combination where the sum is closest to the target number without going over it, then rejected is printed on a display. For example, if the target number is 15, and the number on the sheet of paper is 111, then there are two possible combinations with the highest possible sum of 12: (a) 1 and 11 and (b) 11 and 1; thus rejected is printed. In order to develop such a shredder, you have decided to first make a simple program that would simulate the above characteristics and rules. Given two numbers, where the first is the target number and the second is the number on the sheet of paper to be shredded, you need to figure out how the shredder should "cut up" the second number.
Input
The input consists of several test cases, each on one line, as follows :
tl num1
t2 num2
...
tn numn
0 0
Each test case consists of the following two positive integers, which are separated by one space : (1) the first integer (ti above) is the target number, (2) the second integer (numi above) is the number that is on the paper to be shredded.
Neither integers may have a 0 as the first digit, e.g., 123 is allowed but 0123 is not. You may assume that both integers are at most 6 digits in length. A line consisting of two zeros signals the end of the input.
tl num1
t2 num2
...
tn numn
0 0
Each test case consists of the following two positive integers, which are separated by one space : (1) the first integer (ti above) is the target number, (2) the second integer (numi above) is the number that is on the paper to be shredded.
Neither integers may have a 0 as the first digit, e.g., 123 is allowed but 0123 is not. You may assume that both integers are at most 6 digits in length. A line consisting of two zeros signals the end of the input.
Output
For each test case in the input, the corresponding output takes one of the following three types :
sum part1 part2 ...
rejected
error
In the first type, partj and sum have the following meaning :
1.Each partj is a number on one piece of shredded paper. The order of partj corresponds to the order of the original digits on the sheet of paper.
2.sum is the sum of the numbers after being shredded, i.e., sum = part1 + part2 +...
Each number should be separated by one space.
The message error is printed if it is not possible to make any combination, and rejected if there is
more than one possible combination.
No extra characters including spaces are allowed at the beginning of each line, nor at the end of each line.
sum part1 part2 ...
rejected
error
In the first type, partj and sum have the following meaning :
1.Each partj is a number on one piece of shredded paper. The order of partj corresponds to the order of the original digits on the sheet of paper.
2.sum is the sum of the numbers after being shredded, i.e., sum = part1 + part2 +...
Each number should be separated by one space.
The message error is printed if it is not possible to make any combination, and rejected if there is
more than one possible combination.
No extra characters including spaces are allowed at the beginning of each line, nor at the end of each line.
Sample Input
50 12346 376 144139 927438 927438 18 3312 9 3142 25 1299 111 33333 103 862150 6 1104 0 0
Sample Output
43 1 2 34 6 283 144 139 927438 927438 18 3 3 12 error 21 1 2 9 9 rejected 103 86 2 15 0 rejected
Source
题目大意如下:给定你一个目标数字以及一张数字纸片,需要设计一种切割纸片的程序,使各个切片上数字之和加起来最接近目标数字且不超过它。额外地,有二个条件(话说那个相等条件似乎没用):其一,如果有多个得到最优解的切割方案,则输出"rejected";其二,若求不出合法解,那么输出"error"。
题解如下:由于原题描述用的是中式英语看不大懂,只好抄程序理解。主要思路是预处理求出任意区间代表的数字,从左到右一个一个地按顺序搜索区间,并记录路径,最后递归求解。详细的看我的程序注释吧。
15809294
| ksq2013 | 1416 | Accepted | 700K | 16MS | G++ | 1510B | 2016-07-25 21:11:48 |
#include<cstdio>
#include<cstring>
#include<iostream>
using namespace std;
bool more;
pair<int,int>pre[10][10],ans;
int lim,ln,d[10],num[10][10],minn;
void Init(char *p)
{
ln=strlen(p);
for(int i=0;i<ln;i++)
d[i]=p[i]-'0';
}
void Prep()//预处理程序;
{
memset(num,0,sizeof(num));
for(int i=0;i<ln;i++)
for(int j=i;j<ln;j++)
for(int k=i;k<=j;k++)
num[i][j]=num[i][j]*10+d[k];
}
bool dfs(int l,int r,int cnt)
{
if(r>=ln){
if(cnt>minn&&cnt<=lim){
more=false;
ans.first=l;
ans.second=r-1;//对应下方output函数的递归结束条件,非常巧妙的方法,避开了'0'这一特殊数字;
minn=cnt;
return true;
}
else if(cnt==minn)more=true;//多解了!;
return false;
}
bool adv=false;//advanced,记录该搜索子树的节点是否有解;
for(int i=1;i<=ln-r;i++){//看到i<=ln-r可知,i这里枚举的是准备切割区间的长度,例如(0,0)长度为1;
int now=r+i-1;
if(dfs(r,r+i,cnt+num[r][now])){
pre[r][now].first=l;
pre[r][now].second=r-1;
adv=true;
}
}
return adv;
}
void output(int l,int r)
{
if(l<0&&r<0)return;//对应上方dfs中的pre的存储方式,存的是r-1,所以最先前的区间的pre存的l和r都是-1,成为结束递归的条件;
output(pre[l][r].first,pre[l][r].second);
printf("%d ",num[l][r]);//直接按对应的l和r输出区间代表的数字;
}
int main()
{
char p[10];
while(~scanf("%d%s",&lim,p)){
if(!lim)break;
Init(p);
Prep();
more=false,minn=-1;
dfs(-1,0,0);
if(more){
puts("rejected");
continue;
}
if(minn==-1){
puts("error");
continue;
}
printf("%d ",minn);
output(ans.first,ans.second);//我试着用pair作为函数变量,但是不行,因为pre就是用l和r存的,所以只能代入first和second;
putchar('\n');
}
return 0;
}



