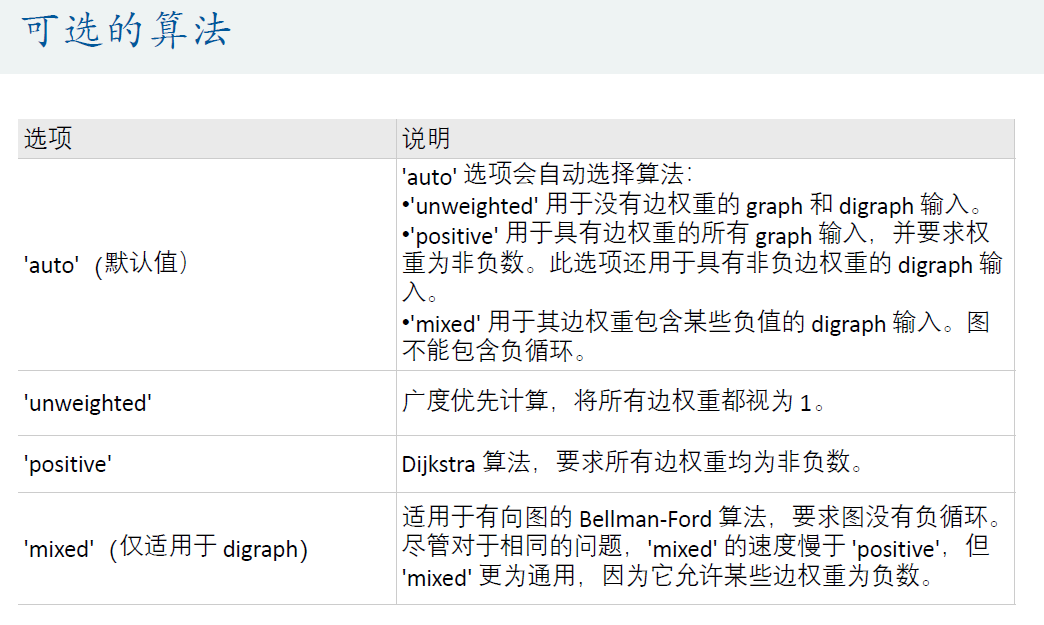
数模-图论（迪杰斯特拉算法）

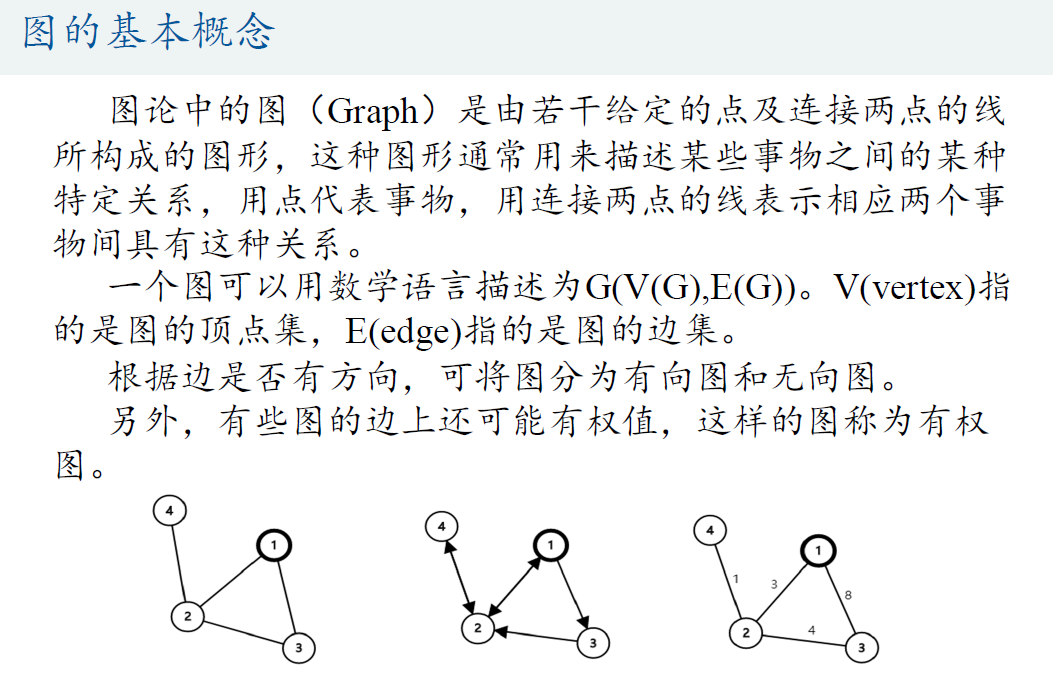
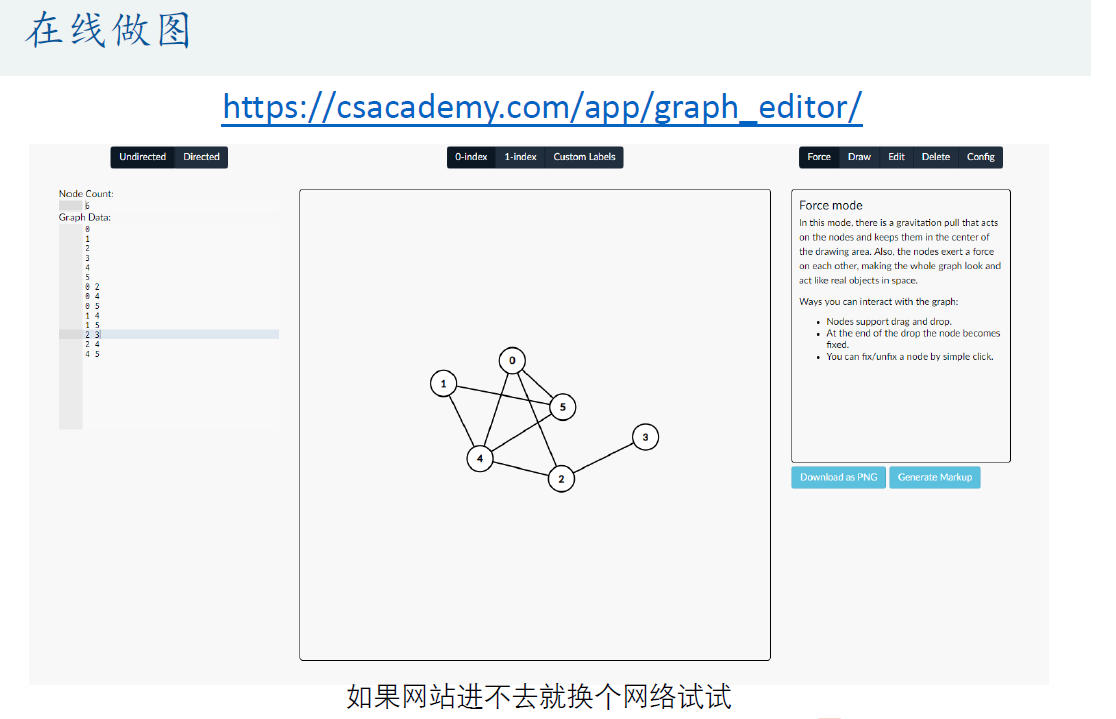
https://csacademy.com/app/graph_editor/

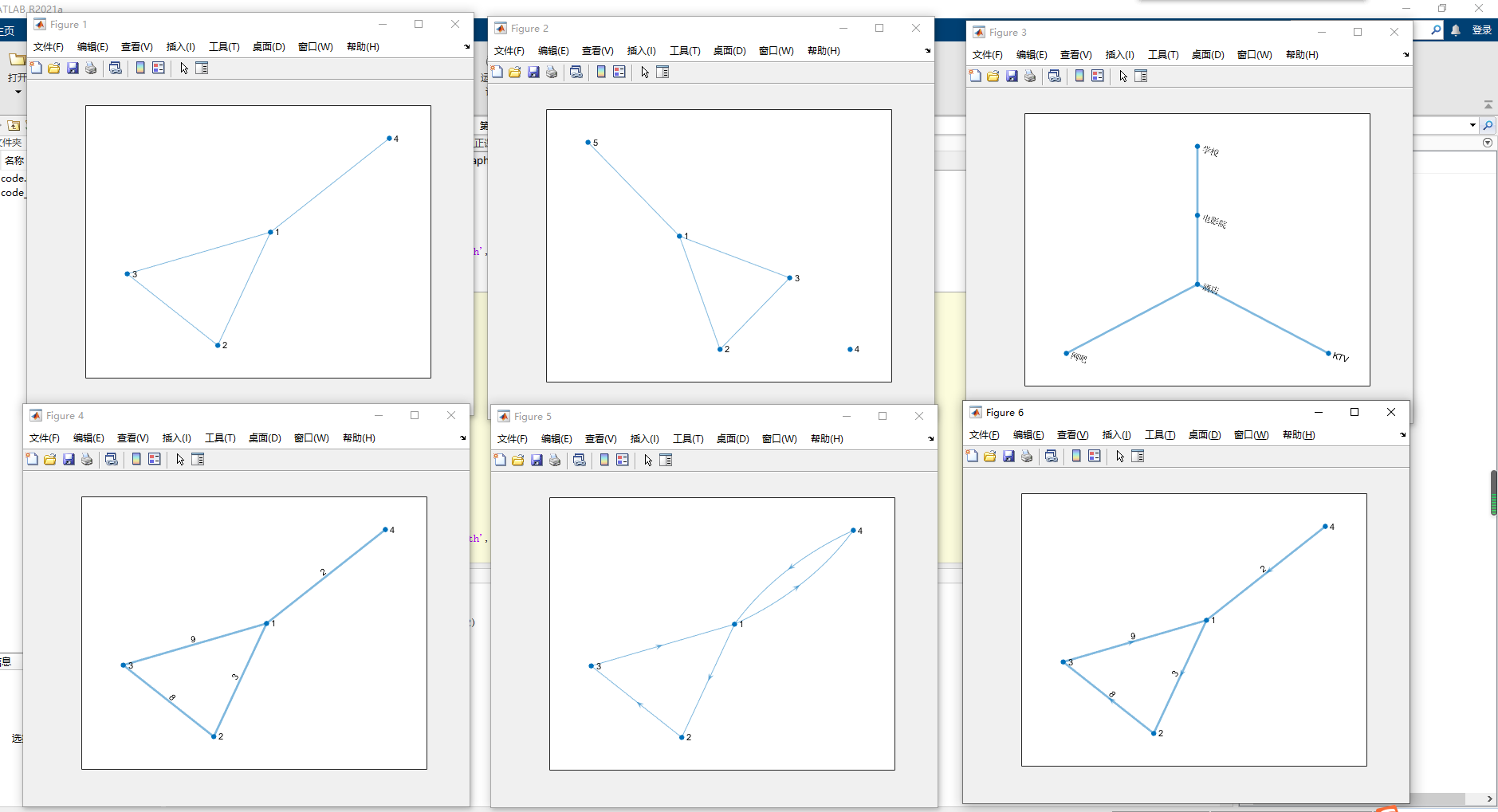
matlab做图的代码

%% 注意：以下代码需要较新版本的matlab才能运行（最好是2016版本及以上哦）
% 如果运行出错请下载新版的matlab代码再运行

%% Matlab作无向图
% （1）无权重（每条边的权重默认为1）
% 函数graph(s,t)：可在 s 和 t 中的对应节点之间创建边，并生成一个图
% s 和 t 都必须具有相同的元素数；这些节点必须都是从1开始的正整数，或都是字符串元胞数组。
s1 = [1,2,3,4];
t1 = [2,3,1,1];
G1 = graph(s1, t1);
plot(G1)
% 注意哦，编号最好是从1开始连续编号，不要自己随便定义编号
s1 = [1,2,3,5];
t1 = [2,3,1,1];
G1 = graph(s1, t1);
plot(G1)

% 注意字符串元胞数组是用大括号包起来的哦
s2 = {'学校','电影院','网吧','酒店'};
t2 = {'电影院','酒店','酒店','KTV'};
G2 = graph(s2, t2);
plot(G2, 'linewidth', 2)  % 设置线的宽度
% 下面的命令是在画图后不显示坐标
set( gca, 'XTick', [], 'YTick', [] );  

% （2）有权重
% 函数graph(s,t,w)：可在 s 和 t 中的对应节点之间以w的权重创建边，并生成一个图
s = [1,2,3,4];
t = [2,3,1,1];
w = [3,8,9,2];
G = graph(s, t, w);
plot(G, 'EdgeLabel', G.Edges.Weight, 'linewidth', 2) 
set( gca, 'XTick', [], 'YTick', [] );  

%% Matlab作有向图
% 无权图 digraph(s,t)
s = [1,2,3,4,1];
t = [2,3,1,1,4];
G = digraph(s, t);
plot(G)
set( gca, 'XTick', [], 'YTick', [] );  

% 有权图 digraph(s,t,w)
s = [1,2,3,4];
t = [2,3,1,1];
w = [3,8,9,2];
G = digraph(s, t, w);
plot(G, 'EdgeLabel', G.Edges.Weight, 'linewidth', 2) 
set( gca, 'XTick', [], 'YTick', [] );  

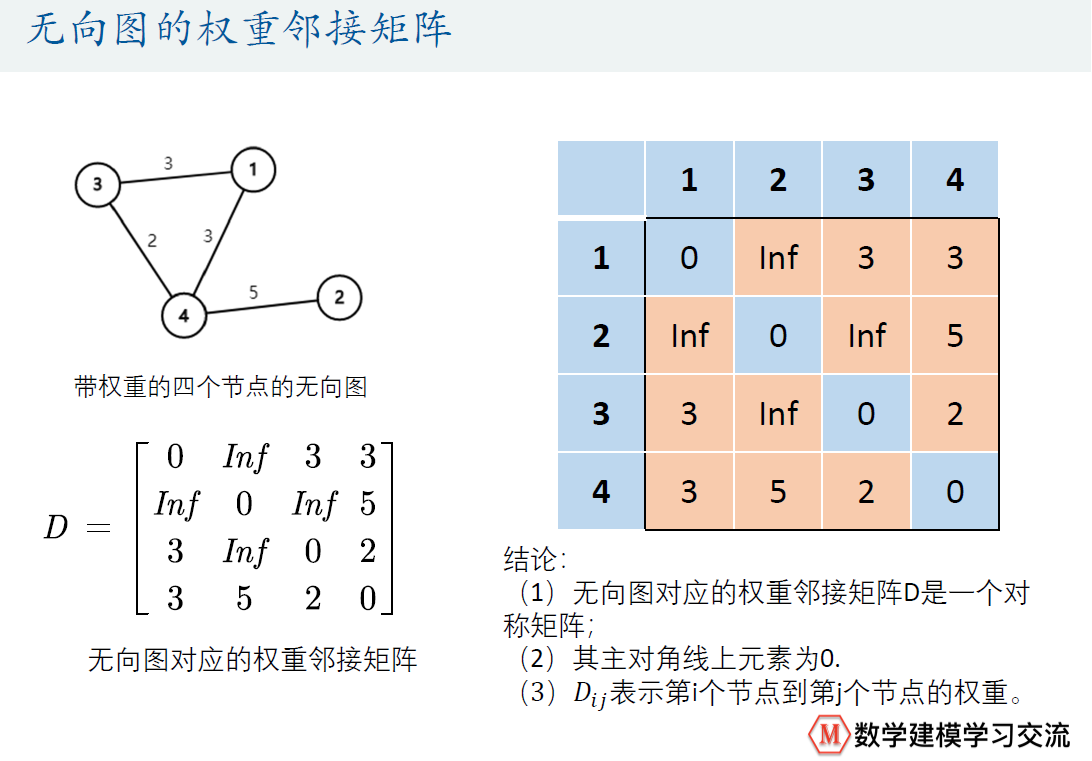
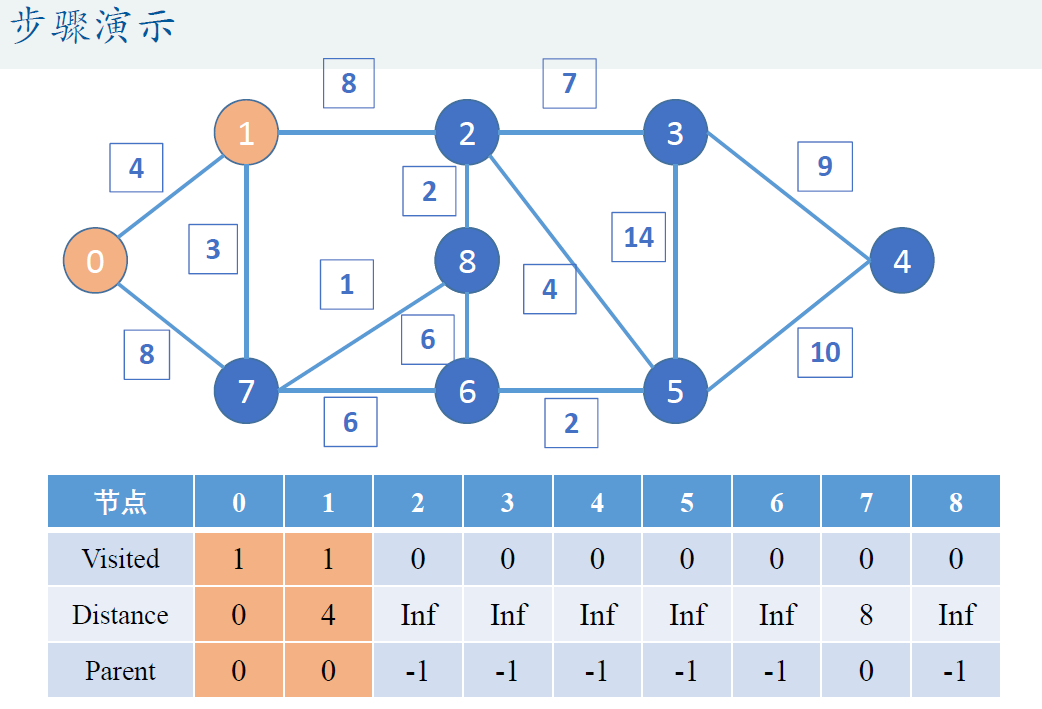
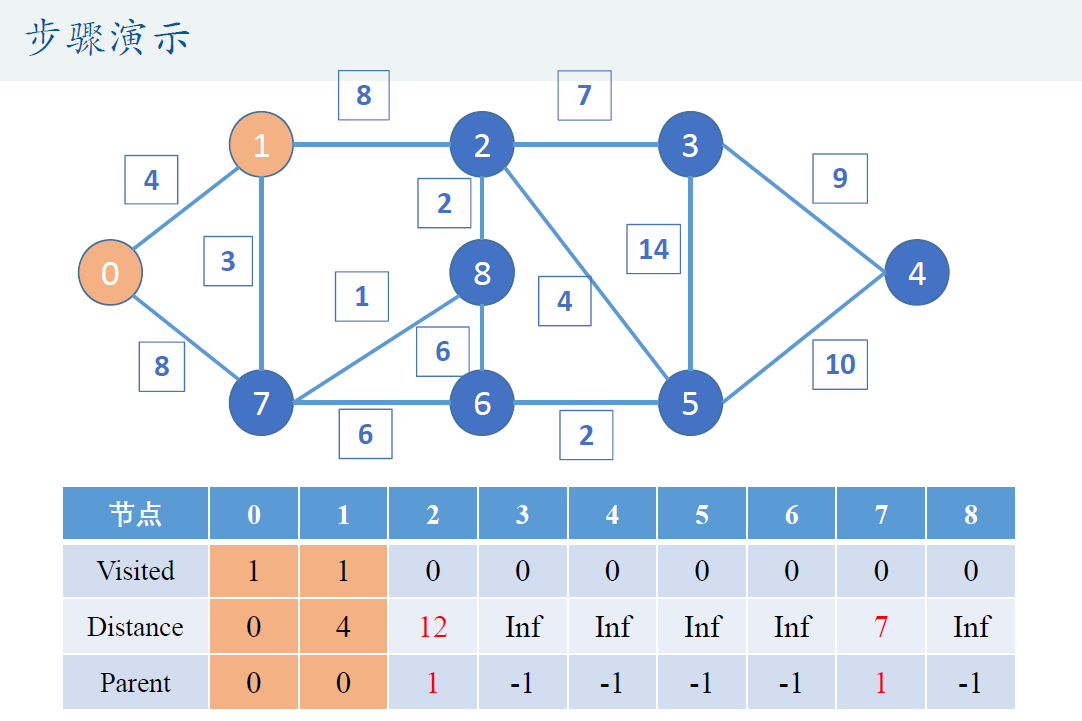
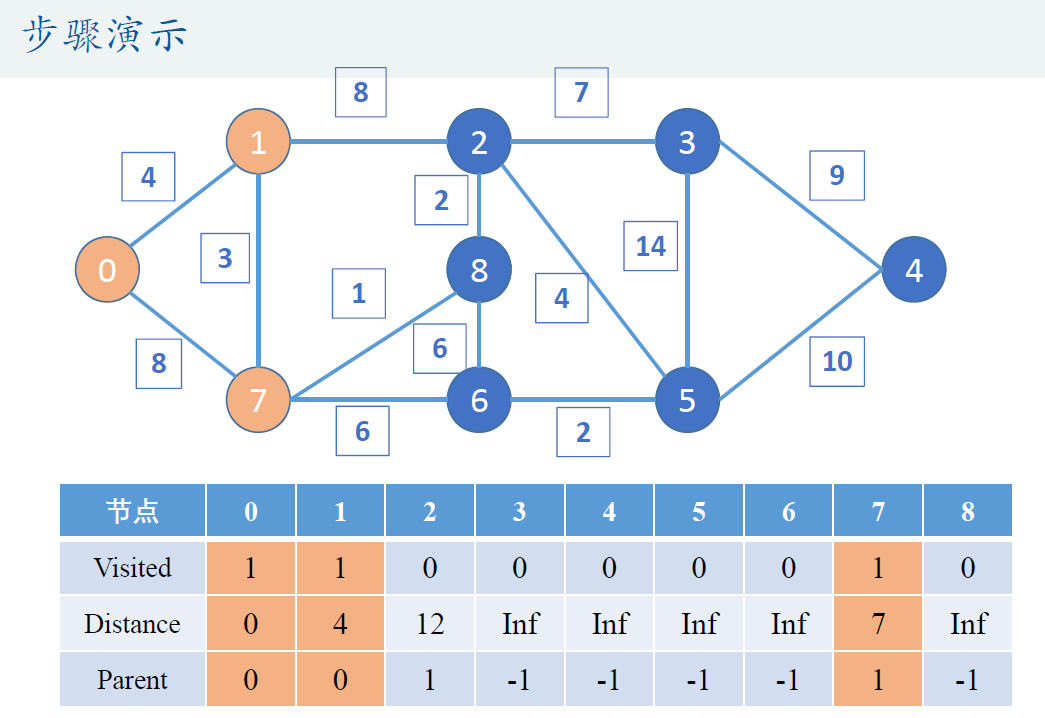
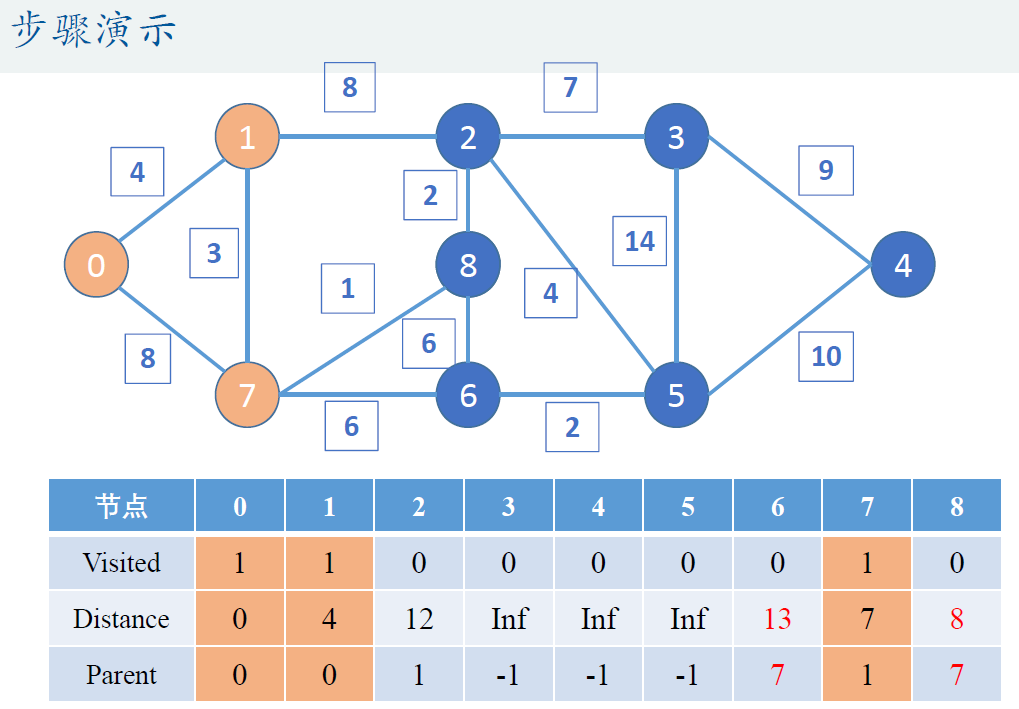
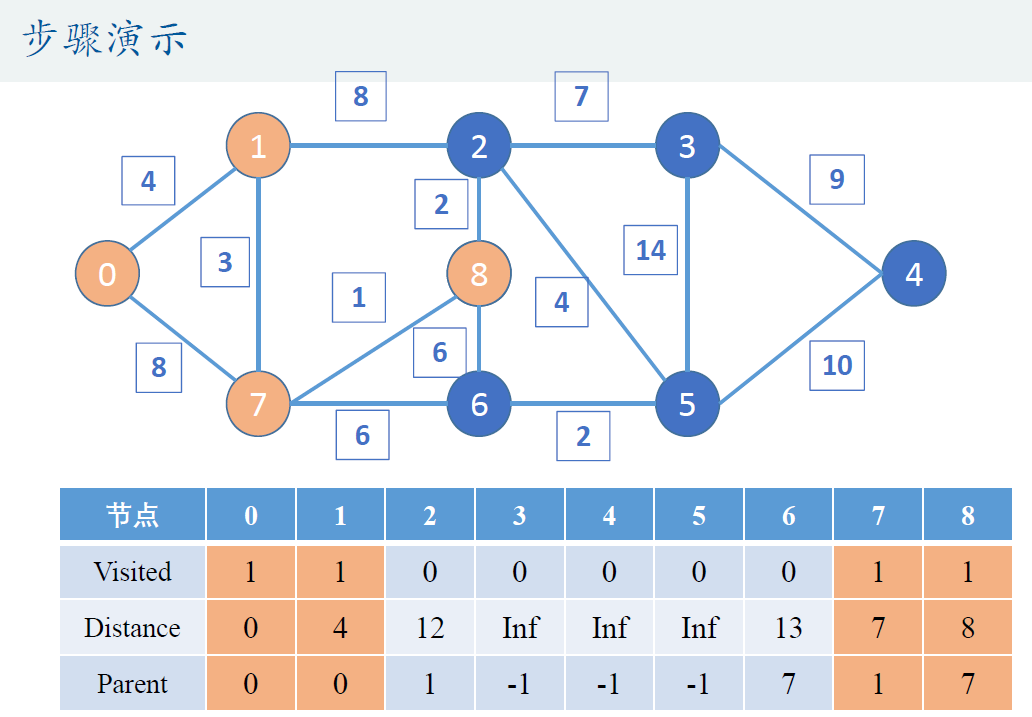
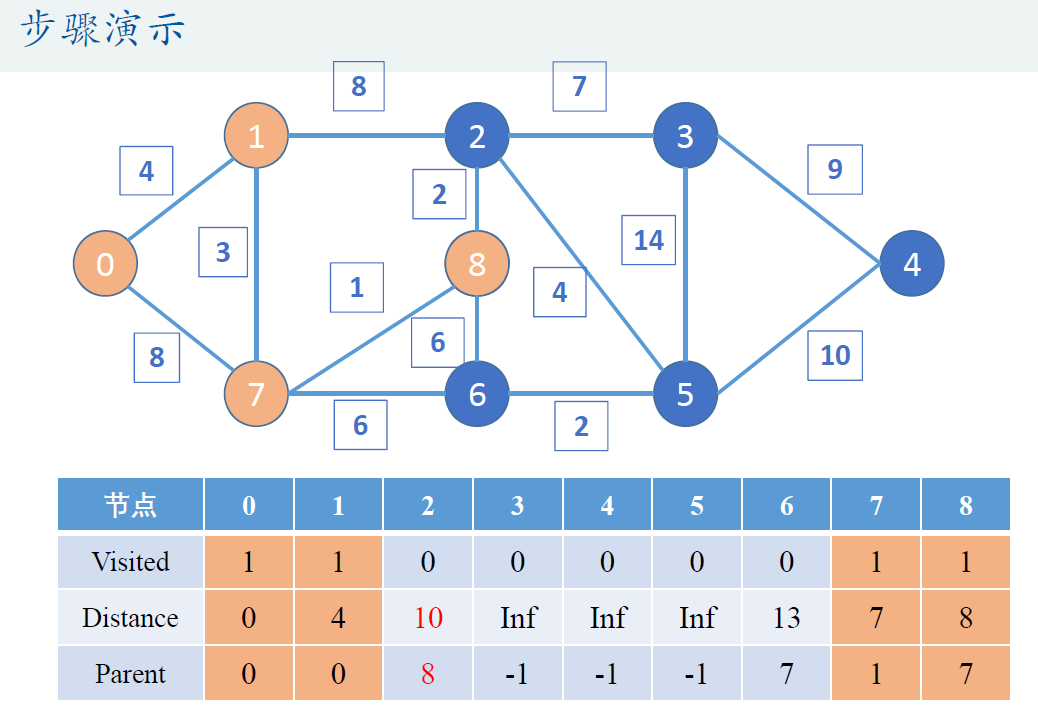
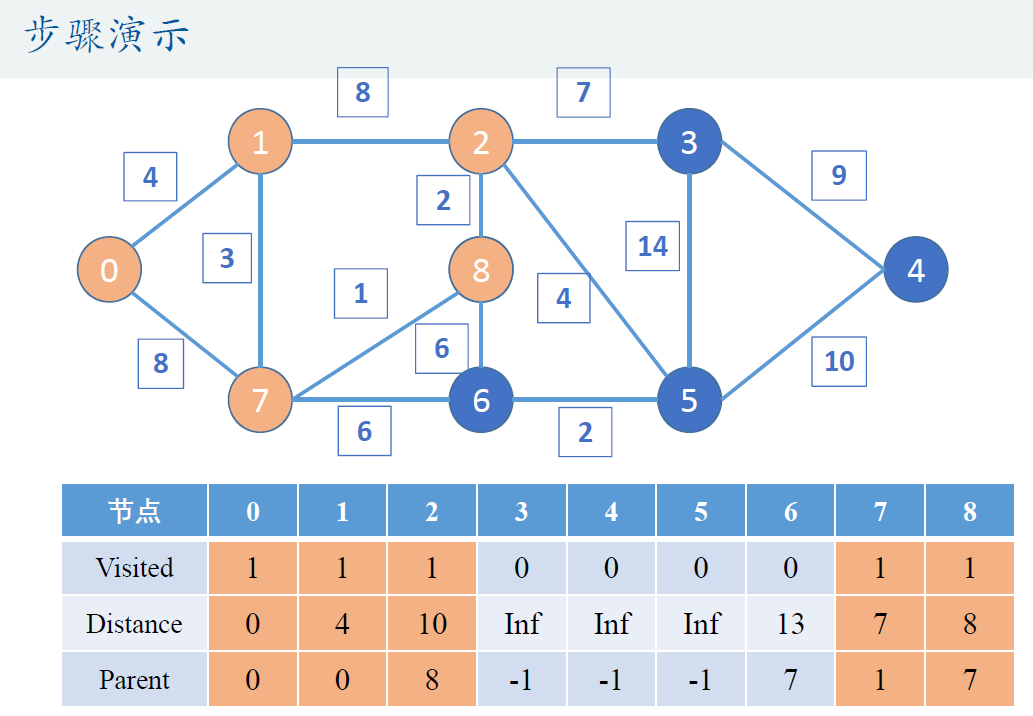
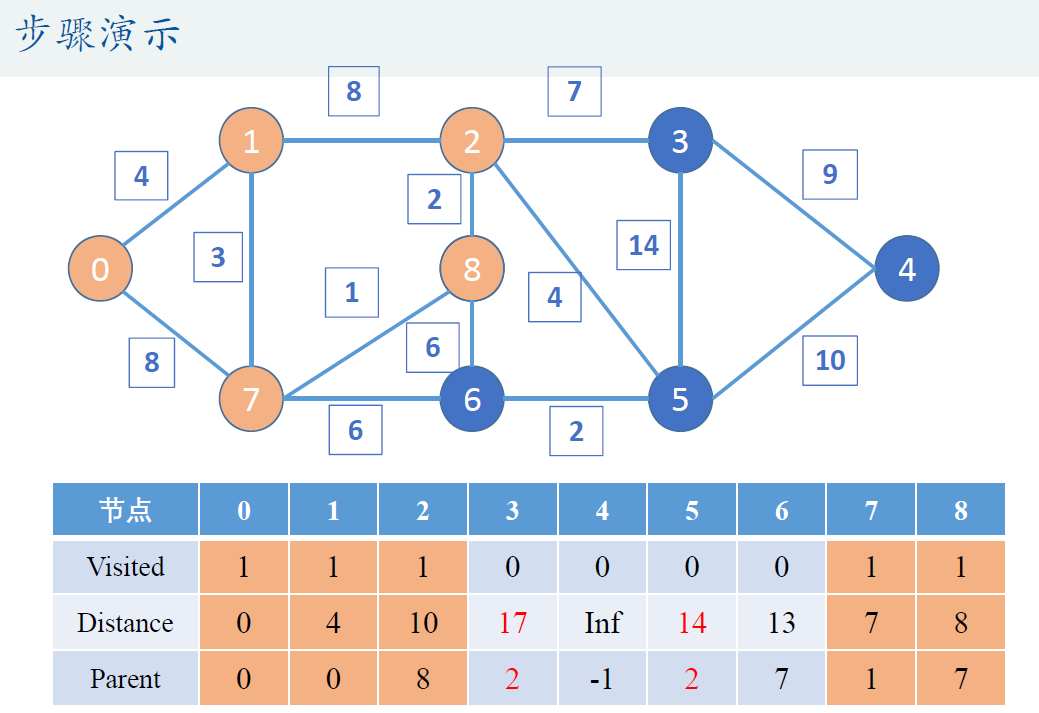
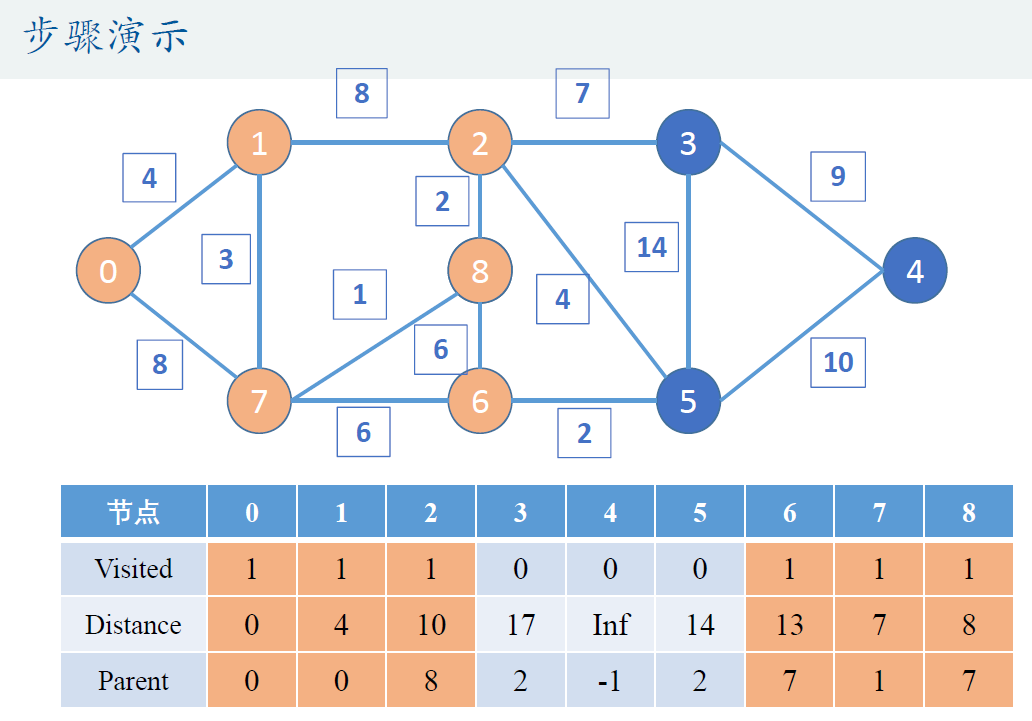
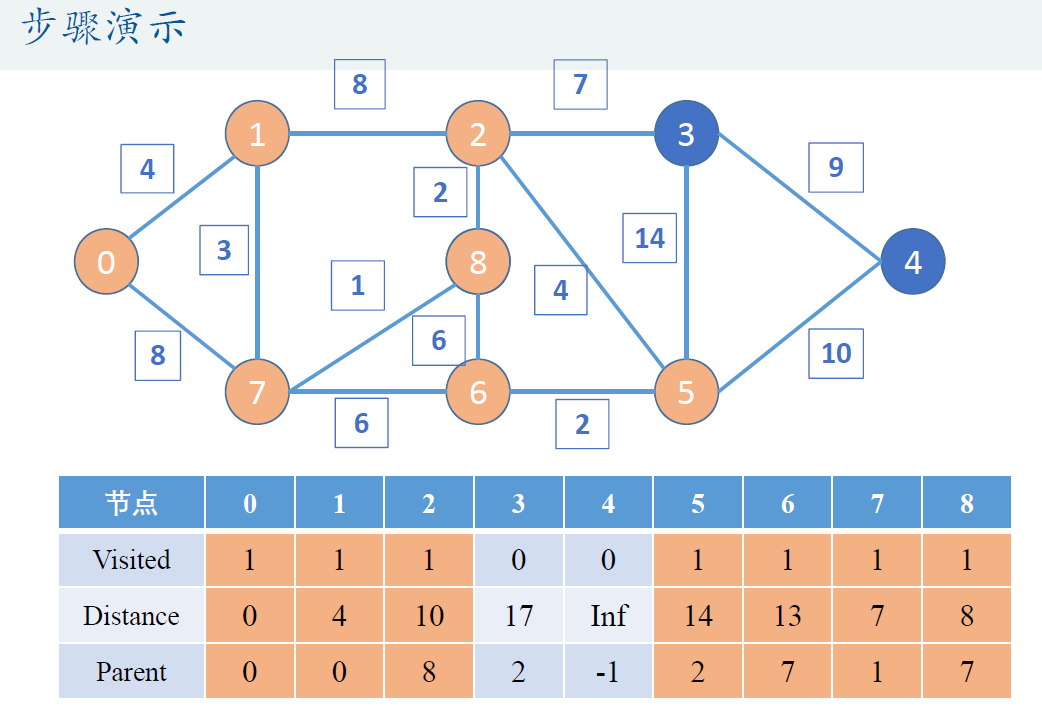
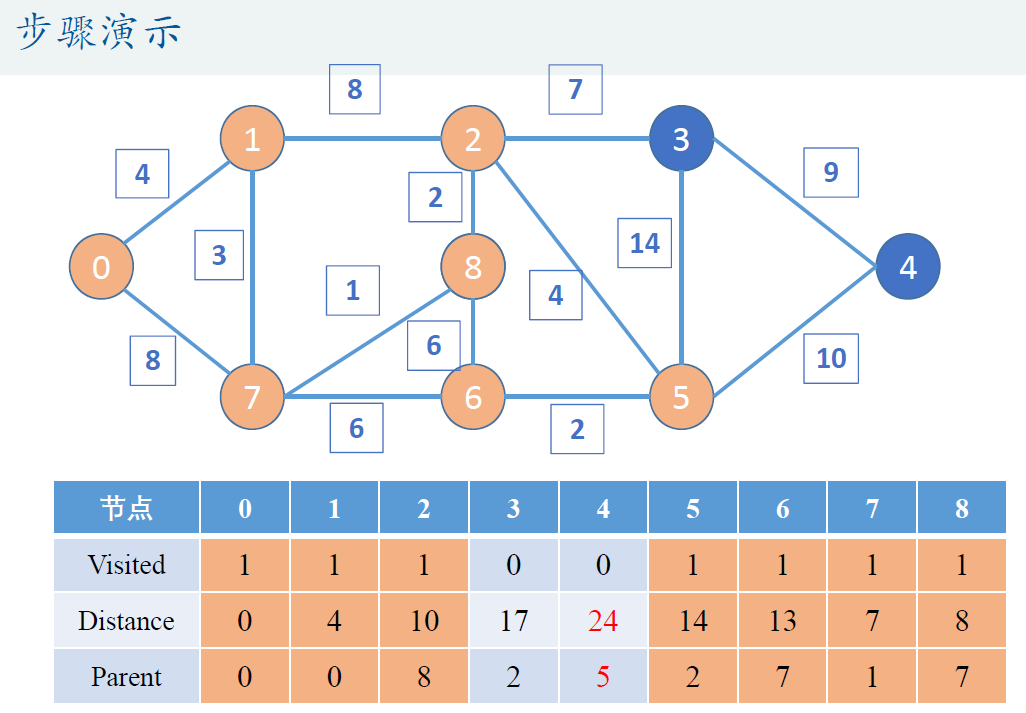
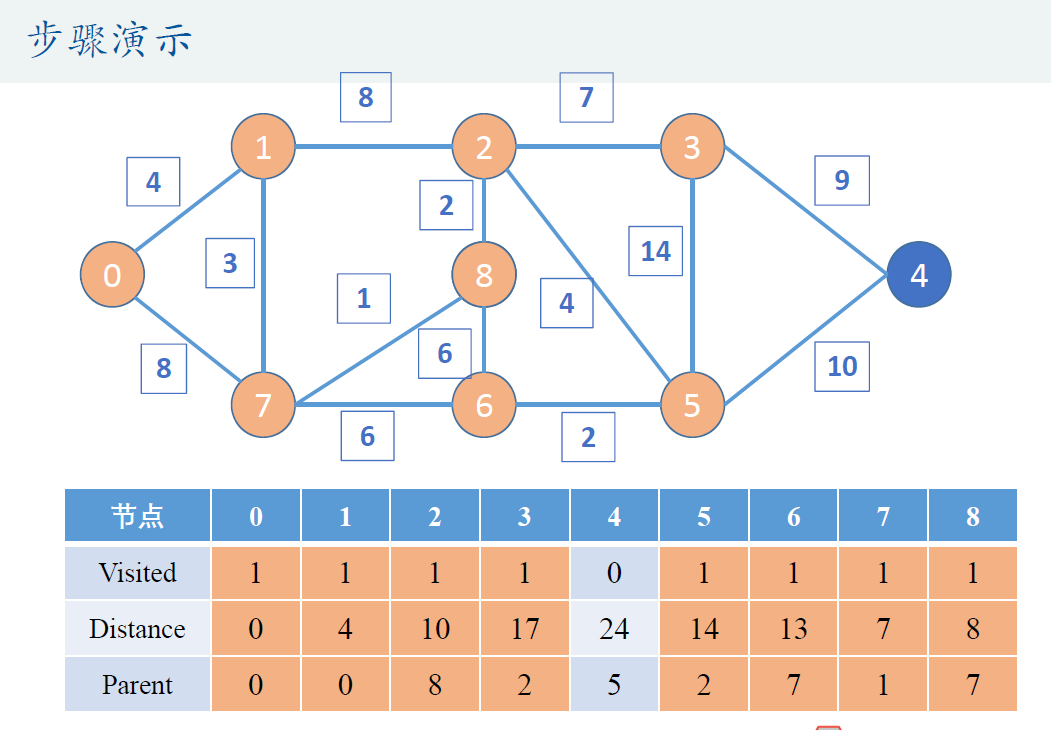
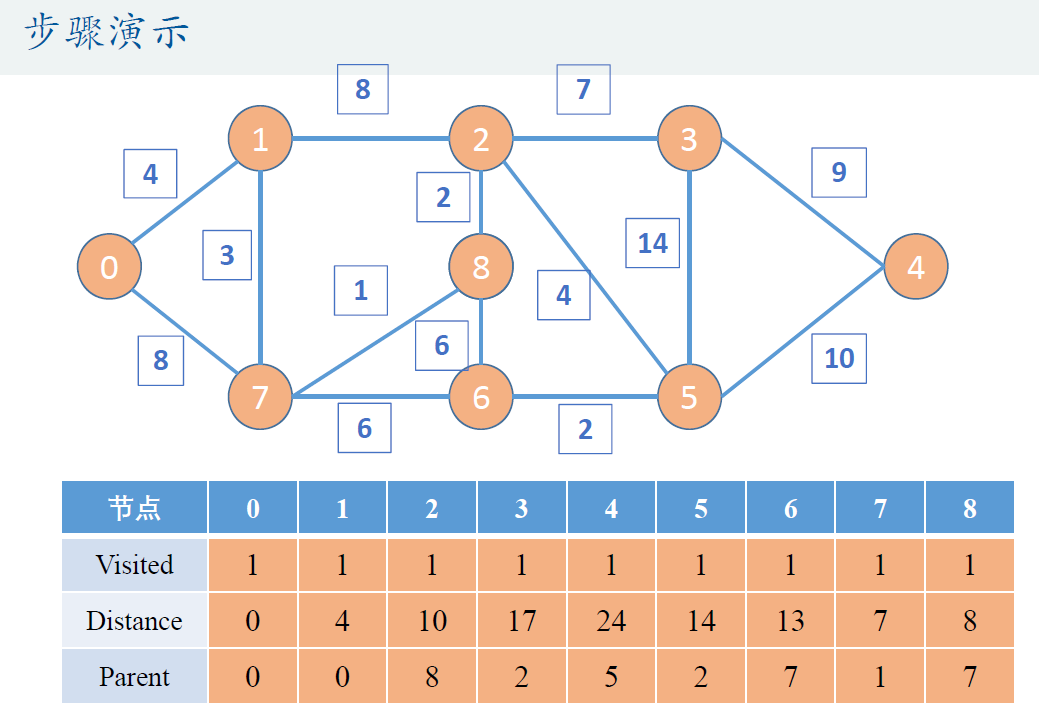
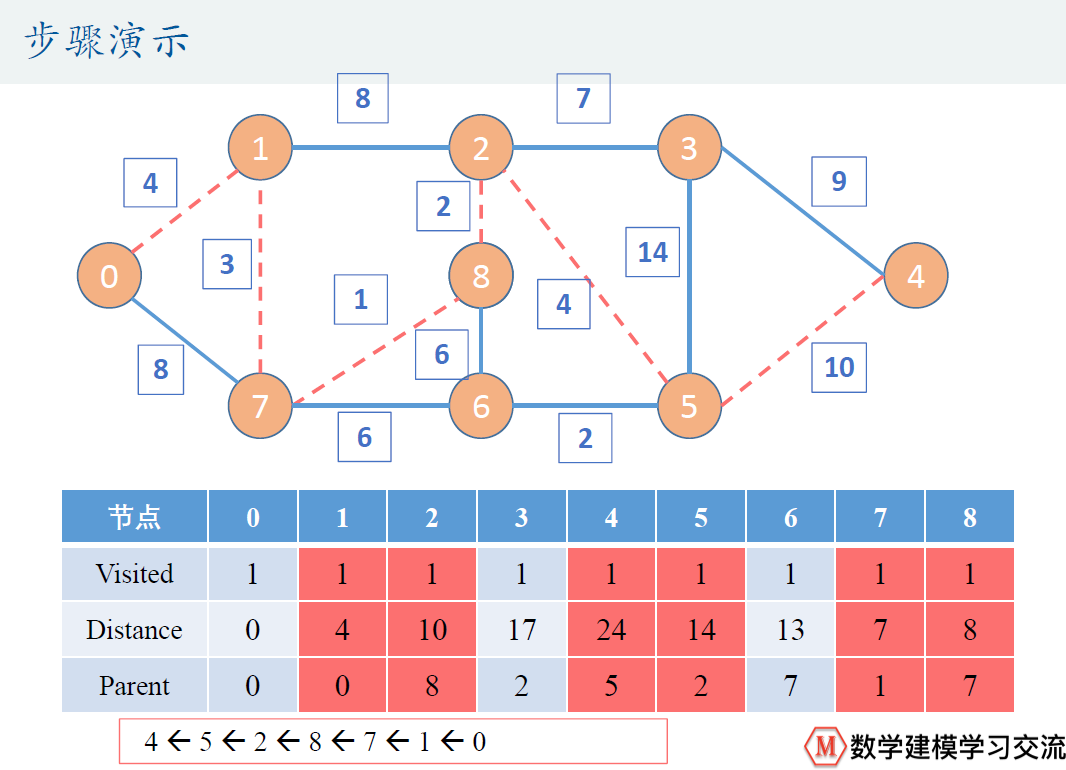
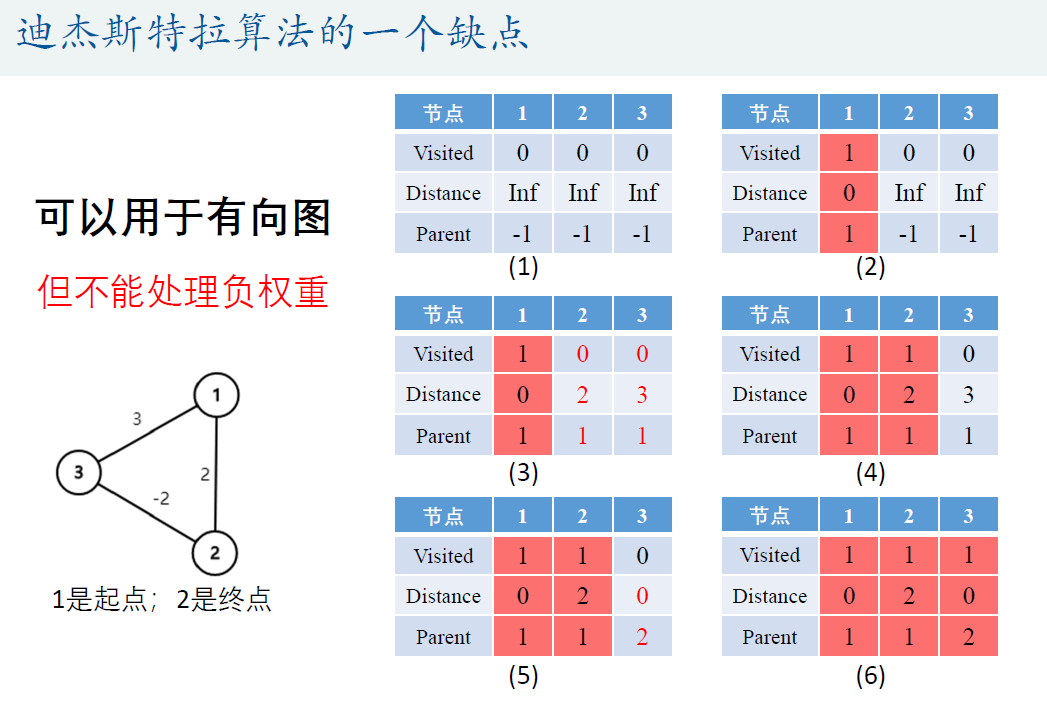
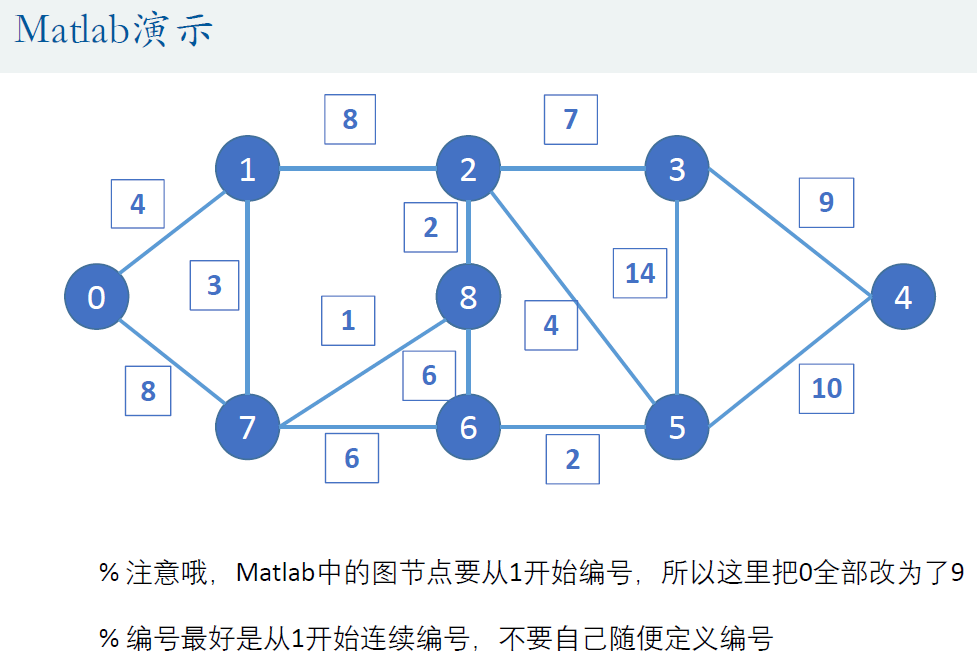
迪杰斯特拉算法代码

%% 注意：以下代码需要较新版本的matlab才能运行（最好是2016版本及以上哦）
% 如果运行出错请下载新版的matlab代码再运行

% 注意哦，Matlab中的图节点要从1开始编号，所以这里把0全部改为了9
% 编号最好是从1开始连续编号，不要自己随便定义编号
s = [9 9 1 1 2 2 2 7 7 6 6  5  5 4];
t = [1 7 7 2 8 3 5 8 6 8 5  3  4 3];
w = [4 8 3 8 2 7 4 1 6 6 2 14 10 9];
G = graph(s,t,w);
plot(G, 'EdgeLabel', G.Edges.Weight, 'linewidth', 2) 
set( gca, 'XTick', [], 'YTick', [] );  
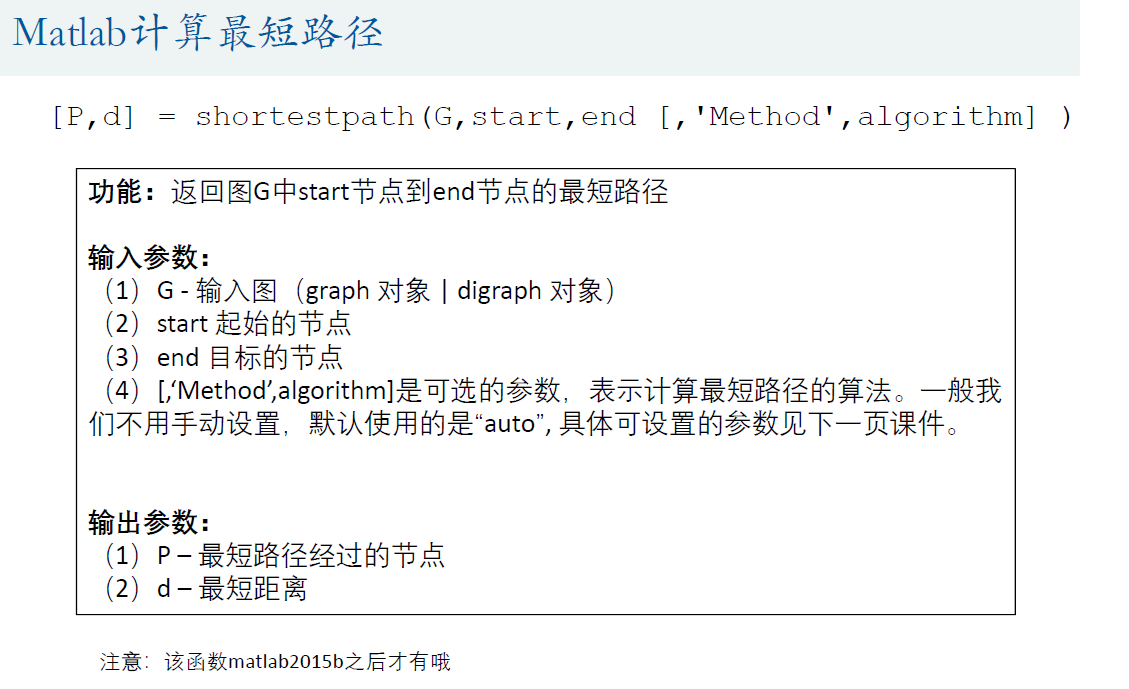
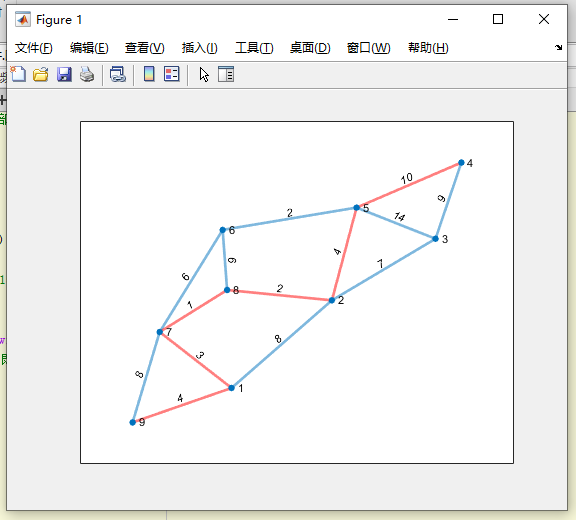
[P,d] = shortestpath(G, 9, 4)  %注意：该函数matlab2015b之后才有哦

% 在图中高亮我们的最短路径
myplot = plot(G, 'EdgeLabel', G.Edges.Weight, 'linewidth', 2);  %首先将图赋给一个变量
highlight(myplot, P, 'EdgeColor', 'r')   %对这个变量即我们刚刚绘制的图形进行高亮处理（给边加上r红色）

% 求出任意两点的最短路径矩阵
D = distances(G)   %注意：该函数matlab2015b之后才有哦
D(1,2)  % 1 -> 2的最短路径
D(9,4)  % 9 -> 4的最短路径

% 找出给定范围内的所有点  nearest(G,s,d)
% 返回图形 G 中与节点 s 的距离在 d 之内的所有节点
[nodeIDs,dist] = nearest(G, 2, 10)   %注意：该函数matlab2016a之后才有哦