236. Lowest Common Ancestor of a Binary Tree 二叉树的最低公共祖先

Given a binary tree, find the lowest common ancestor (LCA) of two given nodes in the tree.

According to the definition of LCA on Wikipedia: “The lowest common ancestor is defined between two nodes p and q as the lowest node in T that has both p and q as descendants (where we allow a node to be a descendant of itself).”

 

Example 1:

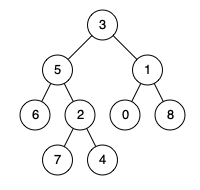
Input: root = [3,5,1,6,2,0,8,null,null,7,4], p = 5, q = 1
Output: 3
Explanation: The LCA of nodes 5 and 1 is 3.

Example 2:

Input: root = [3,5,1,6,2,0,8,null,null,7,4], p = 5, q = 4
Output: 5
Explanation: The LCA of nodes 5 and 4 is 5, since a node can be a descendant of itself according to the LCA definition.

Example 3:

Input: root = [1,2], p = 1, q = 2
Output: 1

复习时还不会的地方：不知道dc的left right为什么要在函数里面inside定义。
因为这就是递归啊！这个节点也有属于它的子节点，需要一直循环下去

 

root和p q有什么关系啊？感觉是三个独立的点，不太懂
思路：反正就是不停地递归，所以root.left定义一次，root.right定义一次。典型的DC。

 

class Solution {
    public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {
        //cc
        if (root == null || p == root || q == root)
            return root;
        
        //定义
        TreeNode left = lowestCommonAncestor(root.left, p, q);
        TreeNode right = lowestCommonAncestor(root.right, p, q);
        
        //分开讨论
        if (left != null && right != null) {
            return root;
        } else if (left == null) {
            return right;
        } else {
            return left;
        } 
                
    }
}