算法(第四版)C# 习题题解——1.1
写在前面
整个项目都托管在了 Github 上:https://github.com/ikesnowy/Algorithms-4th-Edition-in-Csharp
善用 Ctrl + F 查找题目。
本节你可能会需要的两个测试数据文件:
largeW: http://algs4.cs.princeton.edu/11model/largeW.txt
largeT: http://algs4.cs.princeton.edu/11model/largeT.txt
习题 & 题解
练习(1.1.1~1.1.25)
1.1.1
解答
a.7
b.1562500.0015625
c.True
代码
static void Main(string[] args) { int a = (0 + 15) / 2; double b = Math.Pow(2.0, -6) * 100000000.1; //Math.Pow(double x, double y) 求x的y次方 bool c = true && false || true && true; //Console.WriteLine 向控制台窗口输出一行 Console.WriteLine($"a.{a}"); Console.WriteLine($"b.{b}"); Console.WriteLine($"c.{c}"); }
1.1.2
解答
Name Type Value
a System.Double 1.618
b System.Double 10
c System.Boolean True
d System.String 33
代码
static void Main(string[] args) { //var 变量名 = 初始值 根据初始值自动判断变量类型 var a = (1 + 2.236) / 2; var b = 1 + 2 + 3 + 4.0; var c = 4.1 >= 4; var d = 1 + 2 + "3"; //Console.WriteLine 向控制台输出一行 //变量名.GetType() 返回变量类型 //Type.ToString() 将类型名转换为字符串 Console.WriteLine("\tName\tType \tValue"); Console.WriteLine($"\ta\t{a.GetType().ToString()}\t{a}"); Console.WriteLine($"\tb\t{b.GetType().ToString()}\t{b}"); Console.WriteLine($"\tc\t{c.GetType().ToString()}\t{c}"); Console.WriteLine($"\td\t{d.GetType().ToString()}\t{d}"); }
1.1.3
解答
简单的 if 判断即可
代码
static void Main(string[] args) { //Console.ReadLine() 从控制台读入一整行(返回int) //string.Split(char) 根据提供的分隔符将字符串分割,返回字符串数组 //Int32.Parse(string) 将字符串转换为相应的整型数据 string input = Console.ReadLine(); int a = Int32.Parse(input.Split(' ')[0]); int b = Int32.Parse(input.Split(' ')[1]); int c = Int32.Parse(input.Split(' ')[2]); //Console.WriteLine() 向控制台输出一行 if (a == b && b == c) { Console.WriteLine("equal"); } else { Console.WriteLine("not equal"); } }
1.1.4
解答
a. if 后跟 then 的语法不能在 C# 中使用。
b. if 后的判断语句需要在括号内。
c. 正确,只有一条语句时大括号可以省略。
d. c = 0 后缺少分号。
代码
static void Main(string[] args) { int a = 1; int b = 2; int c = 0; //if (a > b) then c = 0; //if 后不能跟 then //if a > b { c = 0; } //if后必须跟括号 if (a > b) c = 0; //正确 //if (a > b) c = 0 else b = 0; //c = 0后缺少分号 }
1.1.5
解答
比较简单,直接判断即可。
代码
static void Main(string[] args) { //修改这两个值进行测试 double x = 0.05; double y = 0.01; if (x > 0 && x < 1 && y > 0 && y < 1) { Console.WriteLine("true"); } else { Console.WriteLine("false"); } }
1.1.6
解答
输出斐波那契数列。
将书中的代码直接实现即可。
代码
//输出斐波那契数列 static void Main(string[] args) { int f = 0; int g = 1; for (int i = 0; i <= 15; i++) { //Console.WriteLine与StdOut.println功能相同 //实现向控制台输出一行 Console.WriteLine(f); f = f + g; g = f - g; } }
1.1.7
解答
同上题,直接实现即可。
a
3.00009
double计算存在误差,并不精确。
b
499500
1000 + 999 + 998……
c
10000
1000 * 10,外层循环的结束条件为 2i > 1000。
代码
private static void a() { Console.WriteLine("a"); double t = 9.0; while (Math.Abs(t - 9.0 / t) > .001) { t = (9.0 / t + t) / 2.0; } Console.Write($"{t:N5}\n");//:N5代表保留5位小数,同理可使用N1、N2…… } private static void b() { Console.WriteLine("\nb"); int sum = 0; for (int i = 1; i < 1000; i++) { for (int j = 0; j < i; j++) { sum++; } } Console.WriteLine(sum); } private static void c() { Console.WriteLine("\nc"); int sum = 0; for (int i = 1; i < 1000; i *= 2) { for (int j = 0; j < 1000; j++) { sum++; } } Console.WriteLine(sum); } static void Main(string[] args) { //a double 计算存在误差 a(); //b 1000+999+998…… b(); //c 由于2^10 = 1024 > 1000,最终sum = 1000 * 10 = 10000 c(); }
1.1.8
解答
b
197
e
代码
static void Main(string[] args) { Console.WriteLine('b'); Console.WriteLine('b' + 'c'); //char 被隐式转为为 int 类型,取 ascii 码 Console.WriteLine((char)('a' + 4)); //强制转换后,ascii 码被转换为相应的字符 }
1.1.9
解答
有两种方法,要么直接调用库函数,要么用书中给出的代码转换。
代码
static void Main(string[] args) { int N = 4; //1.直接转换 Convert.ToString(int, int) 第一个为要转换的数,第二个为要转换的进制 Console.WriteLine($"{Convert.ToString(N, 2)}"); //2.转换为二进制数 string s = ""; for (int n = N; n > 0; n /= 2) { s = (n % 2) + s; } Console.WriteLine(s); }
1.1.10
解答
变量使用前需要先赋值。
代码
static void Main(string[] args) { int[] a; for (int i = 0; i < 10; i++) { a[i] = i * i; //不允许使用未赋值的局部变量 } }
1.1.11
解答
注意,二维数组 bool[M, N] 代表 M 行 N 列的布尔数组。
使用二重循环即可实现。
输出使用制表符 ’\t’ 作为分隔。
代码
static void PrintArray2D(bool[,] array) { int rows = array.GetLength(0);//获取行数 int columns = array.GetLength(1);//获取列数 //输出列号 for (int i = 0; i < columns; i++) { Console.Write($"\t{i + 1}"); } Console.Write("\n"); for (int i = 0; i < rows; i++) { //输出行号 Console.Write($"{i + 1}"); for (int j = 0; j < columns; j++) { if (array[i, j]) { Console.Write($"\t*"); } else { Console.Write($"\t "); } } Console.Write("\n"); } }
1.1.12
解答
第一个循环初始化数组{9, 8, 7, 6, 5, 4, 3, 2, 1, 0}
第二个循环用相应位置的值作为下标取值,例如:a[0] = a[a[0]] = a[9] = 0
最后结果为:0,1,2,3,4,4,3,2,1,0
代码
static void Main(string[] args) { int[] a = new int[10]; for (int i = 0; i < 10; i++) { a[i] = 9 - i; } //a[10] = {9, 8, 7, 6, 5, 4, 3, 2, 1, 0} for (int i = 0; i < 10; i++) { a[i] = a[a[i]]; } //a[0] = a[9] = 0; a[1] = a[8] = 1; a[2] = a[7] = 2;...... for (int i = 0; i < 10; i++) { Console.WriteLine(a[i]); } }
1.1.13
解答
转置输出只需要在二重循环的时候将行、列输出顺序取反即可。
代码
static void Main(string[] args) { int M = 2; int N = 3; int[,] array = new int[M, N]; //新建一个二维数组 for (int i = 0; i < M; i++) { for (int j = 0; j < N; j++) { array[i, j] = i + j; } } Console.WriteLine("Origin"); PrintArray2D(array, M, N); Console.WriteLine("Transposed"); PrintArrayTranspose2D(array, M, N); } //转置输出 private static void PrintArrayTranspose2D(int[,] array, int rows, int columns) { //交换行、列输出顺序 for (int i = 0; i < columns; i++) { for (int j = 0; j < rows; j++) { Console.Write($"\t{array[j, i]}"); } Console.Write("\n"); } } //正常输出 private static void PrintArray2D(int[,] array, int rows, int columns) { for (int i = 0; i < rows; i++) { for (int j = 0; j < columns; j++) { Console.Write($"\t{array[i, j]}"); } Console.Write("\n"); } }
1.1.14
解答
简单使用 log 的定义逼近即可。
代码
static void Main(string[] args) { int N = 9; Console.WriteLine($"{ lg(N)}"); } //利用循环逼近 N,得到 log2(N) 的值 static int lg(int N) { int baseNumber = 2; int pow = 1; int sum = 2; for (pow = 1; sum < N; ++pow) { sum *= baseNumber; } return pow - 1; }
1.1.15
解答
利用二重循环,查找每个值在数组中出现的次数。
代码
static void Main(string[] args) { int[] a = new int[10]; int M = 10; for (int i = 0; i < 10; ++i) { a[i] = i; } int[] result = Histogram(a, M); Console.WriteLine($"a.length: {a.Length}"); Console.WriteLine($"sum of result array: {result.Sum()}"); } static int[] Histogram(int[] a, int M) { int[] result = new int[M]; for (int i = 0; i < M; ++i) { //初始化 result[i] = 0; //遍历数组,计算数组中值为 i 的元素个数 for (int j = 0; j < a.Length; ++j) { if (a[j] == i) //值为 i 的元素 { result[i]++; } } } return result; }
1.1.16
解答
填入代码测试即可。
用字符串拼接的方式展示递归。
类似于这个:
代码
static void Main(string[] args) { Console.WriteLine($"{exR1(6)}"); } //exR1(6) = //exR1(3) + 6 + exR1(4) + 6 //exR1(0) + 3 + exR1(1) + 3 + 6 + exR1(4) + 6 //"" + 3 + exR1(-2) + 1 + exR1(-1) + 1 + 3 + 6 + exR1(4) + 6 //"" + 3 + "" + 1 + "" + 1 + 3 + 6 + exR1(4) + 6 //"31136" + exR1(4) + 6 //...... public static string exR1(int n) { if (n <= 0) { return ""; } return exR1(n - 3) + n + exR1(n - 2) + n; }
1.1.17
解答
书中已经给出了解释。
递归时结束条件必须放在递归语句的前面,否则会不断展开而无法结束。
代码
static void Main(string[] args) { Console.WriteLine($"{exR2(6)}");//抛出 StackOverflow Exception } public static string exR2(int n) { string s = exR2(n - 3) + n + exR2(n - 2) + n;//运行到 exR2 即展开,不会再运行下一句 if (n <= 0) return ""; return s; }
1.1.18
解答
其实就是一种快速乘法的实现,换成乘号之后就变成了快速乘幂。
例如对于乘法 2 * 4,可以用 2 + 2 + 2 + 2 做四次加法计算;也可以变为 (2 + 2) * 2 = (2 + 2) + (2 + 2) 的形式,用两次加法就可以完成(先计算 2 + 2 的值,再计算 4 + 4 的值)。
同理对于乘幂 28,既可以用 2 * 2 * 2 * 2 * 2 * 2 * 2 * 2 做 8 次乘法,也可以只用三次乘法就计算出来:
22 = 2 * 2
24 = 22 * 22
28 = 24 * 24
这样时间复杂度就从 O(n) 变为了 O(log n)。
代码
static void Main(string[] args) { Console.WriteLine($"mystery(2, 25): {mystery(2, 25)}"); Console.WriteLine($"mystery(3, 11): {mystery(3, 11)}"); Console.WriteLine($"mysteryChanged(2, 8): {mysteryChanged(2, 8)}"); Console.WriteLine($"mysteryChanged(3, 2): {mysteryChanged(3, 2)}"); } //mystery(a, b) = a * b //利用等式:a * b = 2a * b/2 = (2a * (b-1) / 2) + a //示例: //mystery(2, 25) = //mystery(2 + 2, 12) + 2 = //mystery(4 + 4, 6) + 2 = //mystery(8 + 8, 3) = //mystery(16 + 16, 1) + 16 + 2 = //mystery(32 + 32, 0) + 32 + 16 + 2 = //0 + 32 + 16 + 2 = //50 public static int mystery(int a, int b) { if (b == 0) return 0; if (b % 2 == 0) return mystery(a + a, b / 2); return mystery(a + a, b / 2) + a; } //mysteryChanged(a, b) = a ^ b //同理(乘方与乘法,乘法与加法之间具有类似的性质) //a ^ b = (a ^ 2) ^ (b / 2) = (a ^ 2) ^ ((b - 1) / 2) * a public static int mysteryChanged(int a, int b) { if (b == 0) return 1; if (b % 2 == 0) return mysteryChanged(a * a, b / 2); return mysteryChanged(a * a, b / 2) * a; }
1.1.19
解答
普通的递归算法效率很低,原因是越到后面重复运算的数目越多。
比如:
F(2) = F(1) + F(0)
F(3) = F(2) + F(1) = F(1) + F(1) + F(0)
可以看到 F(1) 被重复计算了两次。
改进的方式是将每次运算的结果保存在数组中,之后计算过的数据直接从数组中提取。
代码
class Fibnacci { //long 类型不够大,换成 UINT64 类型 //用于保存计算结果的数组,UInt64? 代表可以赋值为普通 UInt64 类型的值以及 null 值 private static UInt64?[] fibnacciResults = new UInt64?[100]; static void Main(string[] args) { /* * 测试环境 * * Surface Pro3 i7 * i7 4650U + 8G * */ Stopwatch timer = Stopwatch.StartNew(); for (int N = 0; N < 100; ++N) { //书本中的代码,非常慢,1小时后 N = 50 //Console.WriteLine($"{N} {F(N)}"); //利用已知结果加速 //全部计算完毕耗时 84ms Console.WriteLine($"{N} {BetterF(N)}"); } Console.WriteLine($"{timer.ElapsedMilliseconds} ms"); } //书中提供的代码 public static UInt64 F(int N) { if (N == 0) return 0; if (N == 1) return 1; return F(N - 1) + F(N - 2); } //更好的实现,将已经计算的结果保存,不必重复计算 public static UInt64? BetterF(int N) { if (N == 0) return 0; if (N == 1) return 1; if (fibnacciResults[N] != null) //如果已经计算过则直接读取已知值 { return fibnacciResults[N]; } else { fibnacciResults[N] = BetterF(N - 1) + BetterF(N - 2); return fibnacciResults[N]; } } }
1.1.20
解答
根据对数的性质可以得到:
ln(N!) = ln(N) + ln(N – 1) + ln(N – 2)…
代码
static void Main(string[] args) { int N = 4; Console.WriteLine($"{factorialLn(N)}"); } //ln(N!) = //ln(N * (N - 1) * ... * 1) = //ln(N) + ln((N - 1)!) public static double factorialLn(int N) { if (N == 1) { return 0; } return Math.Log(N) + factorialLn(N - 1); }
1.1.21
解答
实现上没什么难度,打印表格的部分可以参考之前打印二位布尔数组的方法。
注意整型数据之间相除得到的仍然是整型,小数部分会直接舍去,例如 2 / 3 = 0。
代码
static void Main(string[] args) { int columns = 2; int rows = int.Parse(Console.ReadLine()); //行号 string[] names = new string[rows]; //姓名 int[,] array = new int[rows, columns]; //输入的两个整数 double[] results = new double[rows]; //计算结果 for (int i = 0; i < rows; ++i) { string temp = Console.ReadLine(); names[i] = temp.Split(' ')[0]; for (int j = 0; j < columns; ++j) { array[i, j] = int.Parse(temp.Split(' ')[j + 1]); } results[i] = (double)array[i, 0] / array[i, 1]; } PrintArray2D(names, array, results); } static void PrintArray2D(string[] names, int[,] array, double[] results) { int rows = array.GetLength(0);//获取行数 int columns = array.GetLength(1);//获取列数 for (int i = 0; i < rows; i++) { Console.Write($"\t{names[i]}"); for (int j = 0; j < columns - 1; j++) { Console.Write($"\t{array[i, j]}"); } Console.Write($"\t{array[i, columns - 1]}"); Console.Write($"\t{results[i]:N3}"); //变量名:N3 保留三位小数 Console.Write("\n"); } }
1.1.22
解答
按照书中的提示增加一个保存深度的参数。
代码
class BinarySearch { static void Main(string[] args) { int[] array = new int[10] { 1, 2, 3, 4, 5, 6, 7, 8, 9, 10 }; rank(9, array); } //重载方法,用于启动二分查找 public static int rank(int key, int[] a) { return rank(key, a, 0, a.Length - 1, 1); } //二分查找 public static int rank(int key, int[] a, int lo, int hi, int number) { for (int i = 0; i < number; ++i) { Console.Write(" "); } Console.WriteLine($"{number}: {lo} {hi}"); if (lo > hi) { return -1; } int mid = lo + (hi - lo) / 2; if (key < a[mid]) { return rank(key, a, lo, mid - 1, number + 1); } else if (key > a[mid]) { return rank(key, a, mid + 1, hi, number + 1); } else { return mid; } } }
1.1.23
解答
在主函数里做一下判断就可以了,加号则输出所有找不到的值,减号则相反。
代码
static void Main(string[] args) { //从largeW.txt中读取数据 string[] whiteList = File.ReadAllLines("largeW.txt"); int[] WhiteList = new int[whiteList.Length]; for (int i = 0; i < whiteList.Length; ++i) { WhiteList[i] = int.Parse(whiteList[i]); } Array.Sort<int>(WhiteList); Console.WriteLine("Type the numbers you want to query: "); //输入样例:5 824524 478510 387221 string input = Console.ReadLine(); int[] Query = new int[input.Split(' ').Length]; for (int i = 0; i < Query.Length; ++i) { Query[i] = int.Parse(input.Split(' ')[i]); } Console.WriteLine("Type '+' to get the numbers that not in the whitelist," + "'-' to get the numbers that in the whitelist."); char operation = Console.ReadLine()[0]; foreach (int n in Query) { if (rank(n, WhiteList) == -1) { if (operation == '+') { Console.WriteLine(n); } } else if (operation == '-') { Console.WriteLine(n); } } } //重载方法,用于启动二分查找 public static int rank(int key, int[] a) { return rank(key, a, 0, a.Length - 1); } //二分查找 public static int rank(int key, int[] a, int lo, int hi) { if (lo > hi) { return -1; } int mid = lo + (hi - lo) / 2; if (key < a[mid]) { return rank(key, a, lo, mid - 1); } else if (key > a[mid]) { return rank(key, a, mid + 1, hi); } else { return mid; } }
1.1.24
解答
在书本中 GCD 的基础上,在函数开始时增加一条输出语句即可。
代码
static void Main(string[] args) { GCD(105, 24); Console.WriteLine(); GCD(111111, 1234567); } public static int GCD(int a, int b) { Console.WriteLine($"{a} {b}"); if (b == 0) { return a; } return GCD(b, a % b); }
1.1.25
解答
证明见代码。
也可以访问维基百科:辗转相除法
代码
namespace _1._1._25 { /* * 1.1.25 * * 用数学归纳法证明欧几里得算法能够计算出任意一对非负整数 p 和 q 的最大公约数 * */ class Program { static void Main(string[] args) { /* 证明: * * 已知: a, b 皆为正整数,且 a > b。g 是 a、b 的最大公约数 * 设 r0 = a % b, rk = rk-2 % rk-1 * 那么有 gcd(a, b) = gcd(b, r0) = gcd(r0, r1)... = gcd(rn-1, rn) * 且 rn = 0 (此时算法终止) * * 由于 rn-2 = qn * rn - 1 + rn = qn * rn-1 (qn = [rn-2 / rn-1] []代表向下取整) * 可得 rn-2 能被 rn-1 整除 * 则 * rn-3 = qn-1 * rn-2 + rn-1 * = qn-1 * (qn * rn-1) + rn-1 (代入 rn-2 = qn * rn-1) * = qn-1 * qn * rn-1 + rn-1 * = (qn-1 * qn + 1) * rn-1 * 可得 rn-3 也能被 rn-1 整除 * 以此类推,rn-1 可以整除 a 和 b,即 rn-1 是 a 和 b 的公约数 * 则 rn-1 <= g * * 因为 g 是 a、b 的最大公约数,由性质可得: * a = mg, b = ng (m、n 是自然数) * * r0 * = a % b * = a - q0 * b (q0 = [a / b] []代表向下取整) * = mg - q0 * ng (代入 34 行的结论) * = (m - q0 * n)g * * 可得 r0 能被 g 整除 * 同理 r1, r2, r3, ..., rn-1 都可以被 g 整除 * 因此 g <= rn-1 * * 综合 31 行和 44 行的结论可得 rn-1 = g * * 证明完毕 */ } static int gcd(int p, int q) { if (q == 0) { return p; } int r = p % q; return gcd(q, r); } } }
提高题(1.1.26~1.1.34)
1.1.26
解答
见代码部分。
代码
static void Main(string[] args) { int a = 3; int b = 2; int c = 1; int t = 0; if (a > b) { t = a; a = b; b = t; } //如果 a > b,那么 a, b 交换,保证b >= a if (a > c) { t = a; a = c; c = t; } //如果 b >= a > c,那么 a, c 交换,保证 c >= a if (b > c) { t = b; b = c; c = t; } //如果 b > c >= a,那么 b, c 交换,保证 c >= b Console.WriteLine($"{a} {b} {c}"); //最终结果为 c >= b >= a,保证升序排列 }
1.1.27
解答
与之前的斐波那契数列类似,都是重复计算的问题。
7751次。
代码
class Program { static int BinomialCalled = 0; //计算递归调用次数 static double?[,] BinomialCache; //保存计算结果的数组 static void Main(string[] args) { BinomialCache = new double?[101, 51]; Console.WriteLine(Binomial(100, 50, 0.25)); Console.WriteLine(BinomialCalled); } public static double? Binomial(int N, int k, double p) { BinomialCalled++; if (N == 0 && k == 0) return 1.0; if (N < 0 || k < 0) return 0.0; if (BinomialCache[N, k] != null) { return BinomialCache[N, k]; } else { BinomialCache[N, k] = (1.0 - p) * Binomial(N - 1, k, p) + p * Binomial(N - 1, k - 1, p); return BinomialCache[N, k]; } } }
1.1.28
解答
实现方法有很多,这里是使用一个 HashSet 做中转,删除所有的重复元素。
也可以使用 Linq 里的 Distinct() 方法,
也可以排序后直接遍历一遍,遇到相同的就删除,遇到不同的就保存起来用于之后的比较。
代码
static void Main(string[] args) { //从largeW.txt中读取数据 //用 HashSet 的不可重复性去除重复 HashSet<string> h = new HashSet<string>(File.ReadAllLines("largeW.txt")); string[] whiteList = new string[h.Count]; h.CopyTo(whiteList); int[] WhiteList = new int[whiteList.Length]; for (int i = 0; i < whiteList.Length; ++i) { WhiteList[i] = int.Parse(whiteList[i]); } Array.Sort<int>(WhiteList); Console.WriteLine("Type the numbers you want to query: "); //输入样例:5 824524 478510 387221 string input = Console.ReadLine(); int[] Query = new int[input.Split(' ').Length]; for (int i = 0; i < Query.Length; ++i) { Query[i] = int.Parse(input.Split(' ')[i]); } Console.WriteLine("Irrelevant:"); foreach (int n in Query) { if (rank(n, WhiteList) == -1) { Console.WriteLine(n); } } } //重载方法,用于启动二分查找 public static int rank(int key, int[] a) { return rank(key, a, 0, a.Length - 1); } //二分查找 public static int rank(int key, int[] a, int lo, int hi) { if (lo > hi) { return -1; } int mid = lo + (hi - lo) / 2; if (key < a[mid]) { return rank(key, a, lo, mid - 1); } else if (key > a[mid]) { return rank(key, a, mid + 1, hi); } else { return mid; } }
1.1.29
解答
查找小于指定值的元素数量可以多次使用二分查找实现。
例如:
序号:0 1 2 3 4 5 6 7 8
元素:1 2 2 2 2 2 2 2 3
二分查找返回 4
再次在 0~3 之间查找
二分查找返回 1
再次在 0~1 之间查找
二分查找返回 -1,没有指定值了
因此小于该值的元素数量就是 1 – 0 = 1 个
用同样的方法可以找到大于指定值的元素个数,从总数中减去这两个数值就是等于指定值的元素数量。
代码
static void Main(string[] args) { int[] WhiteList = new int[] { 1, 1, 2, 2, 3, 3, 4, 4, 5, 5, 6, 6 }; Array.Sort<int>(WhiteList); Console.WriteLine("Type the numbers you want to query: "); string input = Console.ReadLine(); int[] Query = new int[input.Split(' ').Length]; for (int i = 0; i < Query.Length; ++i) { Query[i] = int.Parse(input.Split(' ')[i]); } Console.WriteLine("Result:"); foreach (int n in Query) { int less = rank(n, WhiteList); int equal = count(n, WhiteList); Console.WriteLine($"Less: {less} Equal: {equal}"); } } //返回数组中相等元素的个数 public static int count(int key, int[] a) { int lowerBound = rank(key, a); int upperBound = lowerBound; if (lowerBound == -1) return 0; int result = 0; while (true) { result = rank(key, a, upperBound + 1, a.Length - 1); if (result == -1) break; if (result > upperBound) { upperBound = result; } } return upperBound - lowerBound + 1; } //返回数组中小于该数的数字个数 public static int rank(int key, int[] a) { int mid = rank(key, a, 0, a.Length - 1); if (mid == -1) return 0; int result = mid; while (true) { result = rank(key, a, 0, mid - 1); if (result == -1) break; if (result < mid) mid = result; } return mid; } //二分查找 public static int rank(int key, int[] a, int lo, int hi) { if (lo > hi) { return -1; } int mid = lo + (hi - lo) / 2; if (key < a[mid]) { return rank(key, a, lo, mid - 1); } else if (key > a[mid]) { return rank(key, a, mid + 1, hi); } else { return mid; } } }
1.1.30
解答
互质可以用之前的 GCD 最大公因数算法判断,如果最大公因数是 1 则两数互质。
代码
//互质 = 最大公约数为 1 = gcd(i, j) == 1 static void Main(string[] args) { int N = int.Parse(Console.ReadLine()); bool[,] a = new bool[N, N]; for (int i = 0; i < N; ++i) { for (int j = 0; j < N; ++j) { a[i, j] = (gcd(i, j) == 1); } } PrintArray2D(a, N, N); } static int gcd(int a, int b) { if (b == 0) return a; return gcd(b, a % b); } private static void PrintArray2D(bool[,] array, int rows, int columns) { for (int i = 0; i < rows; i++) { for (int j = 0; j < columns; j++) { Console.Write($"\t{array[i, j]}"); } Console.Write("\n"); } } }
1.1.31
解答
概率的实现方法:
例如概率是 60 %,就在 [0, 100) 之间随机一个值,小于等于 60 则执行操作,反之不执行。
需要更精确的情况可以增大随机的范围,例如 [0, 1000)。
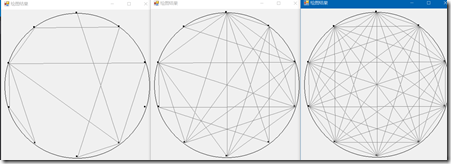
绘图结果:
N = 10,p = 0.2, 0.5, 1
完整项目可以到 Github 上下载。
代码(绘图部分)
/// <summary> /// 主绘图函数 /// </summary> /// <param name="N">点的总数目</param> /// <param name="p">每对点之间连接的概率</param> public static void StartDrawing(int N, double p) { int pointSize = 5;//每个点绘制的大小 int precious = 1000;//概率判断的精度 //新建一个绘图窗口 Form2 DrawPad = new Form2(); //显示绘图窗口 DrawPad.Show(); //新建画布 Graphics graphics = DrawPad.CreateGraphics(); //建立绘图区域(矩形) Rectangle rect = new Rectangle(10, 10, 400, 400); //画圆 graphics.DrawEllipse(Pens.Black, rect); //计算旋转角度 double rotateDgree = 360.0 / N; //计算点的坐标 Point Center = new Point(rect.Top + rect.Height / 2, rect.Top + rect.Height / 2); Point[] points = new Point[N]; points[0].X = rect.Left + rect.Width / 2; points[0].Y = rect.Top; for (int i = 1; i < N; ++i) { points[i] = Rotate(Center, points[i - 1], rotateDgree); } //绘制点 foreach (Point point in points) { graphics.FillEllipse(Brushes.Black, point.X - pointSize, point.Y - pointSize, pointSize, pointSize); } //按照概率绘制直线 Random random = new Random(); for (int i = 0; i < N; ++i) { for (int j = i + 1; j < N; ++j) { //举例:输入概率为 0.6,精度为 1000 //在 0~1000 范围内等概率取值,如果小于等于 600 则视为事件发生 if (random.Next(0, precious) <= p * precious) { graphics.DrawLine(Pens.Gray, points[i], points[j]); } } } //释放资源 graphics.Dispose(); } /// <summary> /// 计算一个点绕某点旋转之后的坐标值 /// </summary> /// <param name="origin">旋转的圆心</param> /// <param name="point">需要旋转的点</param> /// <param name="dgree">旋转的角度(逆时针)</param> /// <returns>返回旋转后的坐标</returns> public static Point Rotate(Point origin, Point point, double dgree) { Point rotated = new Point(); double dgreePi = dgree / 180 * Math.PI; rotated.X = (int)((point.X - origin.X) * Math.Cos(dgreePi) - (point.Y - origin.Y) * Math.Sin(dgreePi) + origin.X); rotated.Y = (int)((point.X - origin.X) * Math.Sin(dgreePi) + (point.Y - origin.Y) * Math.Cos(dgreePi) + origin.Y); return rotated; }
1.1.32
解答
绘图结果:
完整的项目代码可以去 Github 上下载。
代码(绘图部分)
public static void StartDrawing(double[] array, int N, double l, double r) { //创建并显示绘图窗口 Form2 DrawPad = new Form2(); DrawPad.Show(); //新建画布 Graphics graphics = DrawPad.CreateGraphics(); //翻转默认坐标系 graphics.TranslateTransform(0, DrawPad.Height); graphics.ScaleTransform(1, -1); //对原始数组排序 Array.Sort(array); //计算各区域的值 int[] counts = new int[N]; int index = 0; for (int i = 0; i < N; ++i) { for (int j = index; j < array.Length; ++j) { if (array[j] <= (r - l) * (i + 1) / N) { counts[i]++; index++; } else { break; } } } //获取最大值 double max = counts.Max(); //计算间距 double unit = DrawPad.Width / (3.0 * N + 1); //计算直方图的矩形 Rectangle[] rects = new Rectangle[N]; rects[0].X = (int)unit; rects[0].Y = 0; rects[0].Width = (int)(2 * unit); rects[0].Height = (int)((counts[0] / max) * DrawPad.Height); for (int i = 1; i < N; ++i) { rects[i].X = (int)(rects[i - 1].X + 3 * unit); rects[i].Y = 0; rects[i].Width = (int)(2 * unit); rects[i].Height = (int)((counts[i] / (max + 1)) * DrawPad.Height); } //绘图 graphics.FillRectangles(Brushes.Black, rects); //释放资源 graphics.Dispose(); }
1.1.33
解答
这里矩阵使用交错数组实现(方便取行向量),不是普通的二维数组。
矩阵和矩阵、矩阵和向量、向量和矩阵都使用行向量点乘列向量的方式计算。
代码
public class Matrix { /// <summary> /// 计算两个向量的点积 /// </summary> /// <param name="x">需要点乘的向量</param> /// <param name="y">需要点乘的另一个向量</param> /// <returns>返回点乘的结果</returns> /// <exception cref="FormatException"></exception> public static double Dot(double[] x, double[] y) { //确保两向量等长 if (x.Length != y.Length) { throw new FormatException("the length of two vectors must be equal"); } //点乘 double result = 0; for (int i = 0; i < x.Length; ++i) { result += x[i] * y[i]; } return result; } /// <summary> /// 计算两个矩阵相乘的结果,返回一个矩阵 /// </summary> /// <param name="a">用交错数组表示的 m * p 矩阵</param> /// <param name="b">用交错数组表示的 p * n 矩阵</param> /// <returns>返回 m * n 的矩阵</returns> /// <exception cref="FormatException"></exception> /// <example> /// a = {(1,2,3),(4,5,6)} /// b = {(1,4),(2,5),(3,6)} /// Mult(a, b) = {(14,32),(32,77)} /// </example> public static double[][] Mult(double[][] a, double[][] b) { if (a[0].Length != b.GetLength(0)) { throw new FormatException("a's column number must be equal to b's row number"); } int m = a.GetLength(0); int n = b[0].Length; int p = a[0].Length; double[][] result = new double[m][]; for (int i = 0; i < m; ++i) { double[] resultrow = new double[n]; for (int j = 0; j < n; ++j) { //result[i][j] = 行向量 a[i] 与列向量 b[j] 的点积 double[] row = a[i]; double[] col = new double[p]; //取得列向量 for (int k = 0; k < p; ++k) { col[k] = b[k][j]; } //点积 resultrow[j] = Dot(row, col); } result[i] = resultrow; } return result; } /// <summary> /// 将一个矩阵转置 /// </summary> /// <param name="a">待转置的矩阵</param> /// <returns>返回转置后的数组</returns> public static double[][] Transpose(double[][] a) { double[][] trans = new double[a[0].Length][]; for (int i = 0; i < a[0].Length; ++i) { double[] row = new double[a.GetLength(0)]; for (int j = 0; j < a.GetLength(0); ++j) { row[j] = a[j][i]; } trans[i] = row; } return trans; } /// <summary> /// 计算矩阵与向量的乘积 /// </summary> /// <param name="a">左乘的矩阵</param> /// <param name="x">列向量</param> /// <returns>返回一个向量</returns> /// <exception cref="FormatException"></exception> public static double[] Mult(double[][] a, double[] x) { if (a[0].Length != x.Length) { throw new FormatException("a's column number must be equal to x's length"); } double[] result = new double[a.GetLength(0)]; for (int i = 0; i < a.GetLength(0); ++i) { result[i] = Dot(a[i], x); } return result; } /// <summary> /// 计算向量与矩阵的乘积 /// </summary> /// <param name="x">行向量</param> /// <param name="a">矩阵</param> /// <returns>返回一个向量</returns> /// <exception cref="FormatException"></exception> public static double[] Mult(double[] x, double[][] a) { if (a.GetLength(0) != x.Length) { throw new FormatException("a's column number must be equal to x's length"); } double[] result = new double[a[0].Length]; for (int i = 0; i < a[0].Length; ++i) { double[] colVector = new double[a.GetLength(0)]; for (int j = 0; j < colVector.Length; ++j) { colVector[j] = a[j][i]; } result[i] = Dot(x, colVector); } return result; } /// <summary> /// 在控制台上输出矩阵 /// </summary> /// <param name="a">需要输出的矩阵</param> public static void PrintMatrix(double[][] a) { for (int i = 0; i < a.GetLength(0); ++i) { for (int j = 0; j < a[i].Length; ++j) { Console.Write($"\t{a[i][j]}"); } Console.Write("\n"); } } /// <summary> /// 在控制台上输出一行向量 /// </summary> /// <param name="a">需要输出的向量</param> public static void PrintVector(double[] a) { for (int i = 0; i < a.Length; ++i) { Console.Write($"\t{a[i]}"); } Console.Write("\n"); } }
1.1.34
解答
第二个以及最后三个需要,其他都可以设计成过滤器的模式。
这里的 largeW.txt 只需要保留前 100 个数字就可以了,太多的话最后两个测试会刷屏。
代码
static void Main(string[] args) { string[] AllNumbers = File.ReadAllLines("largeW.txt"); int N = AllNumbers.Length; int[] input = new int[N]; for (int i = 0; i < N; ++i) { input[i] = int.Parse(AllNumbers[i]); } MinAndMax(input); Console.WriteLine(); MidNumber(input); Console.WriteLine(); NumberK(4, input); Console.WriteLine(); SquareSum(input); Console.WriteLine(); AboveAverage(input); Console.WriteLine(); Ascending(input); Console.WriteLine(); Shuffle(input); Console.WriteLine(); } /// <summary> /// 获取最大值和最小值 /// </summary> /// <param name="input">输入流</param> static void MinAndMax(int[] input) { //只用到了两个变量 int min = input[0]; int max = input[0]; //只对输入值正向遍历一遍,不需要保存 for (int i = 1; i < input.Length; ++i) { if (input[i] > max) { max = input[i]; } if (input[i] < min) { min = input[i]; } } Console.WriteLine("Min and Max:"); Console.WriteLine($"Min: {min}\nMax: {max}"); } /// <summary> /// 获取中位数 /// </summary> /// <param name="input">输入流</param> /// <returns>中位数</returns> static int MidNumber(int[] input) { //需要对输入值进行去重 & 排序,故需要保存 List<int> DistinctNumbers = new List<int>(input.Distinct()); DistinctNumbers.Sort(); Console.WriteLine("MidNumber:"); Console.WriteLine(DistinctNumbers[DistinctNumbers.Count / 2]); return DistinctNumbers[DistinctNumbers.Count / 2]; } /// <summary> /// 获取第 k 小的数 /// </summary> /// <param name="k">需要获取的排名</param> /// <param name="input">输入流</param> /// <returns>第 k 小的数</returns> static int NumberK (int k, int[] input) { int[] temp = new int[101]; //只正向遍历一遍,不需要保存 for (int i = 0; i < input.Length; ++i) { if (i < 100) { temp[i] = input[i]; } else { temp[100] = input[i]; Array.Sort(temp); } } Console.WriteLine("NumberK"); Console.WriteLine($"No.k: {temp[k - 1]}"); return temp[k - 1]; } /// <summary> /// 计算输入流中所有数的平方和 /// </summary> /// <param name="input">输入流</param> /// <returns>所有数的平方和</returns> static long SquareSum(int[] input) { long sum = 0; //只正向遍历一遍,不需要保存 for (int i = 0; i < input.Length; ++i) { sum += input[i] * input[i]; } Console.WriteLine("Sum Of Square:"); Console.WriteLine(sum); return sum; } /// <summary> /// 计算所有输入数据的平均值 /// </summary> /// <param name="input">输入流</param> /// <returns>所有输入数据的平均值</returns> static double Average(int[] input) { long sum = 0; //只遍历一遍,且不保存整个数组 for (int i = 0; i < input.Length; ++i) { sum += input[i]; } double ave = sum / (double)input.Length; Console.WriteLine("Average:"); Console.WriteLine(ave); return ave; } /// <summary> /// 计算大于平均值的元素数量 /// </summary> /// <param name="input">输入流</param> /// <returns>大于平均值的元素数量</returns> static double AboveAverage(int[] input) { double ave = Average(input); Console.WriteLine(); double count = 0; for (int i = 0; i < input.Length; ++i) { if (input[i] > ave) { count++; } } Console.WriteLine("AboveAverage:"); Console.WriteLine($"{(count / input.Length) * 100}%"); return count; } /// <summary> /// 升序打印数组 /// </summary> /// <param name="input">输入流</param> static void Ascending(int[] input) { Array.Sort(input); Console.WriteLine("Ascending:"); for (int i = 0; i < input.Length; ++i) { Console.Write($" {input[i]}"); } Console.Write("\n"); } /// <summary> /// 随机打印数组 /// </summary> /// <param name="input">输入流</param> static void Shuffle(int[] input) { Random random = new Random(); List<int> All = new List<int>(input); int N = input.Length; int temp = 0; Console.WriteLine("Shuffle:"); for (int i = 0; i < N; ++i) { temp = random.Next(0, All.Count - 1); Console.Write($" {All[temp]}"); All.RemoveAt(temp); } }
实验题(1.1.35~1.1.39)
1.1.35
解答
这里用 Random 类模拟掷骰子并计算概率,最后和程序得出的比较。
代码
//程序运行大概需要十几秒时间(也可能更长,看运气) //我的数据: //24098 44448 37776 44401 32541 static void Main(string[] args) { //书中给出的程序 int SIDES = 6; double[] dist = new double[2 * SIDES + 1]; for (int i = 1; i <= SIDES; i++) for (int j = 1; j <= SIDES; j++) dist[i + j] += 1.0; for (int k = 2; k <= 2 * SIDES; k++) dist[k] /= 36.0; //不断进行模拟,直至误差小于 0.001 int N = 36; bool isAccepted = false; double[] disttemp = null; double error = 0.001; while (isAccepted == false) { disttemp = PlayDice(N); isAccepted = true; for (int i = 0; i < disttemp.Length; ++i) { if (Math.Abs(disttemp[i] - dist[i]) >= error) isAccepted = false; } N++; } Console.WriteLine($"N:{N}\n"); for (int i = 0; i < dist.Length; ++i) { Console.WriteLine($"{i}:\n Standerd:{dist[i]}\nSimulated:{disttemp[i]}\nOffset:{Math.Abs(disttemp[i] - dist[i])}"); } } //利用随机数模拟掷骰子 static double[] PlayDice(int N) { Random random = new Random(); int SIDES = 6; double[] dist = new double[2 * SIDES + 1]; //掷 N 次 int sumtemp = 0; for (int i = 0; i < N; ++i) { sumtemp = random.Next(1, 7) + random.Next(1, 7); dist[sumtemp]++; } //计算概率 for (int i = 0; i < dist.Length; ++i) { dist[i] /= N; } return dist; }
1.1.36
解答
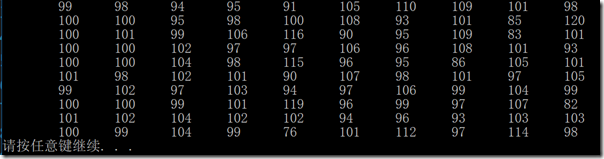
N 取到 1000 左右数据就比较明显了。
N = 1000, M = 10
代码
static void Main(string[] args) { int M = 10;//数组大小 int N = 1000;//打乱次数 int[] a = new int[10]; int[,] result = new int[M, M]; for (int i = 0; i < N; ++i) { //初始化 for (int j = 0; j < a.Length; ++j) { a[j] = j; } //打乱 Shuffle(a, i); //记录 for (int j = 0; j < M; ++j) { result[a[j], j]++; } } PrintMatrix(result); } /// <summary> /// 打乱数组 /// </summary> /// <param name="a">需要打乱的数组</param> /// <param name="seed">用于生成随机数的种子值</param> static void Shuffle(int[] a, int seed) { int N = a.Length; Random random = new Random(seed); for (int i = 0; i < N; ++i) { int r = i + random.Next(N - i);//等于StdRandom.uniform(N-i) int temp = a[i]; a[i] = a[r]; a[r] = temp; } } /// <summary> /// 在控制台上输出矩阵 /// </summary> /// <param name="a">需要输出的矩阵</param> public static void PrintMatrix(int[,] a) { for (int i = 0; i < a.GetLength(0); ++i) { for (int j = 0; j < a.GetLength(1); ++j) { Console.Write($"\t{a[i,j]}"); } Console.Write("\n"); } }
1.1.37
解答
使用 0~N-1 的随机数会导致每次交换的数字可能相同。
例如:
原数组: 1 2 3 4。
第一次: 2 1 3 4 random = 1,第 0 个和第 1 个交换。
第二次: 1 2 3 4 random = 0,第 1 个和第 0 个交换。
代码
static void Main(string[] args) { int M = 10;//数组大小 int N = 100000;//打乱次数 int[] a = new int[10]; int[,] result = new int[M, M]; for (int i = 0; i < N; ++i) { //初始化 for (int j = 0; j < a.Length; ++j) { a[j] = j; } //打乱 Shuffle(a, i); //记录 for (int j = 0; j < M; ++j) { result[a[j], j]++; } } PrintMatrix(result); } /// <summary> /// 打乱数组(不够好的版本) /// </summary> /// <param name="a">需要打乱的数组</param> /// <param name="seed">用于生成随机数的种子值</param> static void Shuffle(int[] a, int seed) { int N = a.Length; Random random = new Random(seed); for (int i = 0; i < N; ++i) { //int r = i + random.Next(N - i); int r = random.Next(N); //返回的是 0 ~ N-1 之间的随机整数 int temp = a[i]; a[i] = a[r]; a[r] = temp; } } /// <summary> /// 在控制台上输出矩阵 /// </summary> /// <param name="a">需要输出的矩阵</param> public static void PrintMatrix(int[,] a) { for (int i = 0; i < a.GetLength(0); ++i) { for (int j = 0; j < a.GetLength(1); ++j) { Console.Write($"\t{a[i, j]}"); } Console.Write("\n"); } }
1.1.38
解答
为了使差距比较明显,故意取了比较靠后的数字。
代码
static void Main(string[] args) { string[] largeWString = File.ReadAllLines("largeW.txt"); int[] largeW = new int[largeWString.Length]; for (int i = 0; i < largeW.Length; ++i) { largeW[i] = int.Parse(largeWString[i]); } Stopwatch timer = Stopwatch.StartNew(); BruteForceSearch(111111, largeW); Console.WriteLine($"BruteForceSearch: {timer.ElapsedMilliseconds} ms"); timer.Restart(); rank(111111, largeW); Console.WriteLine($"BinarySearch: {timer.ElapsedMilliseconds} ms"); string[] largeTString = File.ReadAllLines("largeT.txt"); int[] largeT = new int[largeTString.Length]; for (int i = 0; i < largeW.Length; ++i) { largeT[i] = int.Parse(largeTString[i]); } timer.Restart(); BruteForceSearch(111111, largeT); Console.WriteLine($"BruteForceSearch: {timer.ElapsedMilliseconds} ms"); timer.Restart(); rank(111111, largeT); Console.WriteLine($"BinarySearch: {timer.ElapsedMilliseconds} ms"); } //暴力查找 public static int BruteForceSearch(int key, int[] a) { for (int i = 0; i < a.Length; ++i) { if (a[i] == key) return i; } return -1; } //重载方法,用于启动二分查找 public static int rank(int key, int[] a) { return rank(key, a, 0, a.Length - 1, 1); } //二分查找 public static int rank(int key, int[] a, int lo, int hi, int number) { if (lo > hi) { return -1; } int mid = lo + (hi - lo) / 2; if (key < a[mid]) { return rank(key, a, lo, mid - 1, number + 1); } else if (key > a[mid]) { return rank(key, a, mid + 1, hi, number + 1); } else { return mid; } }
1.1.39
解答
按照要求编程就好,视机器不同需要的时间也不同。
代码
//需要 6 秒左右的运算时间 static void Main(string[] args) { Random r = new Random(); int baseNum = 10; int powNum = 3; int T = 10; int M = 4; double[,] Matrix = new double[M,2]; for (int i = 0; i < M; ++i) { int N = (int)Math.Pow(baseNum, powNum + i); double sum = 0; for (int j = 0; j < T; ++j) { sum += Test(N, r.Next()); } Matrix[i, 0] = N; Matrix[i, 1] = sum / T; } PrintMatrix(Matrix); } /// <summary> /// 执行一次“实验” /// </summary> /// <param name="N">数组的大小</param> /// <param name="seed">随机种子</param> /// <returns>返回相同数字的数目</returns> static int Test(int N, int seed) { Random random = new Random(seed); int[] a = new int[N]; int[] b = new int[N]; int count = 0; for (int i = 0; i < N; ++i) { a[i] = random.Next(100000, 1000000); b[i] = random.Next(100000, 1000000); } for (int i = 0; i < N; ++i) { if (rank(a[i], b) != -1) count++; } return count; } //重载方法,用于启动二分查找 public static int rank(int key, int[] a) { return rank(key, a, 0, a.Length - 1, 1); } //二分查找 public static int rank(int key, int[] a, int lo, int hi, int number) { if (lo > hi) { return -1; } int mid = lo + (hi - lo) / 2; if (key < a[mid]) { return rank(key, a, lo, mid - 1, number + 1); } else if (key > a[mid]) { return rank(key, a, mid + 1, hi, number + 1); } else { return mid; } } /// <summary> /// 在控制台上输出矩阵 /// </summary> /// <param name="a">需要输出的矩阵</param> public static void PrintMatrix(double[,] a) { for (int i = 0; i < a.GetLength(0); ++i) { for (int j = 0; j < a.GetLength(1); ++j) { Console.Write($"\t{a[i, j]}"); } Console.Write("\n"); } }