2D空间中求一点是否在多边形内

参考自这篇博文：http://www.cnblogs.com/dabiaoge/p/4491540.html

 

一开始没仔细看做法，浪费了不少时间。下面是最终实现的效果：

 

大致流程：

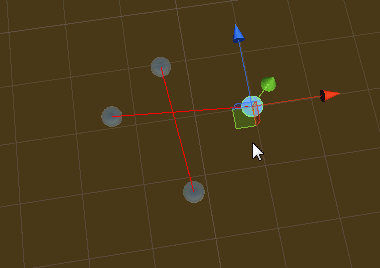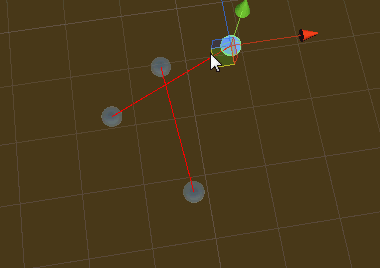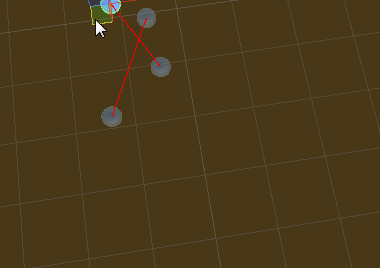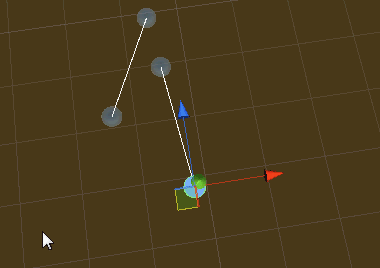
1.随便选取多边形上任意一条边，以比较点和边的中心点做一条射线(这里用的伪射线)

2.用这条射线与其他所有边判断是否相交

3.将所有与线段相交的数量相加，如果是奇数就在多边形内

 

特殊情况：

1.刚好在点上或者在线上。实际运用时会有点误差，但不影响

2.必须是闭合线段，且不能存在包含的情况。但如果是一个数组生成的线段，不会有这种情况

 

代码：

using UnityEngine;

public class Test1 : MonoBehaviour
{
    private const float kRaycastLen = 100000f;
    public Transform[] points;
    public Transform compare;


    private bool IsContact(Transform compare)
    {
        Vector3 comparePoint = (points[1].position + points[0].position) * 0.5f;
        Vector3 originPoint = compare.transform.position;
        comparePoint += (comparePoint - originPoint).normalized * kRaycastLen;

        Debug.DrawLine(originPoint, comparePoint);

        int count = 0;
        for (int i = 0; i < points.Length; i++)
        {
            Transform a = points[i % points.Length];
            Transform b = points[(i + 1) % points.Length];

            bool r = IsIntersection(a.position, b.position, originPoint, comparePoint);

            if (r) count++;
        }

        return count % 2 == 1;
    }

    void OnDrawGizmos()
    {
        if (!compare) return;

        if (IsContact(compare))
            Gizmos.color = Color.red;

        for (int i = 0; i < points.Length; i++)
        {
            Transform a = points[i % points.Length];
            Transform b = points[(i + 1) % points.Length];

            Gizmos.DrawLine(a.position, b.position);
        }
    }

    private bool IsIntersection(Vector3 a, Vector3 b, Vector3 c, Vector3 d)
    {
        float crossA = Vector3.Cross(d - c, a - c).y;
        float crossB = Vector3.Cross(d - c, b - c).y;

        if (!(crossA > 0f ^ crossB > 0f)) return false;

        float crossC = Vector3.Cross(b - a, c - a).y;
        float crossD = Vector3.Cross(b - a, d - a).y;

        if (!(crossC > 0f ^ crossD > 0f)) return false;

        return true;
    }
}

 

 

另外参考的文章中没有说差乘判断两个线段是否相交的具体做法，这里说明一下

 

大致流程：

现在有线段AB和线段CD

用线段AB的方向和C，D两点构成的向量分别做差乘比较。如果C,D在同侧则return跳出

用线段CD的方向和A，B两点构成的向量分别做差乘比较。如果A,B在同侧则return跳出

最终返回相交

 

脚本就是上面的IsIntersection函数，最终实现效果（只适用于2D空间，如果是XY轴向要取差乘的z分量做比较）：