BZOJ 1221 [HNOI2001] 软件开发 费用流_建模

题目描述：

 

某软件公司正在规划一项n天的软件开发计划，根据开发计划第i天需要ni个软件开发人员，为了提高软件开发人员的效率，公司给软件人员提供了很多的服务，其中一项服务就是要为每个开发人员每天提供一块消毒毛巾，这种消毒毛巾使用一天后必须再做消毒处理后才能使用。消毒方式有两种，A种方式的消毒需要a天时间，B种方式的消毒需要b天（b>a），A种消毒方式的费用为每块毛巾fA, B种消毒方式的费用为每块毛巾fB，而买一块新毛巾的费用为f（新毛巾是已消毒的，当天可以使用）；而且f>fA>fB。公司经理正在规划在这n天中，每天买多少块新毛巾、每天送多少块毛巾进行A种消毒和每天送多少块毛巾进行B种消毒。当然，公司经理希望费用最低。你的任务就是：为该软件公司计划每天买多少块毛巾、每天多少块毛巾进行A种消毒和多少毛巾进行B种消毒，使公司在这项n天的软件开发中，提供毛巾服务的总费用最低。

 

 

题解：

十分巧妙的建模方式.

首先，设超级源点 $S$ 和超级汇点 $T$.

1、从 $S$ 向每个 $X_{i}$ 连一条容量为$r_{i}$，费用为 $0$ 的有向边.

2、从每个 $Y_{i}$ 向 $T$ 连一条容量为 $r_{i}$, 费用为 $0$ 的有向边.

3、从 $S$ 向每个 $Y_{i}$ 连一条容量为无穷大，费用为 $f$ 的有向边.

4、从每个 $X_{i}$ 向 $X_{i+1}$ $(i+1<=N)$ 连一条容量为无穷大，费用为 $0$ 的有向边.

5、从每个 $X_{i}$ 向 $Y_{i+a+1}$ $(i+a+1<=N)$连一条容量为无穷大，费用为 $f_{a}$的有向边.

6、从每个 $X_{i}$ 向 $Y_{i+b+1}$ $(i+b+1<=N)$ 连一条容量为无穷大，费用为 $f_{b}$ 的有向边.

 

上一个图片： 

 

 

 

 

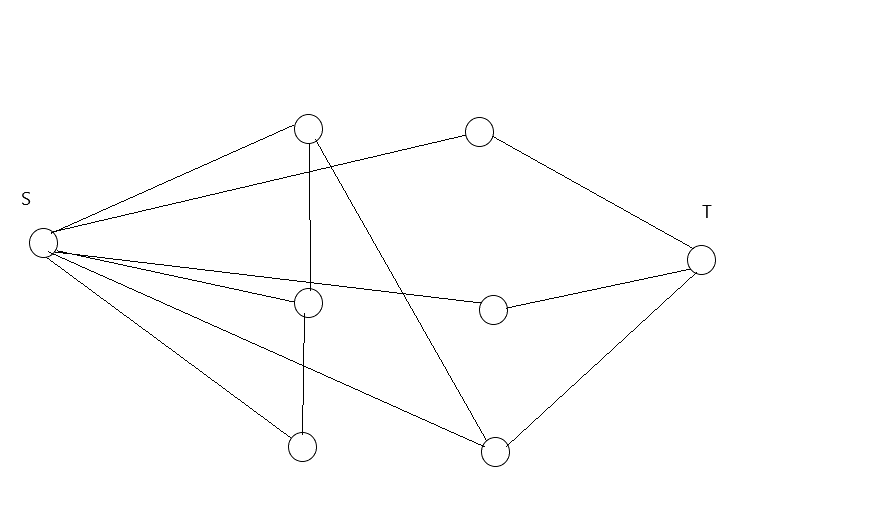
观察上述建模方式与“形象化”图片，我们发现我们构建的网络模型满足流出量与流入量守恒.

每个 $y_{i}$ 会由先前剩下的 $f_{a}$ 与 $f_{b}$ 填充，再由源点直接流入 $y_{i}$ 的 $f$ 所填入. 

一个十分巧妙的网络流模型. 

 

Code:

#include <bits/stdc++.h>
#define setIO(s) freopen(s".in","r",stdin) 
#define maxn 300000  
#define inf 1000000 
#define N 3005 
#define nex(n) (n + 1002) 
using namespace std;
struct Edge
{
    int from,to,cap,cost;
    Edge(int from = 0,int to = 0,int cap = 0,int cost = 0) : from(from),to(to),cap(cap),cost(cost){} 
}; 
vector<Edge>edges;
vector<int>G[maxn]; 
void addedge(int u,int v,int c,int d)
{
    edges.push_back(Edge(u,v,c,d)); 
    edges.push_back(Edge(v,u,0,-d)); 
    int m = edges.size(); 
    G[u].push_back(m - 2); 
    G[v].push_back(m - 1); 
}
int flow2[maxn],d[maxn],inq[N],pre[N],nn[N]; 
int ans,s,t; 
queue <int> Q; 
int spfa()
{
    for(int i = 0;i < N; ++i) d[i] = flow2[i] = inf; 
    memset(inq,0,sizeof(inq)); 
    d[s] = 0, inq[s] = 1;
    Q.push(s);
    while(!Q.empty())
    {
        int u = Q.front(); Q.pop(); 
        inq[u] = 0; 
        for(int sz = G[u].size(),i = 0; i < sz ; ++i)
        {
            Edge e = edges[G[u][i]]; 
            if(d[e.to] > d[u] + e.cost && e.cap > 0)
            {
                d[e.to] = d[u] + e.cost;
                flow2[e.to] = min(e.cap, flow2[u]); 
                pre[e.to] = G[u][i]; 
                if(!inq[e.to]) 
                {
                    Q.push(e.to); 
                    inq[e.to] = 1; 
                }
            } 
        }
    }
    if(flow2[t] == inf) return 0; 
    int f = flow2[t]; 
    edges[pre[t]].cap -= f, edges[pre[t] ^ 1].cap += f; 
    int u = edges[pre[t]].from; 
    while(u != s)
    {
        edges[pre[u]].cap -= f, edges[pre[u] ^ 1].cap += f; 
        u = edges[pre[u]].from; 
    }
    ans += f * d[t]; 
    return 1; 
}
int getcost()
{
    while(spfa());
    return ans; 
}
int main()
{
    // setIO("input"); 
    int n,a,b,f,fa,fb; 
    scanf("%d%d%d%d%d%d",&n,&a,&b,&f,&fa,&fb); 
    for(int i = 1;i <= n; ++i) scanf("%d",&nn[i]);   
    s = 0,t = 2500; 
    for(int i = 1;i <= n; ++i) 
    {
        addedge(s, i, nn[i], 0);
        addedge(nex(i), t, nn[i], 0); 
        addedge(s, nex(i), inf, f); 
        if(i + 1 <= n) addedge(i, i + 1, inf,0); 
    }
    for(int i = 1;i <= n; ++i) 
    {
        if(i + a + 1 <= n) addedge(i,nex(i + 1 + a),inf,fa); 
        if(i + b + 1 <= n) addedge(i,nex(i + b + 1),inf,fb); 
    }    
    printf("%d",getcost()); 
    return 0; 
}