深度优先搜索、递归和回溯，遍历法和分治法的定义和区别

递归 Recursion

递归函数：调用自己的函数。

递归算法：大问题依赖于小问题，先递归函数求解小问题

一般算法中的递归指的是递归函数。

 

深度优先搜索 Depth First Search

递归函数实现，在搜索过程中优先搜索更深的点而不是同层的点的算法。

也可以用栈代替递归函数，不过一般是都递归实现DFS。

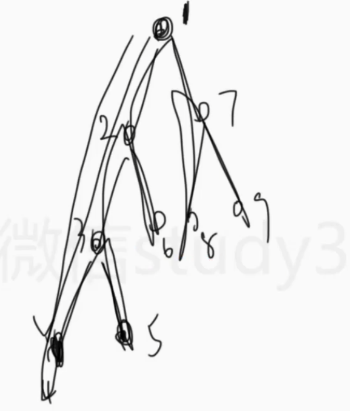
回溯 Backtracking

回溯法：就是深度优先搜索算法

回溯操作：递归函数在回到上一层递归调用时，将参数该回到调用前的值，这个操作就是回溯。

 

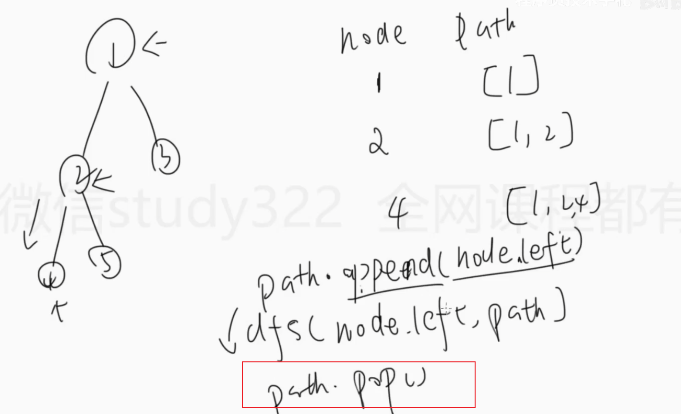
遍历 VS 分治法

遍历：一个小人拿着一个记事本走遍所有节点

分治法：分配小弟去做子任务，自己对子任务的结果进行汇总

 

结果的保存：

遍历：通常用一个全局变量或共享参数

分治法：通常利用返回值记录子问题的结果，当前任务对子任务的结果进行汇总。

 

二叉树分治法模板：

public 返回结果类型 function(TreeNode root)
    {
        if(root==null){
            空树返回结果;
        }
        
        if(root.left==null && root.right==null)
        {
            叶子节点返回的结果;
        }
        
        左子树返回结果=function(root.left);
        右子树返回结果=function(root.left);
        当前节点的结果=按照一定方法合并左右子树的结果;
        return 当前节点的结果;
    }

二叉树的所有路径——分治法、回溯法

题目：二叉树的所有路径

给你一个二叉树的根节点 root ，按 任意顺序 ，返回所有从根节点到叶子节点的路径。

示例 1：

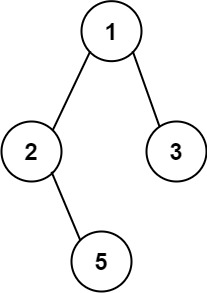
输入：root = [1,2,3,null,5]
输出：["1->2->5","1->3"]

题解

/**
     * 回溯法
*/
    class Solution {
        List<String> res;
        public void dfs(TreeNode root, List<Integer> node)
        {
            if(root==null) return;
            node.add(root.val);
            if(root.left==null && root.right==null)
            {
                StringBuilder sb=new StringBuilder();
                for(int i=0;i<node.size();i++)
                {
                    if(i==0) sb.append(node.get(i));
                    else sb.append("->"+node.get(i));
                }
                res.add(sb.toString());
                node.remove(node.size()-1);
                return;
            }

            dfs(root.left, node);
            dfs(root.right, node);
            node.remove(node.size()-1);
        }
        public List<String> binaryTreePaths(TreeNode root) {
            res=new ArrayList<>();
            List<Integer> node=new ArrayList<>();
            dfs(root, node);

            return res;
        }
    }

 

/**
     * 分治法
     * O(n2)
     */

    class Solution2 {
        public List<String> dfs(TreeNode root)
        {
            List<String> paths=new ArrayList<>();
            if(root==null) return paths;
            if(root.left==null && root.right==null)
            {
                paths.add(""+root.val);
                return paths;
            }

            for(String path: dfs(root.left))
                paths.add(root.val+"->"+path);
            for(String path: dfs(root.right))
                paths.add(root.val+"->"+path);
            return paths;
        }
        pu