[LeetCode] 1260. Shift 2D Grid 二维网格迁移
Given a 2D grid of size m x n and an integer k. You need to shift the grid k times.
In one shift operation:
- Element at
grid[i][j]moves togrid[i][j + 1]. - Element at
grid[i][n - 1]moves togrid[i + 1][0]. - Element at
grid[m - 1][n - 1]moves togrid[0][0].
Return the 2D grid after applying shift operation k times.
Example 1:
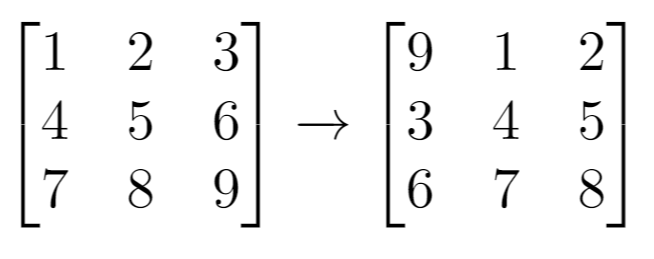
Input: `grid` = [[1,2,3],[4,5,6],[7,8,9]], k = 1
Output: [[9,1,2],[3,4,5],[6,7,8]]
Example 2:
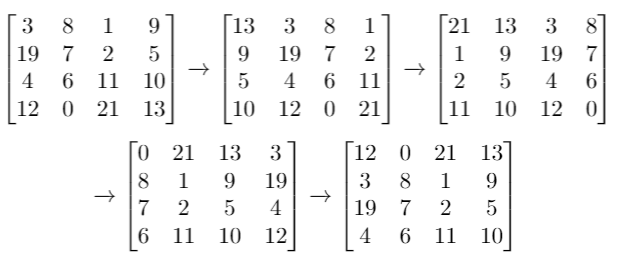
Input: `grid` = [[3,8,1,9],[19,7,2,5],[4,6,11,10],[12,0,21,13]], k = 4
Output: [[12,0,21,13],[3,8,1,9],[19,7,2,5],[4,6,11,10]]
Example 3:
Input: `grid` = [[1,2,3],[4,5,6],[7,8,9]], k = 9
Output: [[1,2,3],[4,5,6],[7,8,9]]
Constraints:
m == grid.lengthn == grid[i].length1 <= m <= 501 <= n <= 50-1000 <= grid[i][j] <= 10000 <= k <= 100
这道题让移动一个二维数组,移动方法是水平移动,即每个元素向右平移一位,行末尾的元素移动到下一行的开头,数组最后一个元素移动到开头的第一个元素,像这样移动k次,返回最终的数组。由于要移动k次,若每次都更新一遍数组的值,实在是不高效,最好直接能计算出最终状态的值,那么关注点就是计算一个元素水平移动k次的新位置。由于是二维数组,所以总是存在一个换行的问题,比较麻烦,一个很好的 trick 就是先将数组拉平,变成一维数组,这样移动k位就很方便,唯一需要注意是加k后可能超过一维数组的范围,需要当作循环数组来处理。明白了思路,代码就很好写了,新建一个和原数组同等大小的数组 res,然后遍历原数组,对于每个位置 (i, j),计算其在拉平后的一维数组中的位置 i*n + j,然后再加上平移k,为了防止越界,最后再对 m*n 取余,得到了其在一维数组中的位置,将其转回二维数组的坐标,并更新结果 res 中的对应位置即可,参见代码如下:
class Solution {
public:
vector<vector<int>> shiftGrid(vector<vector<int>>& grid, int k) {
int m = grid.size(), n = grid[0].size(), len = m * n;
vector<vector<int>> res(m, vector<int>(n));
for (int i = 0; i < m; ++i) {
for (int j = 0; j < n; ++j) {
int idx = (i * n + j + k) % len;
res[idx / n][idx % n] = grid[i][j];
}
}
return res;
}
};
Github 同步地址:
https://github.com/grandyang/leetcode/issues/1260
参考资料:
https://leetcode.com/problems/shift-2d-grid/
https://leetcode.com/problems/shift-2d-grid/discuss/458848/C%2B%2B-Straight-forward-solution






