poj1456——Supermarket

Supermarket

Time Limit: 2000MS		Memory Limit: 65536K
Total Submissions: 14656		Accepted: 6656

Description

A supermarket has a set Prod of products on sale. It earns a profit px for each product x∈Prod sold by a deadline dx that is measured as an integral number of time units starting from the moment the sale begins. Each product takes precisely one unit of time for being sold. A selling schedule is an ordered subset of products Sell ≤ Prod such that the selling of each product x∈Sell, according to the ordering of Sell, completes before the deadline dx or just when dx expires. The profit of the selling schedule is Profit(Sell)=Σ_x∈Sellpx. An optimal selling schedule is a schedule with a maximum profit.
For example, consider the products Prod={a,b,c,d} with (pa,da)=(50,2), (pb,db)=(10,1), (pc,dc)=(20,2), and (pd,dd)=(30,1). The possible selling schedules are listed in table 1. For instance, the schedule Sell={d,a} shows that the selling of product d starts at time 0 and ends at time 1, while the selling of product a starts at time 1 and ends at time 2. Each of these products is sold by its deadline. Sell is the optimal schedule and its profit is 80.

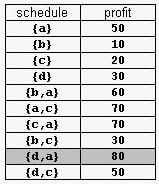
Write a program that reads sets of products from an input text file and computes the profit of an optimal selling schedule for each set of products.

Input

A set of products starts with an integer 0 <= n <= 10000, which is the number of products in the set, and continues with n pairs pi di of integers, 1 <= pi <= 10000 and 1 <= di <= 10000, that designate the profit and the selling deadline of the i-th product. White spaces can occur freely in input. Input data terminate with an end of file and are guaranteed correct.

Output

For each set of products, the program prints on the standard output the profit of an optimal selling schedule for the set. Each result is printed from the beginning of a separate line.

Sample Input

4  50 2  10 1   20 2   30 1

7  20 1   2 1   10 3  100 2   8 2
   5 20  50 10

Sample Output

80
185

Hint

The sample input contains two product sets. The first set encodes the products from table 1. The second set is for 7 products. The profit of an optimal schedule for these products is 185.

 

Solution：

本题题意就是给定n个物品，每个物品有价值和过期时间，在保证卖出的物品不过期的情况下求最大收益。

思路就是贪心，显然对于到了第x天时，我们应该尽量卖出的是在不过期情况下的价值前x大的物品。

于是我们可以先将物品按过期时间从小到大排序，然后依次扫描每个物品：若当前物品的过期天数大于当前小根堆中物品个数，那么就直接插入小根堆中;若当前物品的过期天数等于当前堆中的物品个数，如果该物品价值也大于堆顶的物品价值，则pop出堆顶并插入该物品。

最后小根堆中剩下的物品价值和就是ans了。

 

代码：

#include<iostream>
#include<cstdio>
#include<cstring>
#include<cmath>
#include<algorithm>
#include<queue>
#define il inline
#define ll long long
#define N 100005
using namespace std;
int n;
struct object{
int p,d;
}a[N];
il bool cmp(object a,object b){return a.d<b.d;}
priority_queue<int,vector<int>,greater<int> >q;
int main()
{
    while(scanf("%d",&n)==1)
    {
        int cnt=0,ans=0;
        for(int i=1;i<=n;i++)scanf("%d%d",&a[i].p,&a[i].d);
        sort(a+1,a+n+1,cmp);
        for(int i=1;i<=n;i++)
            if(a[i].d>cnt){cnt++;q.push(a[i].p);}
            else if(a[i].d==cnt){
                if(a[i].p>q.top())q.pop(),q.push(a[i].p);
            }
        while(!q.empty())ans+=q.top(),q.pop();
        printf("%d\n",ans);
    }
    return 0;
}