Friends and Subsequences

Friends and Subsequences

Mike and !Mike are old childhood rivals, they are opposite in everything they do, except programming. Today they have a problem they cannot solve on their own, but together (with you) — who knows?

Every one of them has an integer sequences a and b of length n. Being given a query of the form of pair of integers (l, r), Mike can instantly tell the value of 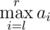 while !Mike can instantly tell the value of .

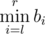Now suppose a robot (you!) asks them all possible different queries of pairs of integers (l, r) (1 ≤ l ≤ r ≤ n) (so he will make exactlyn(n + 1) / 2 queries) and counts how many times their answers coincide, thus for how many pairs 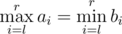 is satisfied.

How many occasions will the robot count?

Input

The first line contains only integer n (1 ≤ n ≤ 200 000).

The second line contains n integer numbers a1, a2, ..., an ( - 109 ≤ ai ≤ 109) — the sequence a.

The third line contains n integer numbers b1, b2, ..., bn ( - 109 ≤ bi ≤ 109) — the sequence b.

Output

Print the only integer number — the number of occasions the robot will count, thus for how many pairs 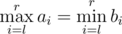 is satisfied.

Examples

input

6
1 2 3 2 1 4
6 7 1 2 3 2

output

2

input

3
3 3 3
1 1 1

output

0

Note

The occasions in the first sample case are:

1.l = 4,r = 4 since max{2} = min{2}.

2.l = 4,r = 5 since max{2, 1} = min{2, 3}.

There are no occasions in the second sample case since Mike will answer 3 to any query pair, but !Mike will always answer 1.

分析:RMQ+二分；

代码：

#include <iostream>
#include <cstdio>
#include <cstdlib>
#include <cmath>
#include <algorithm>
#include <climits>
#include <cstring>
#include <string>
#include <set>
#include <map>
#include <queue>
#include <stack>
#include <vector>
#include <list>
#include <ext/rope>
#define rep(i,m,n) for(i=m;i<=n;i++)
#define rsp(it,s) for(set<int>::iterator it=s.begin();it!=s.end();it++)
#define vi vector<int>
#define pii pair<int,int>
#define mod 1000000007
#define inf 0x3f3f3f3f
#define pb push_back
#define mp make_pair
#define fi first
#define se second
#define ll long long
#define pi acos(-1.0)
const int maxn=2e5+10;
const int dis[][2]={0,1,-1,0,0,-1,1,0};
using namespace std;
using namespace __gnu_cxx;
ll gcd(ll p,ll q){return q==0?p:gcd(q,p%q);}
ll qpow(ll p,ll q){ll f=1;while(q){if(q&1)f=f*p;p=p*p;q>>=1;}return f;}
int n,m,p[maxn],a[20][maxn],b[20][maxn];
ll ans;
void init()
{
    for(int i=2;i<n;i++)p[i]=1+p[i/2];
    for(int i=1;i<20;i++)
        for(int j=0;j+(1<<i)-1<n;j++)
        a[i][j]=max(a[i-1][j],a[i-1][j+(1<<(i-1))]),b[i][j]=min(b[i-1][j],b[i-1][j+(1<<(i-1))]);
    return;
}
int getma(int l,int r)
{
    int x=p[r-l+1];
    return max(a[x][l],a[x][r-(1<<x)+1]);
}
int getmi(int l,int r)
{
    int x=p[r-l+1];
    return min(b[x][l],b[x][r-(1<<x)+1]);
}
int getl(int now)
{
    int l=now-1,r=n;
    while(r-l>1)
    {
        int mid=(l+r)>>1;
        if(getma(now,mid)<getmi(now,mid))l=mid;
        else r=mid;
    }
    return r;
}
int getr(int now)
{
    int l=now-1,r=n;
    while(r-l>1)
    {
        int mid=(l+r)>>1;
        if(getma(now,mid)<=getmi(now,mid))l=mid;
        else r=mid;
    }
    return r;
}
int main()
{
    int i,j,k,t;
    scanf("%d",&n);
    rep(i,0,n-1)scanf("%d",&a[0][i]);
    rep(i,0,n-1)scanf("%d",&b[0][i]);
    init();
    rep(i,0,n-1)ans+=getr(i)-getl(i);
    printf("%lld\n",ans);
    return 0;
}