hdu4565之矩阵快速幂
So Easy!
Time Limit: 2000/1000 MS (Java/Others) Memory Limit: 32768/32768 K (Java/Others)
Total Submission(s): 813 Accepted Submission(s): 226
Problem Description
A sequence S
n is defined as:
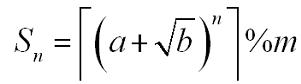
Where a, b, n, m are positive integers.┌x┐is the ceil of x. For example, ┌3.14┐=4. You are to calculate S n.
You, a top coder, say: So easy!

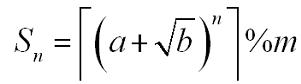
Where a, b, n, m are positive integers.┌x┐is the ceil of x. For example, ┌3.14┐=4. You are to calculate S n.
You, a top coder, say: So easy!

Input
There are several test cases, each test case in one line contains four positive integers: a, b, n, m. Where 0< a, m < 2
15, (a-1)
2< b < a
2, 0 < b, n < 2
31.The input will finish with the end of file.
Output
For each the case, output an integer S
n.
Sample Input
2 3 1 2013
2 3 2 2013
2 2 1 2013
Sample Output
4
14
4
详解在这: http://blog.csdn.net/ljd4305/article/details/8987823
另外Cn=(a+sqrt(b))^n+(a-sqrt(b))^n =>Cn*(a+sqrt(b) + a-sqrt(b))=(a+sqrt(b))^(n+1)+(a-sqrt(b))^(n+1)+(a*a-b)*(a+sqrt(n))^(n-1)+(a*a-b)*(a-sqrt(b))^(n-1)=C(n+1) + (a*a-b)C(n-1) =>C(n+1)=2a*Cn+(b-a^2)*C(n-1)
#include<iostream>
#include<cstdio>
#include<cstdlib>
#include<cstring>
#include<string>
#include<queue>
#include<algorithm>
#include<map>
#include<iomanip>
#define INF 99999999
using namespace std;
const int MAX=10;
__int64 array[2][2],sum[2][2];
void MatrixMult(__int64 a[2][2],__int64 b[2][2],__int64 mod){
__int64 c[2][2]={0};
for(int i=0;i<2;++i){
for(int j=0;j<2;++j){
for(int k=0;k<2;++k){
c[i][j]+=a[i][k]*b[k][j];
}
}
}
for(int i=0;i<2;++i){
for(int j=0;j<2;++j)a[i][j]=c[i][j]%mod;
}
}
__int64 Matrix(__int64 a,__int64 b,__int64 k,__int64 mod){
array[0][0]=a%mod,array[0][1]=(b%mod+mod)%mod;
array[1][0]=1,array[1][1]=0;
sum[0][0]=sum[1][1]=1;
sum[0][1]=sum[1][0]=0;
while(k){
if(k&1)MatrixMult(sum,array,mod);
MatrixMult(array,array,mod);
k>>=1;
}
return (a*sum[0][0]+2*sum[0][1])%mod;
}
int main(){
__int64 a,b,n,m;
while(scanf("%I64d%I64d%I64d%I64d",&a,&b,&n,&m)!=EOF){
if(n == 0)printf("I64d\n",1%m);
else if(n == 1)printf("%I64d\n",2*a%m);
else printf("%I64d\n",Matrix(2*a,b-a*a,n-1,m));
}
return 0;
}





【推荐】国内首个AI IDE,深度理解中文开发场景,立即下载体验Trae
【推荐】编程新体验,更懂你的AI,立即体验豆包MarsCode编程助手
【推荐】抖音旗下AI助手豆包,你的智能百科全书,全免费不限次数
【推荐】轻量又高性能的 SSH 工具 IShell:AI 加持,快人一步
· Linux系列:如何用heaptrack跟踪.NET程序的非托管内存泄露
· 开发者必知的日志记录最佳实践
· SQL Server 2025 AI相关能力初探
· Linux系列:如何用 C#调用 C方法造成内存泄露
· AI与.NET技术实操系列(二):开始使用ML.NET
· 无需6万激活码!GitHub神秘组织3小时极速复刻Manus,手把手教你使用OpenManus搭建本
· C#/.NET/.NET Core优秀项目和框架2025年2月简报
· Manus爆火,是硬核还是营销?
· 终于写完轮子一部分:tcp代理 了,记录一下
· 【杭电多校比赛记录】2025“钉耙编程”中国大学生算法设计春季联赛(1)