数论夏夜实战，然夯实基础尤为重要！

____________________________我往前飞   飞过一片时间海

1.

关于同余式的约去法则：

给出一个正整数m和三个整数a, b和c，d=GCD(c, m)，并且ac≡bc(mod m)。则a≡b(mod (m DIV d))。

 

2.___________________________________大数用于是被其因子这些小数整除的！

我们已经定义了两个数 a 和 b 的最大公约数 (a,b) .关于这个数有一个简单的公式　　　　|  （a，b) = GCD(a,b)!

 

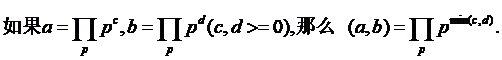
这是 min(c,d) 鸭子！

两个整数 a和b 的最小公倍数(least common multiple  ) 是同时能被 a和 b整除的最小整数.用{a,b}表示。

于是有： a|{a,b}   ,  b|{a,b}

在上述的记号的情况下，有：

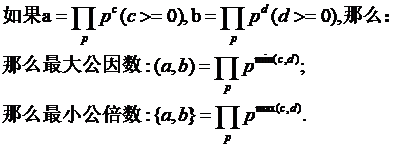图有些糊了，嘻嘻：

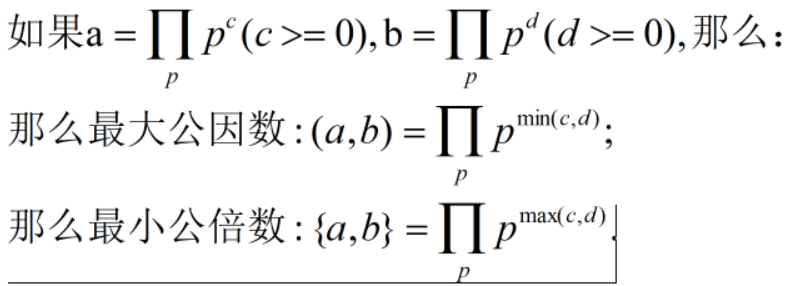
究极总结：

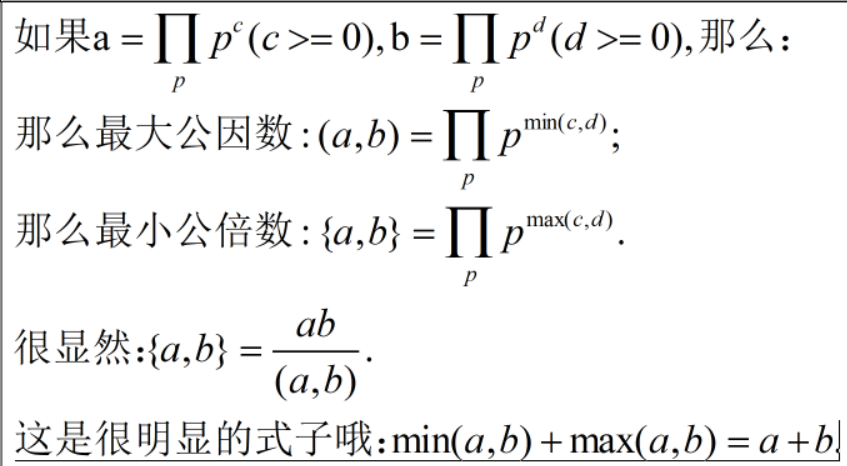
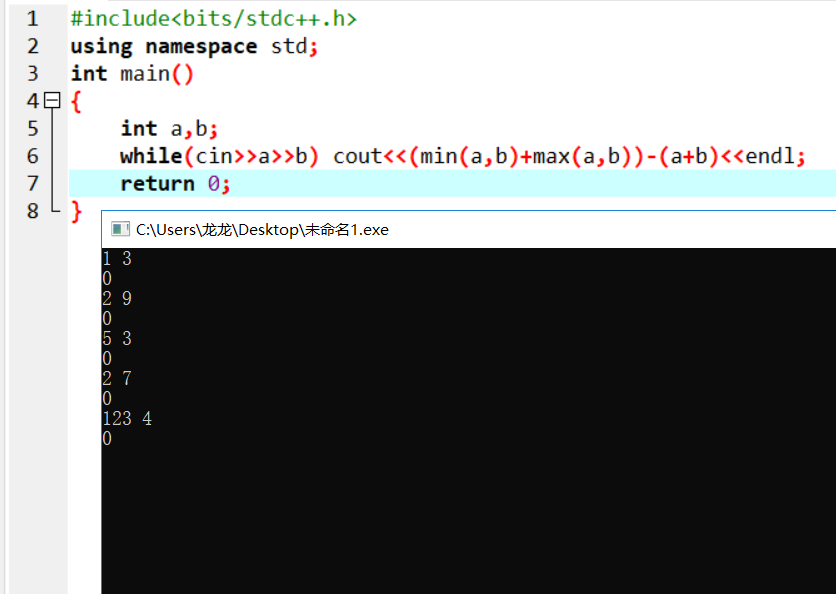
 3.

 

4.有所待：https://vjudge.net/contest/316798#problem/C

 codes:

#include<iostream>
#include<cstring>
#include<algorithm>
using namespace std;
typedef long long ll;
ll pow_mod(ll a,ll p,ll M)
{
	if(p==0) return 1;
	ll ans=pow_mod(a,p/2,M);
	ans=ans*ans%M;
	if(p%2==1) ans=ans*a%M;
	return ans;
}
int main()
{
	int t,h;
	ll M;
	cin>>t;
	while(t--)
	{
		cin>>M>>h;
		ll a,b,ans=0;
		while(h--)
		{
			cin>>a>>b;
			ans=(ans+pow_mod(a,b,M))%M;
		}
		cout<<ans<<endl;
	}
	return 0;
}

　　

 

观览书籍：

《哈代数论》