遗忘因子递推最小二乘法FFRLS

?

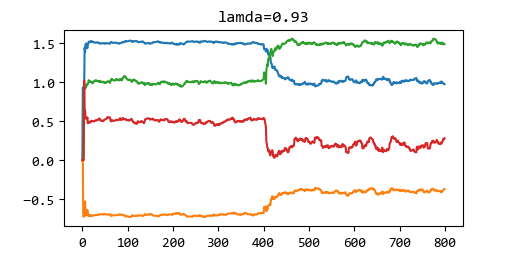
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48

import numpy as np import matplotlib.pyplot as plt from mxulie import M_sequences   if __name__ == '__main__':     L = 800 #序列长度     Y = np.zeros(L)     phi = np.zeros((L,4))     [M,IM]=M_sequences(L)     xi = np.sqrt(0.01) * np.random.randn(L,1)           y1 = y2 =0     u4 = u3 = u2 =u1 =0           P = 1e6 * np.eye(4)     theta1 = np.zeros((L,4))     theta1_1 = np.zeros(4)     lamda = 0.92       for i in np.arange(L):         if i <= 400:             theta = np.array([1.5,-0.7,1,0.5])         else:             theta = np.array([1,-0.4,1.5,0.2])             phi[i,:] = np.array([y1 , y2 ,u3 ,u4])              #Y[i] = 1.5*y1 -0.7*y2 + u3 + 0.5*u4 + xi[i]         Y[i] = np.dot(theta,phi[i,:]) + xi[i]             # FFRLS               K = np.dot(P,phi[i,:])/(lamda+np.dot(np.dot(phi[i,:],P),phi[i,:]))         theta1[i,:] = theta1_1 + K*(Y[i]-np.dot(phi[i,:],theta1_1))         P = (1/lamda)*np.dot(np.eye(4)-phi[i,:]*K.reshape((-1,1)),P)                 # 数据更新         theta1_1 = theta1[i,:]         y2 = y1         y1 = Y[i]           u4 = u3         u3 = u2         u2 = u1         u1 = IM[i]