[LeetCode] 623. Add One Row to Tree

Given the root of a binary tree and two integers val and depth, add a row of nodes with value val at the given depth depth.

Note that the root node is at depth 1.

The adding rule is:

• Given the integer depth, for each not null tree node cur at the depth depth - 1, create two tree nodes with value val as cur's left subtree root and right subtree root.
• cur's original left subtree should be the left subtree of the new left subtree root.
• cur's original right subtree should be the right subtree of the new right subtree root.
• If depth == 1 that means there is no depth depth - 1 at all, then create a tree node with value val as the new root of the whole original tree, and the original tree is the new root's left subtree.

Example 1:

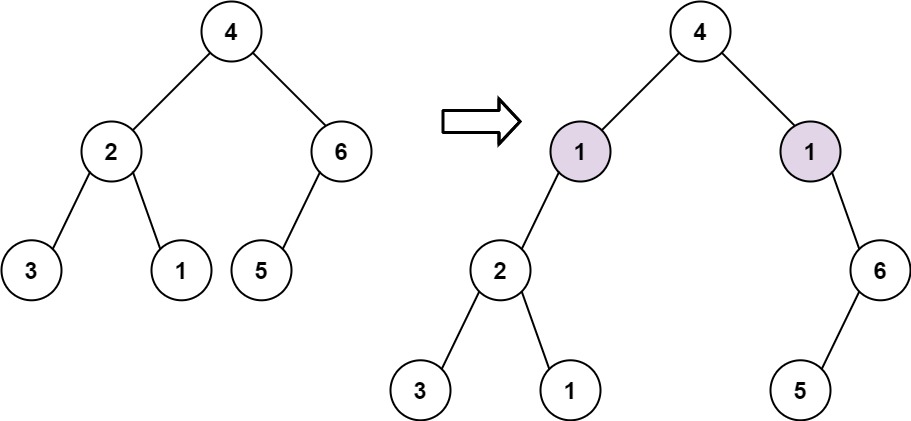
Input: root = [4,2,6,3,1,5], val = 1, depth = 2
Output: [4,1,1,2,null,null,6,3,1,5]

Example 2:

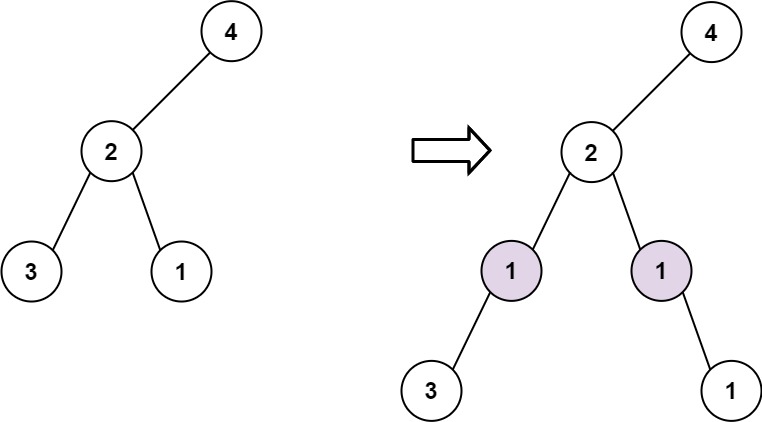
Input: root = [4,2,null,3,1], val = 1, depth = 3
Output: [4,2,null,1,1,3,null,null,1]

Constraints:

• The number of nodes in the tree is in the range [1, 104].
• The depth of the tree is in the range [1, 104].
• -100 <= Node.val <= 100
• -105 <= val <= 105
• 1 <= depth <= the depth of tree + 1

在二叉树中增加一行。

给定一个二叉树的根 root 和两个整数 val 和 depth ，在给定的深度 depth 处添加一个值为 val 的节点行。

注意，根节点 root 位于深度 1 。

加法规则如下:

给定整数 depth，对于深度为 depth - 1 的每个非空树节点 cur ，创建两个值为 val 的树节点作为 cur 的左子树根和右子树根。
cur 原来的左子树应该是新的左子树根的左子树。
cur 原来的右子树应该是新的右子树根的右子树。
如果 depth == 1 意味着 depth - 1 根本没有深度，那么创建一个树节点，值 val 作为整个原始树的新根，而原始树就是新根的左子树。

来源：力扣（LeetCode）
链接：https://leetcode.cn/problems/add-one-row-to-tree
著作权归领扣网络所有。商业转载请联系官方授权，非商业转载请注明出处。

这道题我提供两种做法，BFS和DFS，思路是一样的，都是从根节点往下，一直遍历到 d - 1 层，然后把新的节点加进去。

首先是BFS，我们用queue遍历到目标层 d 的上一层 d - 1，然后对着d - 1 层，先把他的左孩子右孩子用temp指针保存起来，再把需要增加的节点加进去。注意唯一的corner case就是如果新的节点需要加在第一层的话，返回的就是新的根节点了。

时间O(n)

空间O(n)

Java实现

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     int val;
 *     TreeNode left;
 *     TreeNode right;
 *     TreeNode(int x) { val = x; }
 * }
 */
class Solution {
    public TreeNode addOneRow(TreeNode root, int v, int d) {
        // corner case
        if (d == 1) {
            TreeNode newRoot = new TreeNode(v);
            newRoot.left = root;
            return newRoot;
        }

        // normal case
        // BFS遍历node一直到d - 1层
        Queue<TreeNode> queue = new LinkedList<>();
        queue.offer(root);
        for (int i = 1; i < d - 1; i++) {
            int size = queue.size();
            for (int j = 0; j < size; j++) {
                TreeNode cur = queue.poll();
                if (cur.left != null) {
                    queue.offer(cur.left);
                }
                if (cur.right != null) {
                    queue.offer(cur.right);
                }
            }
        }

        // 把新一层的node接在第d - 1和第d层之间
        while (!queue.isEmpty()) {
            TreeNode cur = queue.poll();
            TreeNode temp = cur.left;
            cur.left = new TreeNode(v);
            cur.left.left = temp;
            temp = cur.right;
            cur.right = new TreeNode(v);
            cur.right.right = temp;
        }
        return root;
    }
}

 

DFS，可以直接参见代码。

时间O(n)

空间O(n)

Java实现

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     int val;
 *     TreeNode left;
 *     TreeNode right;
 *     TreeNode(int x) { val = x; }
 * }
 */
class Solution {
    public TreeNode addOneRow(TreeNode root, int v, int d) {
        // corner case
        if (d == 1) {
            TreeNode newRoot = new TreeNode(v);
            newRoot.left = root;
            return newRoot;
        }
        // normal case
        helper(v, root, 1, d);
        return root;
    }

    private void helper(int val, TreeNode root, int curDepth, int d) {
        if (root == null) {
            return;
        }
        if (curDepth == d - 1) {
            TreeNode temp = root.left;
            root.left = new TreeNode(val);
            root.left.left = temp;
            temp = root.right;
            root.right = new TreeNode(val);
            root.right.right = temp;
        } else {
            helper(val, root.left, curDepth + 1, d);
            helper(val, root.right, curDepth + 1, d);
        }
    }
}

 

LeetCode 题目总结